Could Dark Matter just be Normal Stuff that’s Dark?
There’s plenty of gas, dust, planets and rocky bodies out there. Could some combination of them explain dark matter?
Image credit: Chris Blake and Sam Moorfield, via http://www.sdss3.org/surveys/boss.php.
“When a train goes through a tunnel and it gets dark, you don’t throw away the ticket and jump off. You sit still and trust the engineer.” –Corrie Ten Boom
There are plenty of good reasons to believe that dark matter exists: in all sorts of independent situations and from completely unrelated lines of observation, we see the gravitational influence from unseen sources of mass. But there are plenty of known sources of mass out there that don’t emit light at all!
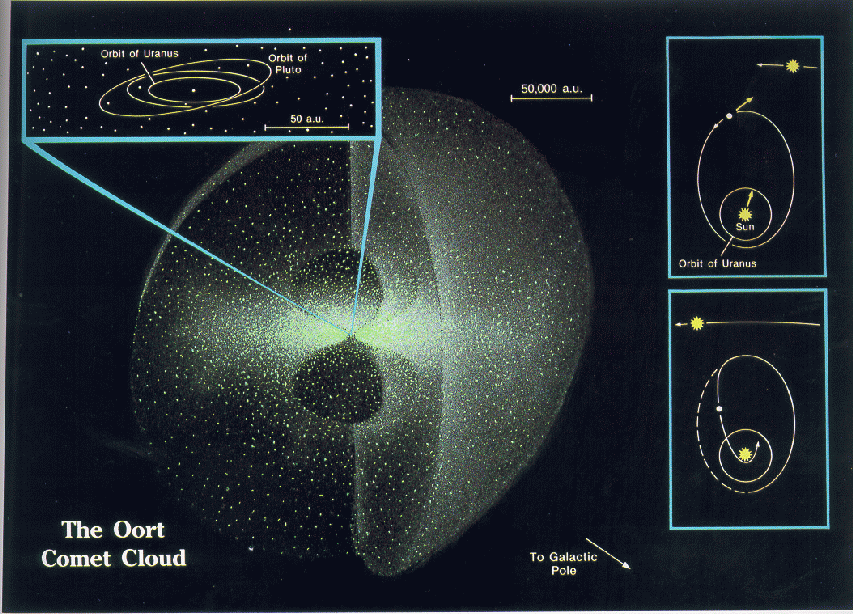
Just because 99.8% of the mass of our Solar System is locked up in our Sun doesn’t mean that stars are a good proxy for the mass in the Universe. Quite to the contrary, we know it’s a lousy one! Moving even slightly beyond the Solar System teaches us that: if we’re willing to include the Oort cloud, a huge cloud of icy bodies extending for approximately a couple of light years in all directions, there are literally trillions of asteroid-and-comet sized objects totaling many times the Earth’s mass, and in the early stages of the Solar System that number was likely even higher.
On scales even larger than that, we can begin to see that there’s also a huge amount of interstellar gas-and-dust in between the stars. That’s obvious from looking at our own galaxy, where the massive “dark spots” in the galactic plane are due to intervening dust blocking the light from stars behind it.

And on even larger scales, we know that intergalactic gas and plasma is ubiquitous, dwarfing all the matter contained in the light-emitting parts of the Universe, the stars, by quite a bit. Is it possible that these forms of matter — gas, dust, ices, rocks and planets — account for all of the missing mass in the Universe?

You might think that something like the above image, of the Bullet Cluster, is a distinct counterexample. After all, if we take two galaxy clusters and collide them, and we see the effects of gravitation (from gravitational lensing, in blue) and the aftermath of high-speed gaseous collisions (emitting X-rays, in pink), doesn’t their failure to match up indicate dark matter? Not necessarily; it only tells us that there isn’t enough gaseous dark matter to explain all of the gravitational effects. But you can also see that the optical matter — the collections of stars in galaxies — passed right through one another, the same way that two guns filled with birdshot and fired at one another would have all but very few pellets miss one another.

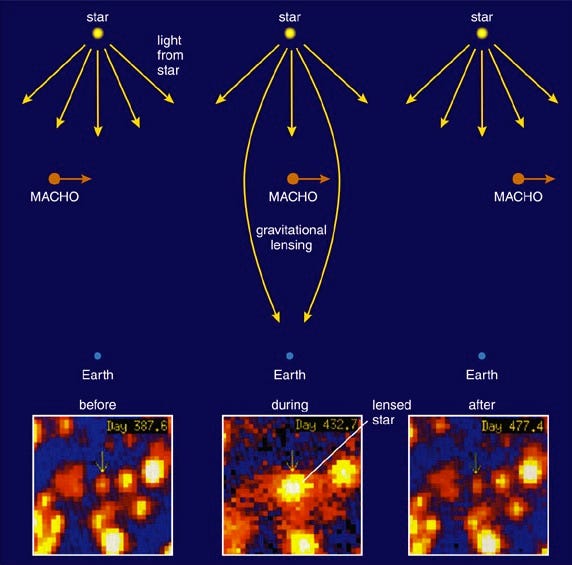
Following that line of thought, you might think that dark matter could be primarily Earth-sized objects, or Jupiter-sized, or Moon-sized. After all, objects that are smaller than the smallest stars (including brown dwarfs) won’t emit any visible light due to their own internal nuclear fusion, and so they’re technically “dark” matter as well. The interesting thing about objects like this is that, if they’re traveling through our galaxy, then every time one transited (passed between) our line-of-sight to a star, we’d get a microlensing event, where the background star briefly appeared to brighten and then returned to its original luminosity. We’ve observed these microlensing events, and we’ve found that for objects ranging from just a tiny fraction of the mass of the Moon and up, they can’t be more than a few percent of the dark matter.
But what, then, about basketball-sized objects? Or what about individual, isolated atoms? Or what about any other intermediate scale that we haven’t been able to (yet) rule out directly? In theory, they could still be the missing dark matter. This game of whack-a-mole, where we try and rule out each individual possible flavor of dark matter individually, is an important avenue of research, but one that’s insufficient for “proving” the existence of dark matter that isn’t made up of normal stuff: protons, neutrons and electrons.
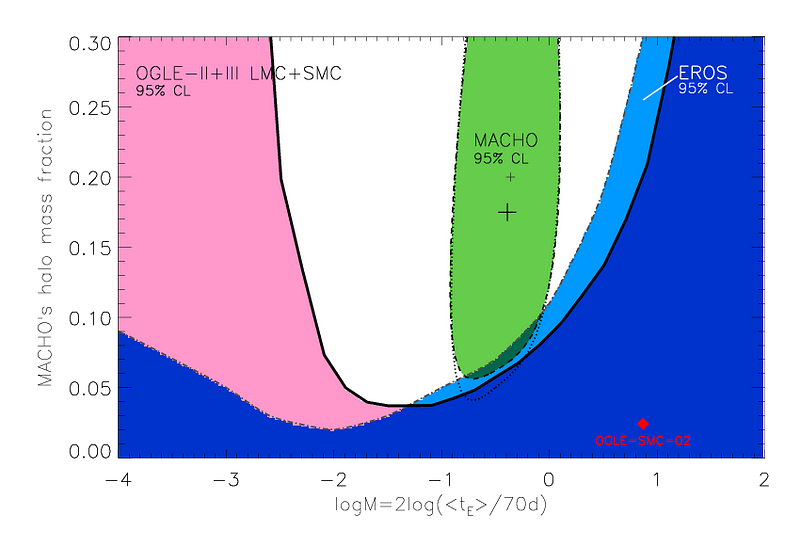
But there is a way to do exactly that! We can find out by a combination of the following methods:
- Measuring the total amount of matter of all forms in the Universe.
- Measuring the total amount of normal matter in the Universe. (Or, alternately, measuring the ratio of normal-to-total matter.)
- Seeing whether these two numbers match. (Or whether the ratio is “1” or some number less than 1.)
Let’s see how to do this.
There are a number of different physical phenomena we can measure in the Universe to figure out what the total matter density of the Universe is. For example, looking at galaxy clustering on the largest scales (using baryon acoustic oscillations, for example) is highly dependent on the total amount of matter. Looking at the cosmic microwave background’s fluctuations — the leftover glow from the Big Bang and its tiny imperfections — tell us how much matter-and-energy combined exist in the Universe. And looking at the redshift vs. distance data for objects in the Universe, and for Type Ia supernovae in particular, also set constraints on how much matter-and-energy of various types exist.
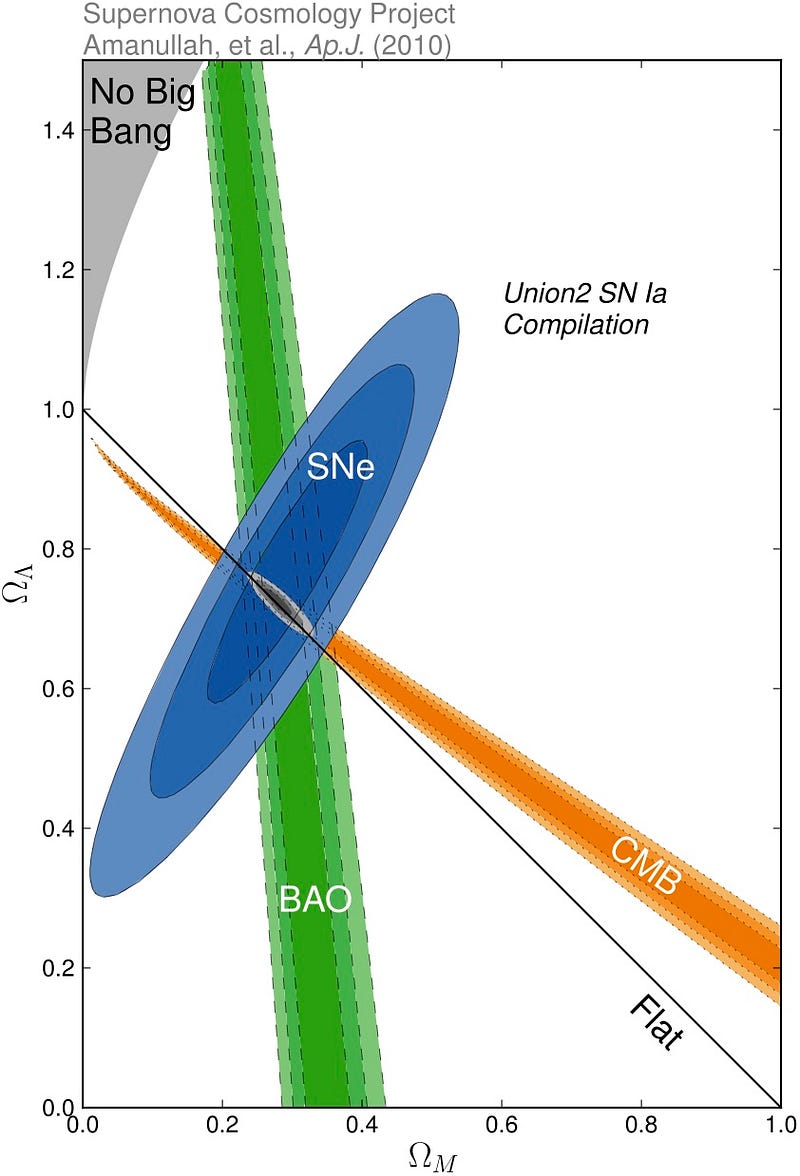
When we combine all that data together, we find that about 30-to-34 percent of the Universe’s critical density exists as some form of matter. So, that’s one of the numbers we need. But we can also figure out what percent of the Universe’s critical density exists as normal matter, or as protons, neutrons and electrons. You see, when the Universe was very young and hot, it couldn’t even form stable atomic nuclei; they would instantly be blasted apart into individual protons-and-neutrons by the hot radiation bath of the Universe. It’s only when the Universe cools beyond a certain point that nuclear fusion can proceed, building the lightest stable elements-and-isotopes in the Universe.
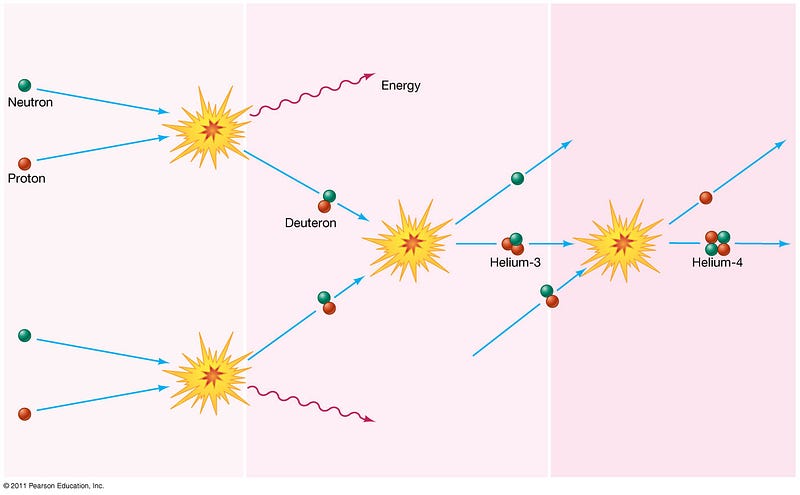
But what you might not realize is that the very specific ratios of elements that get created — how many protons, how much deuterium, how much helium-3 and helium-4, and how much lithium-7 comes to exist — is extremely sensitive to just one simple quantity in the Universe: the ratio of baryons-to-photons. (Where a baryon is either a proton or neutron, for these purposes.) We’re actually able to measure this from looking at molecular gas clouds that have been virtually untouched since the Big Bang, having suffered through negligible amounts of star formation.
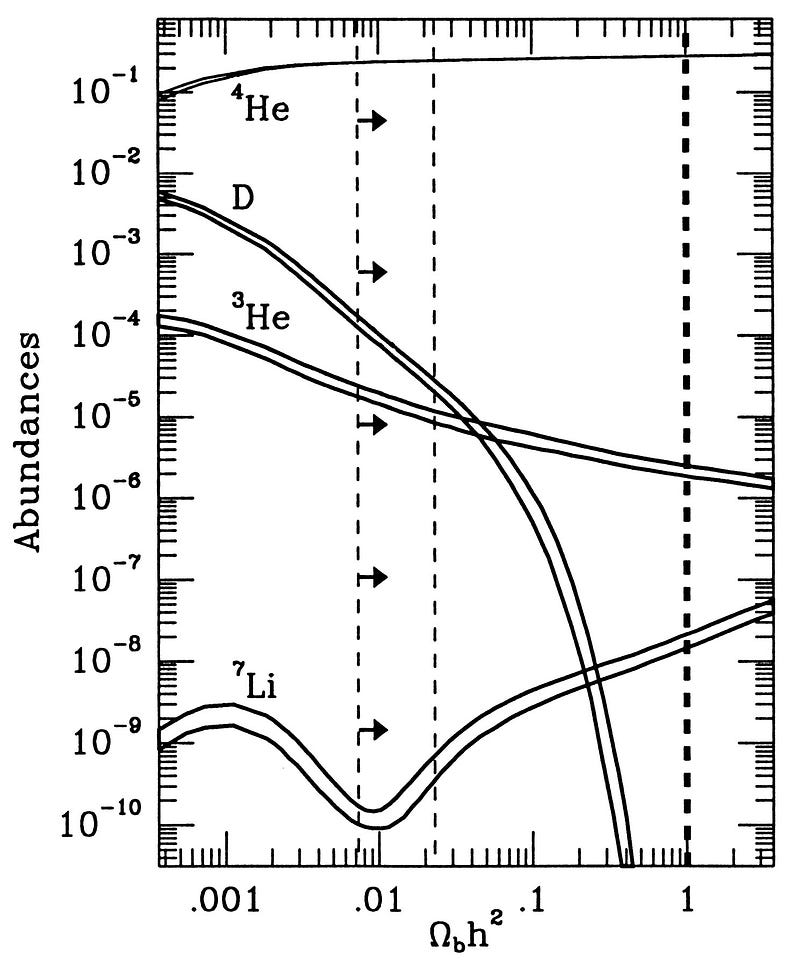
Since we can measure the original photon density (from the cosmic microwave background), figuring out the overall baryon density is relatively easy. What we learn from these observations is that about 4.5-to-5.5 percent of the Universe’s critical density exists in the form of normal matter of all types combined, meaning that whatever the other 25-to-29 percent of dark matter is, it can’t be normal matter of any type!
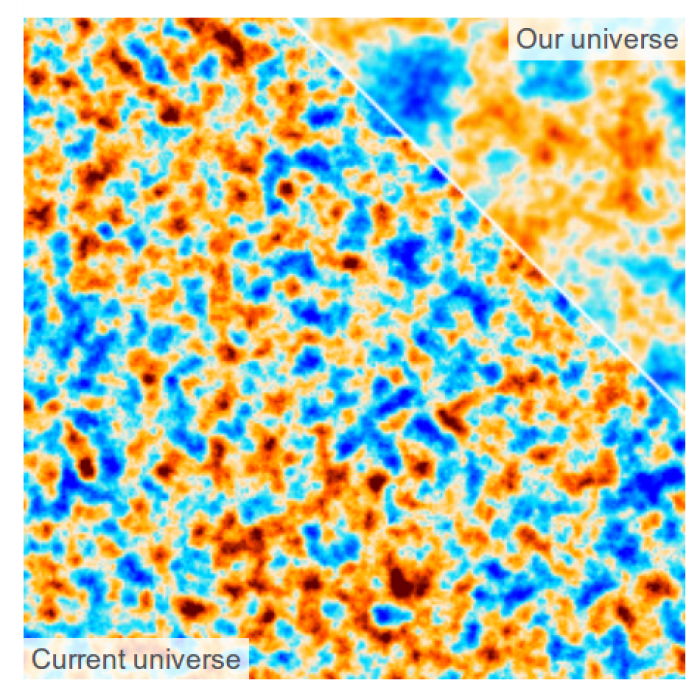
This is something that’s confirmed by observations of fluctuations in the cosmic microwave background, which matches the predictions of a mix of 5 percent normal matter and about 28 percent non-baryonic dark matter…
and from the power spectrum of large-scale structure, which has small “wiggles” in it, consistent with a normal matter-to-total matter ratio of about 1-to-6.
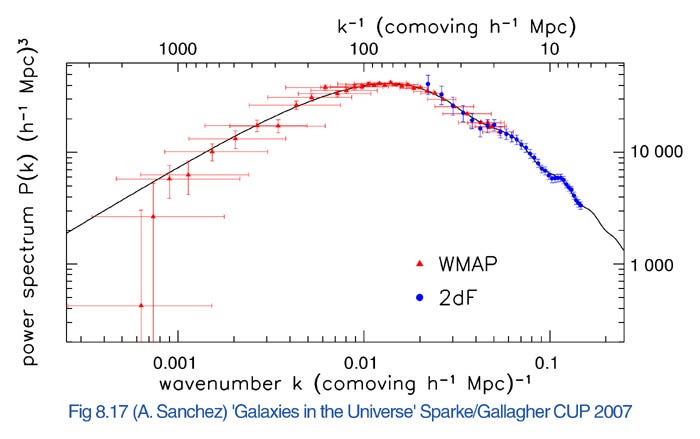
So no, dark matter can’t just be normal matter in some combination of forms that don’t emit light; there really needs to be something else out there that isn’t made of any of the particles in the Standard Model! Not only does dark matter exist, but it’s an entirely new type of matter, something we have yet to discover. The search for understanding just what it is that makes up the majority of the mass of our Universe continues.
Leave your comments at the Starts With A Bang forum on Scienceblogs!