Shortest-lived quantum particles exhibit quantum entanglement, too

Of all the spooky quantum phenomena in our Universe, perhaps the spookiest of them all remains quantum entanglement. The basic idea behind it is that particles don’t just exhibit this weird sort of indeterminism — where they propagate as waves, in indeterminate states, but interact like particles with definitive and measurable properties — but that the quantum state of even disconnected particles can be related to one another. When you measure the quantum state of one entangled particle, you instantly know something about the pair that it’s entangled with: not an exactly-determined state, but with sets of probable outcomes that are superior to mere random chance.

Quantum entanglement has been well explored for conventional particles such as photons, electrons, positrons, protons, neutrons, and other atomic nuclei. However, nearly all of these tests have occurred at relatively low (conventional) energies and for relatively stable (long-lived) particles. Does quantum entanglement work the same way at high energies, and/or for extremely unstable, short-lived particles?

With the technology available at the Large Hadron Collider (LHC), both the ATLAS and CMS collaborations, independently, have made the first measurements that indicate the answer: yes, yes it does. Working with top quarks, with a mean lifetime of just ~5 × 10^-25 s — the shortest-lived particles in the entire Standard Model — LHC scientists have now demonstrated quantum entanglement in two novel regimes for the shortest-lived particles of all. Here’s what we’ve learned.

When two particles are entangled in the quantum mechanical sense, it’s as though some sort of hidden, invisible connection exists between them, and that a seemingly “random” property of one member of the pair can be known to better than “random guessing” simply by measuring the properties of the other member. Entanglement, as far as we can tell, is instantaneous, although no actual information appears to ever be transmitted from one member of the pair to the other.

Quantum entanglement

Nature, let’s face it, is weird. There are all sorts of experiments that we can perform, on a quantum level, that reveal just how weird, spooky, and counterintuitive quantum behavior can truly be.

• Radioactivity: place a large number of radioactive atoms in a container and wait a certain amount of time. You can predict, on average, how many atoms will remain versus how many will have decayed with pretty good accuracy, but there’s no way to know which specific atoms will decay or remain. Quantum physics only gives us statistical probabilities, not definitive predictions, for individual particles in this regard.
• Double slit: fire a series of particles through a narrowly-spaced pair of slits and you’ll be able to predict what sort of interference pattern the particles will create when they land on a screen behind the slits. However, if you fire one individual particle through the slits, you can predict the statistical probabilities of where it will land, but you cannot determine its landing location without making the critical measurement.
• Spin deflection: take many particles that have an inherent spin to them (i.e., fermions) and pass them through a magnetic field, and you’ll see some will split “up” (aligned with the field) and others will split “down” (anti-aligned with the field). If you then pass them through a perpendicular magnet, their originally measured spin direction will become randomized; if not, their spins will be maintained.

This kind of quantum indeterminism, and the associated bizarre behavior, applies equally well to systems of particles as it does to individual particles.

When a particle with quantum spin is passed through a directional magnet, it will split in at least 2 directions, dependent on spin orientation. If another magnet is set up in the same direction, no further split will ensue. However, if a third magnet is inserted between the two in a perpendicular direction, not only will the particles split in the new direction, but the information you had obtained about the original direction gets destroyed, leaving the particles to split again when they pass through the final magnet.

But quantum entanglement is something fundamentally different, that adds a whole new layer to “spooky” behavior. Entanglement occurs when two (or more) particles are created, or a system involving them is prepared, in such a way that their quantum states are correlated with one another. Individually, each member particle of an entangled pair will exhibit the standard indeterminism and uncertainty of any quantum particle of its species: the same types of indeterminism and uncertainty described in the examples above. However, there’s an additional property that comes along with entangled particles: there are correlations between their quantum states that can only be seen when measurements of both particles are brought together.

The reason this is often referred to as so “spooky” is because of a property of Einstein’s relativity: the notion that nothing can ever travel through space faster than the speed of light. Photons, gravitational waves, and other massless, force-carrying particles must move at the speed of light, and hence, that’s the maximum speed at which information can be transferred. But for entangled quantum particles, you can separate them by even an arbitrarily large distance and then measure the quantum state of each member pair to as close to “simultaneously” as possible. When you do, you’ll find that the quantum state of both members, combined, exhibits correlations that appear to be “understood” by each member instantly.

Quantum mechanics’ entangled pairs can be compared to a machine that throws out balls of opposite colors in opposite directions. When Bob catches a ball and sees that it is black, he immediately knows that Alice has caught a white one. In a theory that uses hidden variables, the balls had always contained hidden information about what color to show. However, quantum mechanics says that the balls were gray, or a combination of black and white, until someone looked at them, when one randomly turned white and the other black. Bell inequalities show that there are experiments that can differentiate between these cases. Such experiments have proven that quantum mechanics’ description is correct, and the balls have an indeterminate color until the measurement is made.

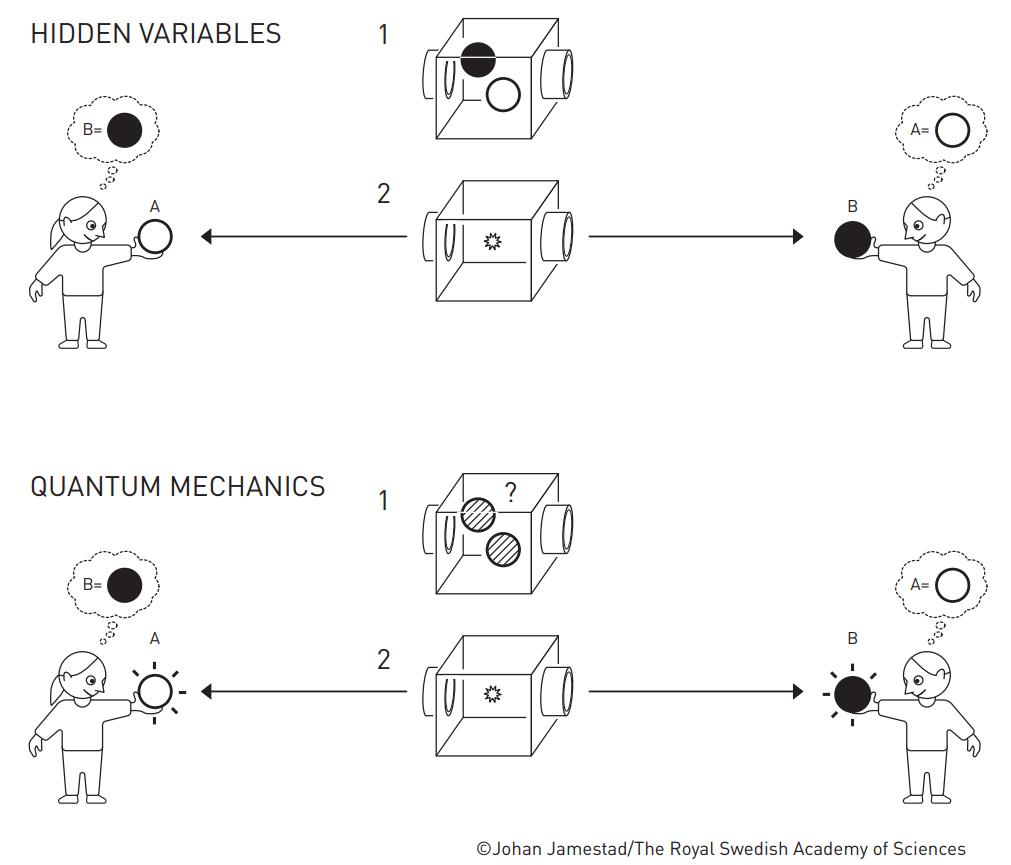
Conventional entanglement limitations

If you have an entangled pair of particles and you measure, for example, the spin of one of the member particles and determine it to be “spin up,” that doesn’t necessarily mean that the other member particle will be “spin down.” It could be “spin down,” but it won’t be “spin down” 100% of the time. If there were no correlations (and hence, no entanglement) between the two member particles, you’d only be able to predict the outcome of your spin measurement of the “other member” particle to 50% accuracy. However, with the correlations brought on by entanglement, you can predict the spin measurement of the “other member” to greater than 50% (but less than 100%) accuracy, depending on your specific experimental setup.

In 2022, the Nobel Prize in physics was awarded for quantum entanglement and its role in quantum information systems, where pioneers:

• John Clauser, who developed the first practical experiments to test quantum entanglement and was the “C” behind the CHSH inequality,
• Alain Aspect, who established violations of Bell’s inequality by more than 30 standard deviations and with correlations that allowed 83% accuracy (as opposed to Clauser’s 55% accuracy) in prediction,
• and Anton Zeilinger, who closed a number of loopholes in quantum entanglement experiments and also devised the setup that enabled quantum teleportation,

shared the 2022 Nobel Prize. However, entanglement was only demonstrated between the conventional particles mentioned earlier: protons, neutrons, atomic nuclei, electrons, positrons, and photons.

The experimentally measured ratio R(ϕ)/R_0 as a function of the angle ϕ between the axes of the polarizers. The solid line is not a fit to the data points, but rather the polarization correlation predicted by quantum mechanics; it just so happens that the data agrees with theoretical predictions to an alarming precision, and one that cannot be explained by local, real correlations between the two photons (that would result in straight, not curved, lines for predictions).

The LHC’s entanglement revolution: part 1

In September of 2023, the ATLAS collaboration — one of the two flagship detectors, along with CMS, at the Large Hadron Collider — released a result that was surprising to many: they claimed to have created top-antitop pairs of particles, and to have measured entanglement between them. It wasn’t a lot of entanglement, but they were able to reduce the entanglement to a single parameter, D, that is inferred by the angle between the charged leptons (electron/muon/tau or positron/antimuon/antitau) emitted by the decaying top and antitop quarks.

If there were no entanglement, D would be no less than -⅓, where D is defined as -3 multiplied by the average value of the dot product (cosine of the angle) between the charged-lepton directions.

However, if there is entanglement, D would be observed to be less than (or more negative than) -⅓, which means that all one has to do, in principle, is:

• collect enough top-antitop pairs that are created at the LHC,
• measure the opening angle between their decay products that include charged leptons,
• measure the original top-antitop pair’s decay products well enough so that you know how to do a velocity transformation into the rest frames of the parent top and parent antitop quarks,
• and then average your results together, once you have sufficient statistics, to determine a measured value for D.

The top graph shows the calibration curve for the detector, while the bottom graph shows the theoretical limit for the entanglement parameter D (on the y-axis) of -1/3. Note that, for the low-momentum case, the data (black), as well as standard model predictions (red and blue), all rule out a no-entanglement scenario.

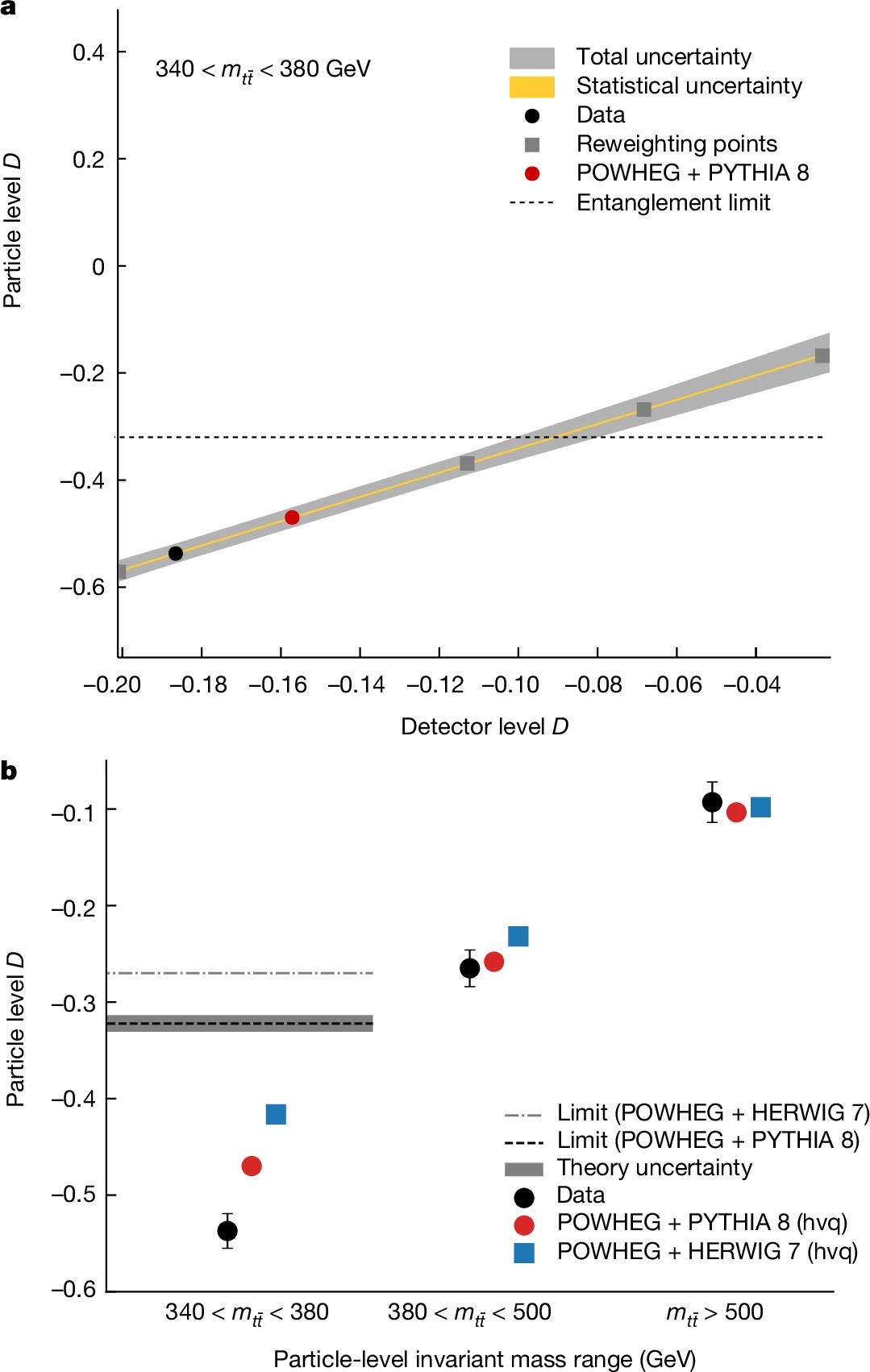
By calibrating their detector properly, the ATLAS collaboration was able to show that, for low-momentum (i.e., where they move very slowly compared to the speed of light) top-antitop production events, and not for higher momentum top-antitop production events, D is indeed not only “more negative” than -⅓, but it’s significantly more negative: around -0.537, with a statistical uncertainty of a paltry ±0.002 and a systematic uncertainty of ±0.019. This tells us, in the collaboration’s own impressive words from their Nature paper, that:

“The observed result is more than five standard deviations from a scenario without entanglement and hence constitutes the first observation of entanglement in a pair of quarks and the highest-energy observation of entanglement so far.”

In their paper, they then go on to diss their rival collaboration, CMS, by pointing to one of their older (2019) papers and noting:

“It should be noted that the CMS collaboration has already measured D = −0.237 ± 0.011 inclusively, showing no signal of entanglement.”

However, if the ATLAS collaboration had grouped all of their top-antitop events together and had simply taken an average, they wouldn’t have seen entanglement either.

This plot shows the boundary for entanglement in the parameter D at right, along with the simulated (violet, blue, yellow) Standard Model predictions and the data (black points with error bars) from the CMS collaboration. The experimental values for D, being significantly more negative than -0.333, show clear evidence for entanglement in top-antitop events.

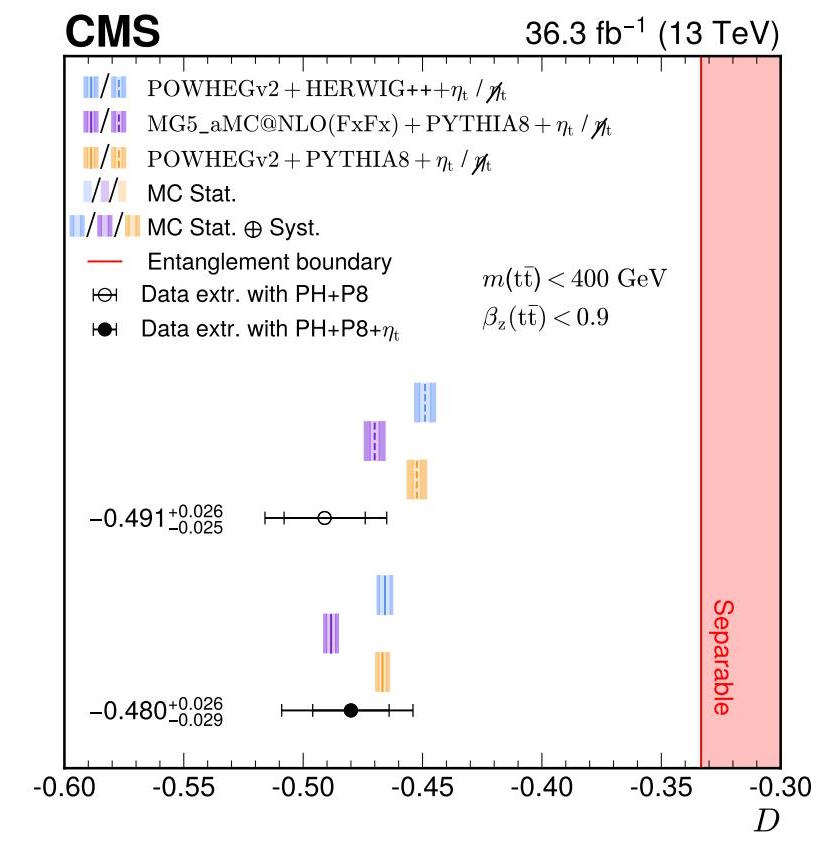
Top quark entanglement from CMS

Back in June of 2024, the CMS collaboration came out with a new preprint of a paper where they noted how the approach of grouping all of their data would not reveal entanglement, and instead undertook an analysis to restrict themselves to low-momentum top-antitop production events, just as the ATLAS collaboration had done the year prior. This time, with the data set restricted to the relevant set of events, they not only observed quantum entanglement and measured a D that was indeed below (and more negative than) -⅓, but they found something that was both consistent with the ATLAS results and also crossed the vaunted 5-sigma threshold (just as the ATLAS results did) for demonstrating the necessity of entanglement.

The results, as shown here, show that D is either -0.491 or -0.480, depending on which types of relevant data were included or excluded, with uncertainties largely driven by systematics that were at the ±0.026 level or thereabouts. Although the detector differs, the data chosen differs (as CMS uses a maximum rest energy plus kinetic energy of the top-antitop pair of 400 GeV as compared with ATLAS’s 380 GeV), and the analysis differs, the results of the two collaborations are in pretty good agreement. Moreover, both of them contradict the “no entanglement” interpretation. For the first time, in exotic, short-lived top quarks, quantum entanglement has been demonstrated.

With the experimental inputs of the determined masses of the top quark, the W-boson, and the Higgs boson all shown on the same graph, tests for their mutual compatibility and their consistency with the Standard Model. With ATLAS (or, now, CMS) instead of the disputed CDF data, they all align brilliantly.

Top quark appreciation

It’s been around 30 years since the first scientific announcements that showed evidence for the top quark’s existence were made: way back in 1994. In the time since, we’ve honed in on the top quark’s properties, but two of the most remarkable are these: it’s the shortest-lived particle known to humanity, and the most massive particle in the entire Standard Model.

The top quark, as mentioned at the start of this piece, has a mean lifetime of just ~5 × 10^-25 s: or one-half of a yoctosecond. This is incredibly important in particle physics, because the amount of time it takes quarks (or antiquarks) to form a hadron, such as a meson, baryon, or antibaryon, is a little bit more than one full yoctosecond. Top (and antitop) quarks are the only species of quark that, once you create them, don’t bind into composite particles with other quarks or antiquarks (what physicists call “hadronize”), but instead just decay.

This short lifetime means something profound for the top (and antitop) quark’s mass: it cannot be known exactly. Just as there’s an inherent uncertainty between a quantum particle’s “position” and its “momentum,” there’s also an uncertainty between a particle’s “energy” and its “lifetime.” Because the top quark is the shortest-lived particle of all, it means that it has the greatest uncertainty in the amount of energy inherent to it, and hence, in its mass (via E = mc²) as well. The top quark may have an average (energy-equivalent) mass of around 172.5 GeV, but most top quarks will either be somewhat larger or smaller than this value owing to this quantum uncertainty.

This graph shows a series of top quark events that result in jets. The top-antitop production contribution is highlighted in red, while the total signal is shown in green and the jet background (not involving top-antitop events) is shown in blue. Note how the mass of the top quark is not constant at ~172 GeV, but rather is spread out over a wide range between ~150 and ~195 GeV before dipping below the background.

The short lifetime comes along with another consequence as well: it’s the only Standard Model particle whose lifetime is shorter than what we know as the spin decorrelation timescale, which is specific to each particle and depends on its mass. For the top quark, even though that timescale is still incredibly short (at ~10^-21 seconds), the top quark’s lifetime is even shorter, which means that the spin information of top-antitop pairs that are produced gets preserved in the angular distribution (which includes spin information) of its decay products.

A second, high-momentum test of entanglement from CMS

If you study both polarization and spin correlation in the decay events that result from top-antitop pair production, you should indeed be able to test for the imprint of quantum entanglement, even once the originally produced top-antitop particles have decayed away.

In a second paper, the CMS collaboration undertakes a much less straightforward analysis that looks at very high momentum top-antitop systems — one where the top-antitop quarks have a total of at least 130% the kinetic energy of their rest-mass energy — and measures their decay products once again. This time, the analysis reveals a level of entanglement again between the pairs that cannot be explained by a no-entanglement scenario. For not only low-momentum systems, but very high-momentum systems, it appears that quantum entanglement applies even to unstable, heavy quarks like top-antitop pairs.

This graph shows the observed levels of entanglement, characterized by the y-axis markings of ∆E, which becomes very large in the high-momentum regime. Compared to the critical value for the no-entanglement case (blue dotted line), quantum entanglement is clearly present in the high-momentum states.

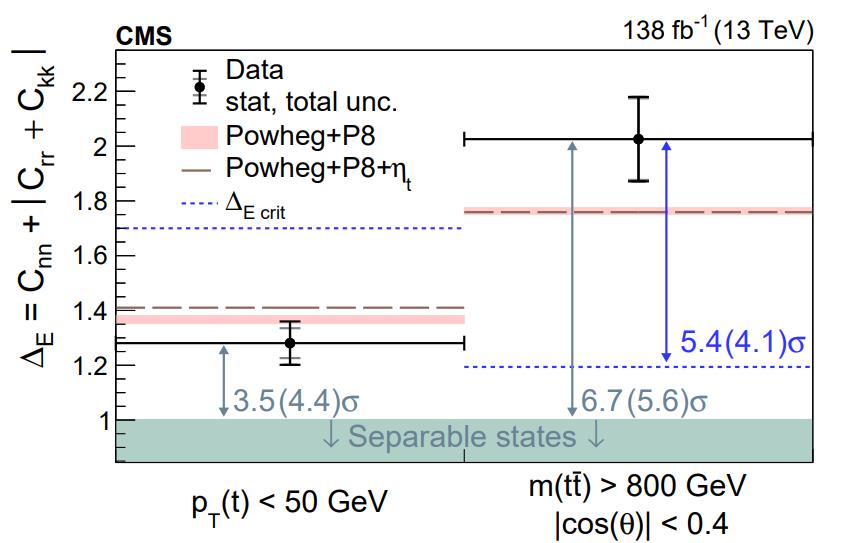
Of course, making the discovery of quantum entanglement in exotic, short-lived quantum particles is no surprise: quantum entanglement ought to be a fundamental property of nature. Although it’s difficult to measure, the ATLAS and CMS collaborations have proven themselves up to the challenge, and have now demonstrated quantum entanglement between the heaviest, shortest-lived particles in the entire Standard Model, top-antitop pairs of quarks. One of the tasks on the short-term horizon will be to do an apples-to-apples comparison, and to determine whether the ATLAS and CMS results are consistent with one another.

ATLAS, remember, measured D in the low-momentum regime to be -0.537, with an uncertainty of around ±0.020, while CMS measured D in a similar low-momentum regime to be -0.480 or -0.491, depending on which data set is used, with again an uncertainty of around ±0.026. These results are close, but more work will be needed to determine whether they mutually point to the same level of entanglement.

And what about the high-momentum regime? What about other high-mass, short-lived Standard Model particles, like the Higgs boson or the W-and-Z bosons? Do they exhibit quantum entanglement as well, and is there an even stronger test we can perform to measure it? Nature often has surprises lurking in the most difficult-to-access corners of reality. By finding quantum entanglement at these unprecedented high energies, it’s yet another victory for our standard picture of reality.