Ask Ethan: Could The Shape Of Our Universe Be Closed Instead Of Flat?

The shape of our Universe has long been recognized to be flat. But that isn’t the only possibility.

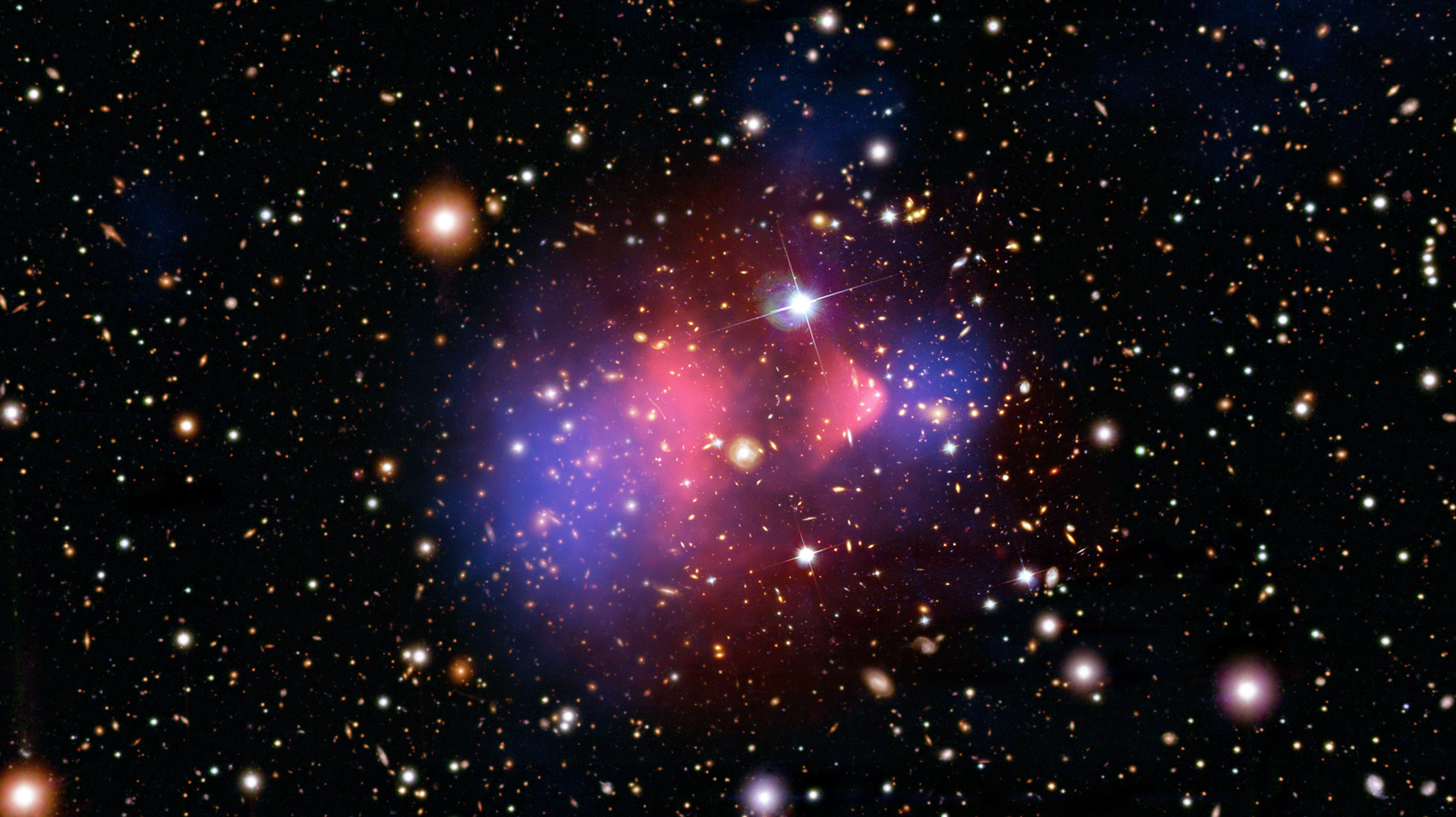
The Universe is expanding, and goes on for farther than even our most powerful telescopes can see. The part of it that we can see, scientists have long concluded, is indistinguishable from being perfectly spatially flat. At least, that’s been the conventional wisdom for a long time. But a couple of weeks ago, a new paper (full, free version here) used the latest data from the Planck satellite to draw the opposite conclusion: perhaps the Universe isn’t flat at all, but curved with a particular, closed geometry. Is this possible? Many have asked, including Tom Ensalata, who wants to know:

I thought that the curvature parameter had been essentially settled upon by WMAP, Planck, and other astronomical measurements. I am curious what you think about the validity of this recent paper. Is the Universe actually closed with a detectable positive curvature as the authors of the Nature Astronomy paper suggest? If the Universe is spherical, then how big would the sphere be according their measurements?

There’s a lot to unpack here, so let’s start at the beginning: with the idea of spatial curvature itself.

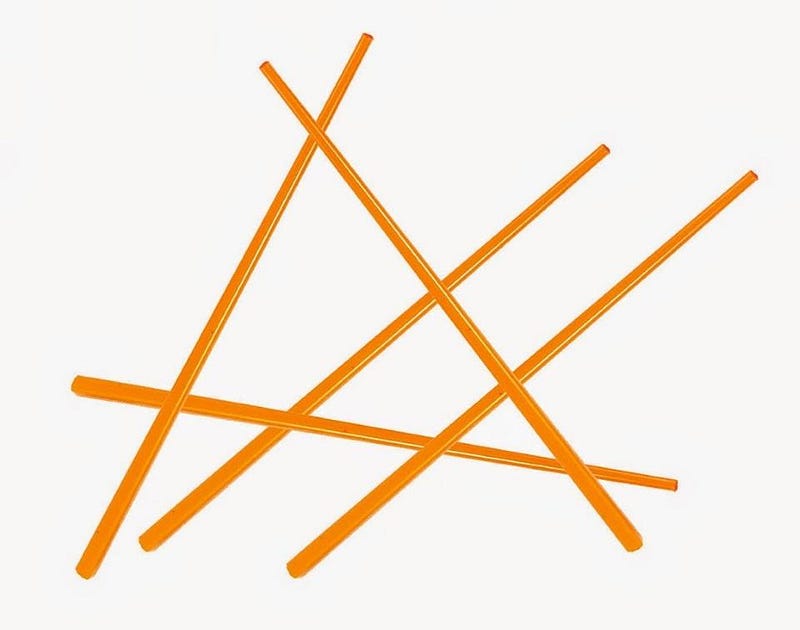
Flat space is what we’re used to working in. Draw a triangle on a sheet of paper, and you can be confident of a number of facts about it, including that the sum of its three internal angles will always add up to 180 degrees. Whether it’s a right triangle, isoceles, or scalene doesn’t matter; by the virtue of having three sides and existing in a flat, two-dimensional plane, its internal angles will always add up to that same value.

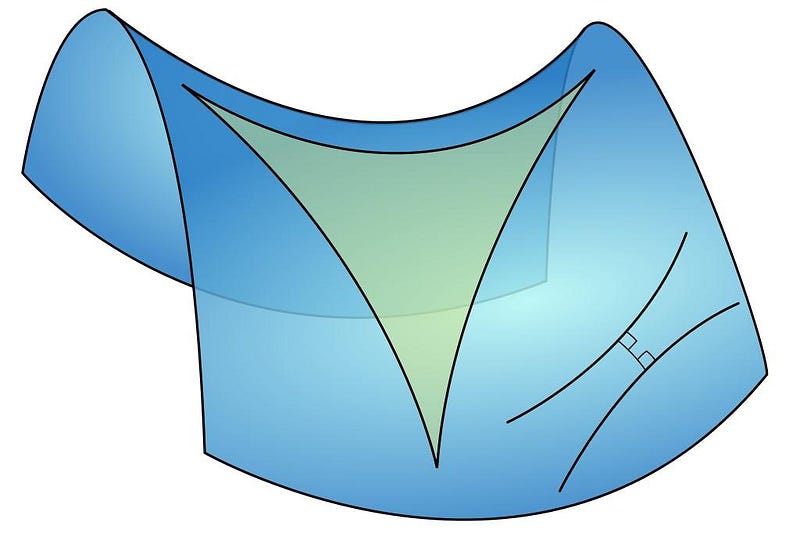
Unless, of course, your two-dimensional plane isn’t flat at all. If you cut a triangular section out of your paper and taped it back together, you’d discover that the triangle you now drew would contain more than 180 degrees; you would have created a surface of positive curvature. Insert that triangle into another flat sheet of paper, and you create a surface of negative curvature; any triangle you draw will contain fewer than 180 degrees.

While a two-dimensional plane can be flat, it can also be curved, either positively (like a sphere, where a triangle’s angles are greater than 180 degrees) or negatively (like a saddle, where a triangle’s angles are less than 180 degrees). This applies not just two any two-dimensional surfaces we can imagine, but also to higher-dimensional spaces as well.

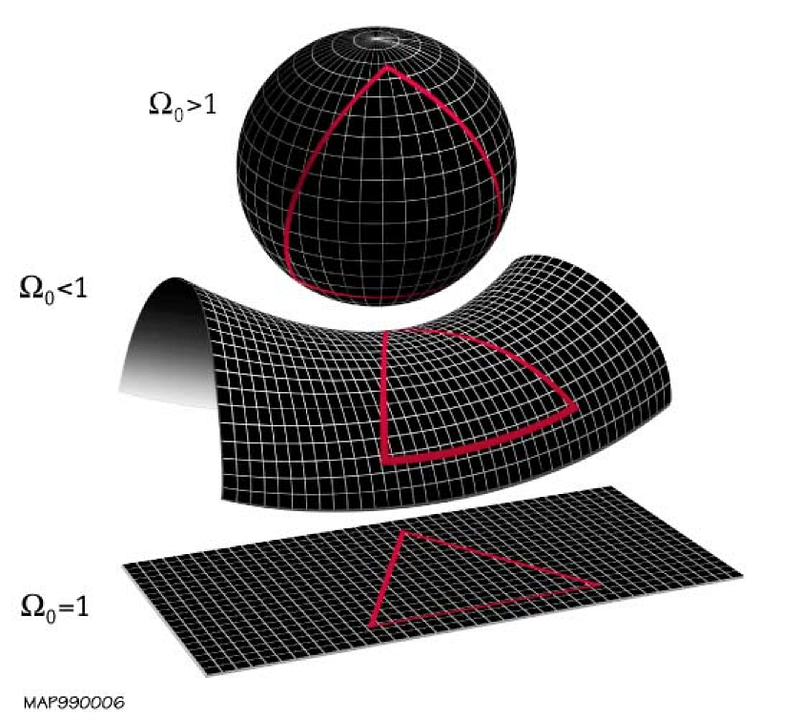
When we consider our entire Universe, we have three spatial dimensions, but again they can have any type of curvature at all: positive, negative, or flat. A positively curved Universe can be sphere-like in nature, but still expand or contract as the Universe evolves.

In fact, if the Universe were made of matter only (instead of also having radiation and dark energy), whether a Universe is curved positively, flat, or negatively determines whether the Universe is closed (and will recollapse), critical (on the border between recollapse and expansion forever), or open (destined to expand forever).

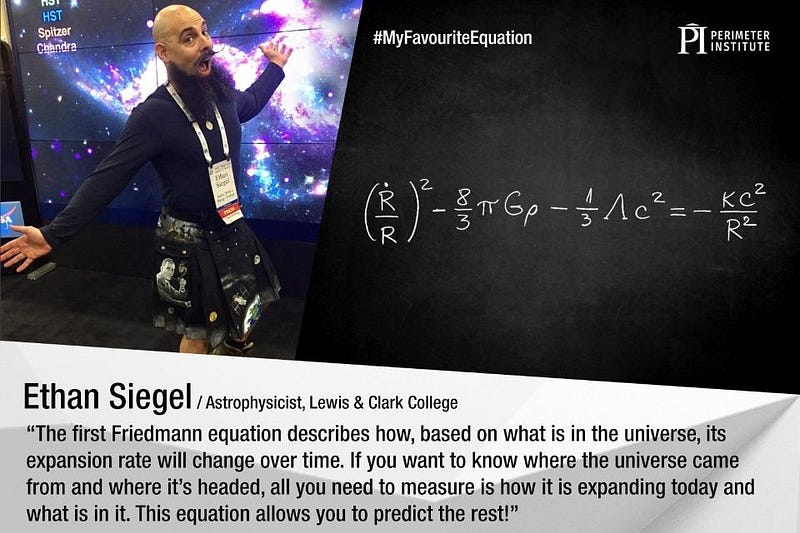
The reason for this is simple: the Universe, as we know it, is roughly (on the largest scales) filled with the same amount of stuff no matter where you go. At all locations and in all directions, the matter, radiation and energy in the Universe is the same. In the language of mathematics, the Universe is isotropic (the same in all directions) and homogeneous (the same everywhere). When we apply those properties to General Relativity, we get a unique and powerful set of equations that defines how the Universe will evolve over time.

On one hand, we obtain how the scale of the Universe changes with time: either an expansion or a contraction rate. On the other side, we have all the different forms of matter and energy in the Universe. And, if there’s any mismatch, the leftover balance goes into spatial curvature, yielding a flat Universe if and only if the expansion rate and the overall energy density match precisely.

From the moment the expanding Universe was first derived, it was known that if the Universe wasn’t perfectly flat, it was at least close. A Universe that was too severely curved, either positively or negatively, would either recollapse almost immediately or expand off into oblivion so quickly that the formation of stars or galaxies would be impossible. But there was nothing mandating that the Universe needed to be exactly flat; it would rely on measurements to give us that information.

As it turned out, our first robust measurements came courtesy of the Cosmic Microwave Background. In the late 1990s, the BOOMERanG experiment was groundbreaking in this regard, determining that the Universe was at least very close to spatially flat. The way it did so was straightforward, simple, direct, and extremely compelling.

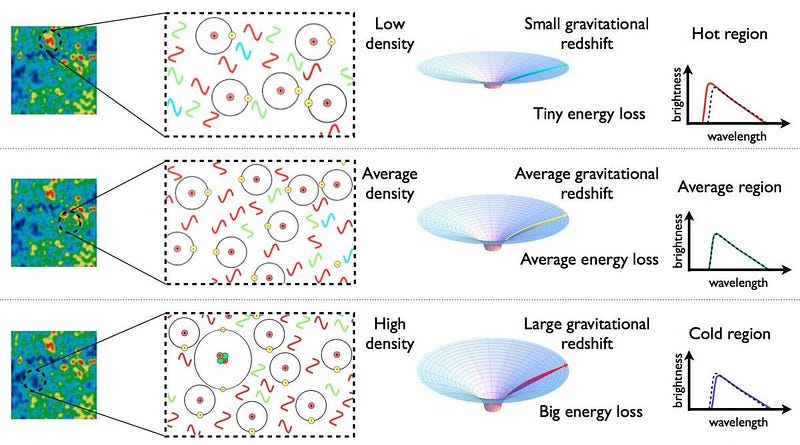
The Cosmic Microwave Background, you see, is the leftover glow from the Big Bang. Although, at first glance, it appears to be a uniform 2.725 K in all directions, a closer examination reveals that there are imperfections at about the ~100 microkelvin level. These imperfections aren’t because the Universe is fundamentally hotter or colder in one direction than the other, but rather because there are density imperfections that exist throughout the Universe.

Where you have an overdense region (with more matter than average), the light will have a harder time climbing out of that gravitational potential well, and hence it loses more energy than average and appears colder. Similarly, it’s easier than average for light to escape an underdense region, and those spots appear hotter than average. By looking at the angular scales of these temperature fluctuations, we can reconstruct the geometry of the Universe.

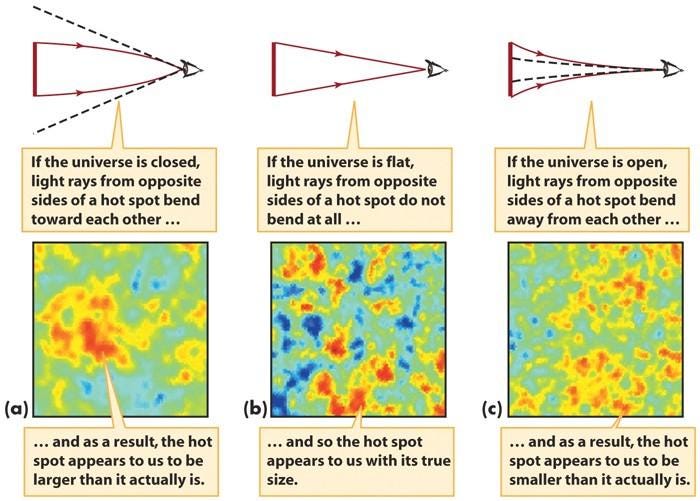
The results from BOOMERanG were spectacular, indicating a flat Universe, and this only got better as our measurements of the Cosmic Microwave Background improved. WMAP taught us that the Universe was flat to about the level of around 10%, and with Planck, that improved to about 2%. Combined with the data from supernovae and large-scale structure, it became clear that a flat Universe was the best option of all.

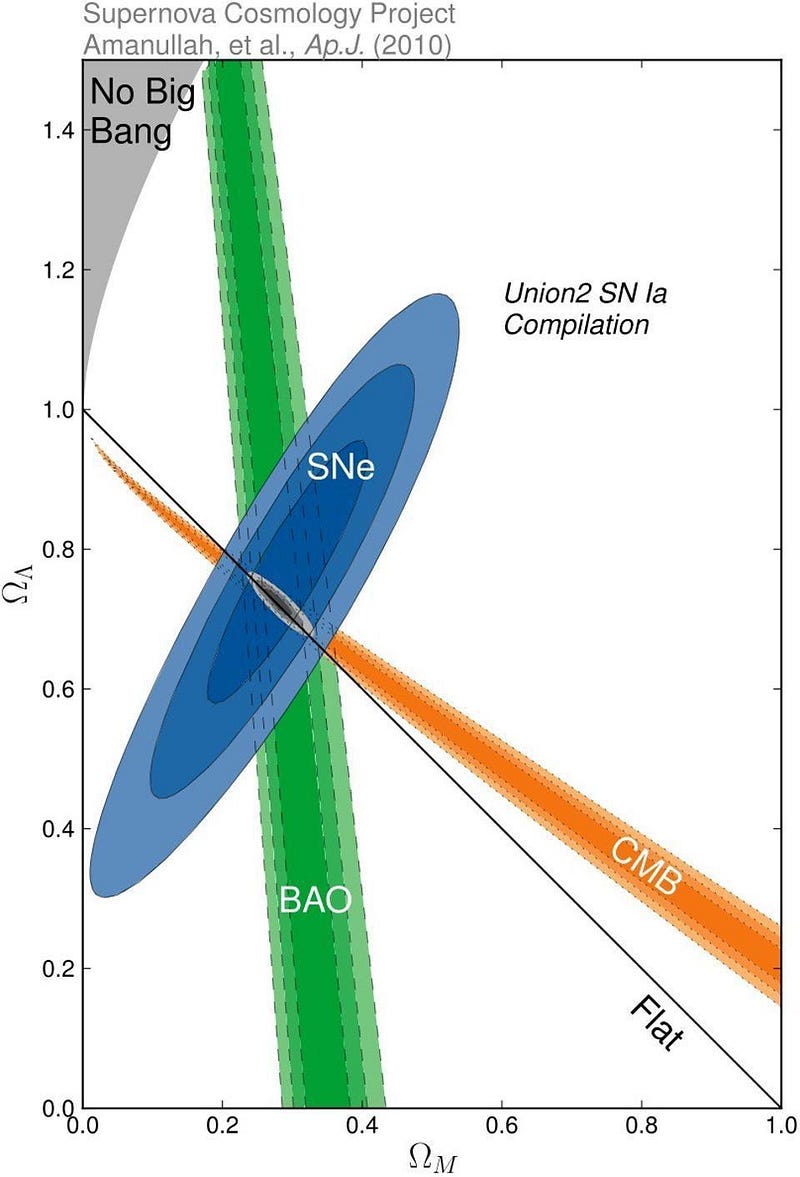
However, it would be unfair to say that the Cosmic Microwave Background demonstrated that the Universe was unambiguously flat, since the patterns of temperature fluctuations that it revealed had multiple possible solutions. The Universe could be expanding somewhat faster or slower at the expense of adjusting some of the parameters; a Universe that was slightly either closed (and overdense) or open (and underdense) were still permitted. There is wiggle-room with the CMB alone.

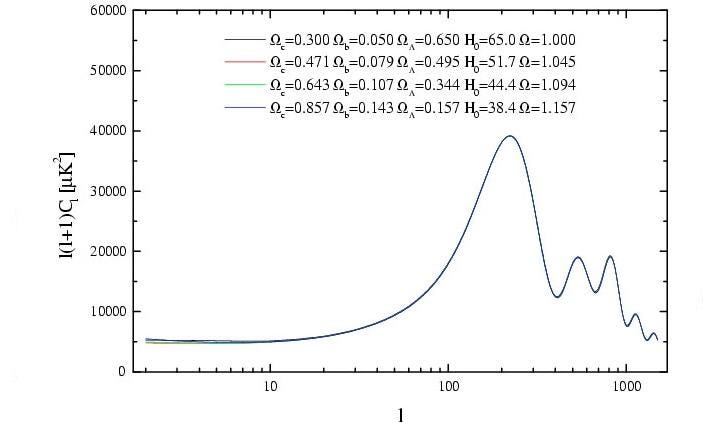
In the new analysis that calls the CMB’s results into question, the authors argue that it isn’t the temperature power spectrum (the wiggles, above, that you see which relate the magnitude of the mean temperature fluctuations to a particular angular scale) that favors a closed Universe, but rather a different extracted signal: the enhancement of a higher-than-expected lensing amplitude.

Gravitational lensing is a cumulative effect of having matter in between your observation point and the source you’re measuring: in this case, the Cosmic Microwave Background itself. The identification of a stronger-than-expected lensing signal, one possible interpretation of the Planck data, suggests that there’s more matter density than previously expected. If there’s more matter than other indicators suggest, perhaps that means the Universe is closed and overdense, and there’s a slight amount of (positive) spatial curvature.

The authors note — importantly but controversially — that a number of other anomalies might fit in perfectly with this. A closed and overdense Universe could explain why the temperature fluctuations on the largest angular scales (corresponding to scales of ~30 million light-years or so) are lower than expected. In addition, changing the curvature and energy content of the Universe changes the preferred value of the Hubble constant.

Given that the Cosmic Microwave Background prefers a value of about 67 km/s/Mpc, while distance ladder methods prefer 73 km/s/Mpc, it’s reasonable to hope that playing with this extra wiggle-room might help solve a large number of problems. When the authors run their analysis, they find that the best fit to all the data involves a slightly overdense Universe with positive curvature at the 4.4% level, achieving about a 3-sigma statistical significance favoring this value.

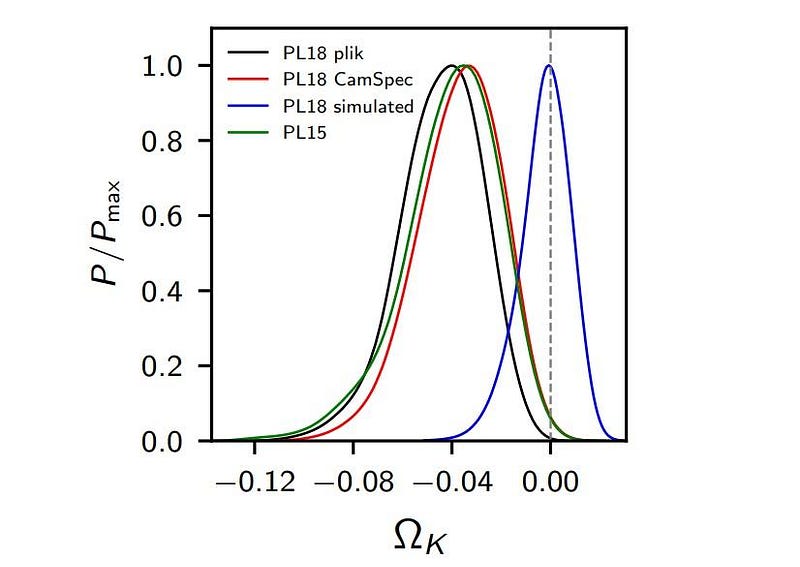
Unfortunately, that’s where the good news for this alternative interpretation ends. If you make these alterations to your cosmological model, the tension in the Hubble constant gets far worse, as adding more matter and closing the Universe forces you to even lower — I daresay absurdly low — values of the Hubble constant: values in the mid-50s.

Additionally, the best constraints on spatial curvature no longer come from Cosmic Microwave Background experiments, but from a different source: measurements of baryon acoustic oscillations. By mapping the large-scale structure of the Universe and determining how galaxies clump, cluster, and correlate on large scales, we’ve been able to constrain the curvature of the Universe to ~0.4% precision. When we use that data, we find that the Universe is perfectly spatially flat, and that a curvature of ~4.4% is ruled out at greater than 10-sigma significance, something the authors themselves acknowledge.

In science, it’s always fun to play around with alternative explanations and interpretations of your data, particularly when there are a few facets you cannot explain with the most common, conventional model. However, adding in a bit of extra matter and a bit of extra curvature, as attractive as it might be as a potential fix for a few cosmological puzzles, falls apart dramatically upon close inspection. The Universe’s expansion rate and galaxy clustering properties are all wrong for a closed Universe: catastrophically so.

The idea that the Universe may not be perfectly spatially flat is one that we must always keep in mind when we do our analysis, but one that we ought not to take seriously unless it’s compatible with the full suite of cosmological evidence. This new analysis presents an interesting tension in a novel way, but a closed, overdense Universe cannot be the solution. As is so often the case, this simple solution to an unexplained phenomenon creates far more problems than it solves.

Send in your Ask Ethan questions to startswithabang at gmail dot com!