Does time really exist?
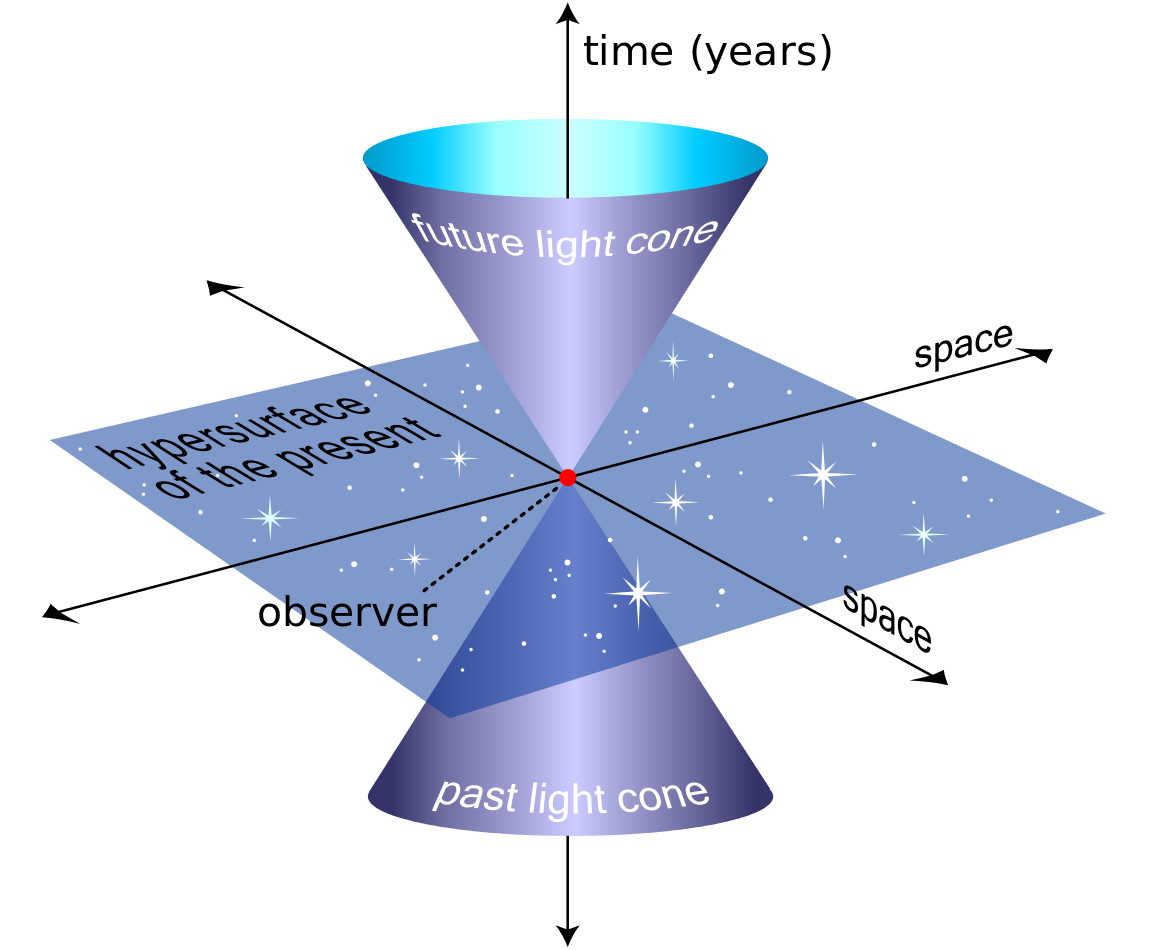
- If you want to describe precisely when and where something takes place, you need four coordinates: three spatial ones and one temporal one, i.e., time.
- Einstein taught us that time is relative for each and every observer, and there’s no such thing as “absolute time”.
- Some go a step further and claim that time is merely a persistent illusion. Can the case be made that time doesn’t even exist?
In a philosophical sense, we’re taught to doubt and question everything. Even the reality of ourselves and our own experiences are up for debate, as we have to make certain assumptions about how trustworthy our sensors — and our own senses, for that matter — actually are in order to arrive at any satisfactory conclusions. Sure, certain things might appear real, but isn’t it possible that those appearances are deceiving, and that quantities or concepts that we take for granted might be nothing more than very convincing illusions?
From a physical, scientific perspective, however, these sorts of questions take on a different meaning. We’ve learned lots of surprising and counterintuitive lessons from our investigations of time. Time is relative, not absolute. Time always marches forward, not backward, but we still lack an explanation for the arrow of time. Thermodynamically, the Universe has an arrow of time, which “flows” in the same direction as increasing entropy. And when we investigate the Universe on a fundamental level, it turns out that time may not be fundamental at all.
But existence itself? It’s very, very difficult to take that property away from time and to still wind up with a Universe consistent with what we observe. Here’s why.

When it comes to the question of existence, physics is very simple and straightforward about what it considers to be a satisfactory answer.
- Can you measure it?
- Can you quantify it?
- Can you define it in a mathematically self-consistent way?
- Is it, itself, an observable quantity, and do other observables depend on it in an inextricable way?
If your answers to these questions are all in the affirmative, there’s no way out of it: you’ve got yourself a quantity that exists.
The reason why is simple: as far as reality goes, “what is real” are those things that themselves are measurable, observable, quantifiable, and not pathological. In layperson’s terms, pathological is what happens when you pose a reasonable question to the Universe and you get inconsistent nonsense back. There are plenty of questions that do yield pathological behavior, and in those instances, the pathologies indicate to us that we have further work to do. “What happens at a black hole’s central singularity?” “What happens to quantum fluctuations on length scales smaller than the Planck length?” “What happens when a mass travels through the spacetime that is distorted by the presence of that mass itself?” These are all questions that, at present, are as pathological as dividing by zero.
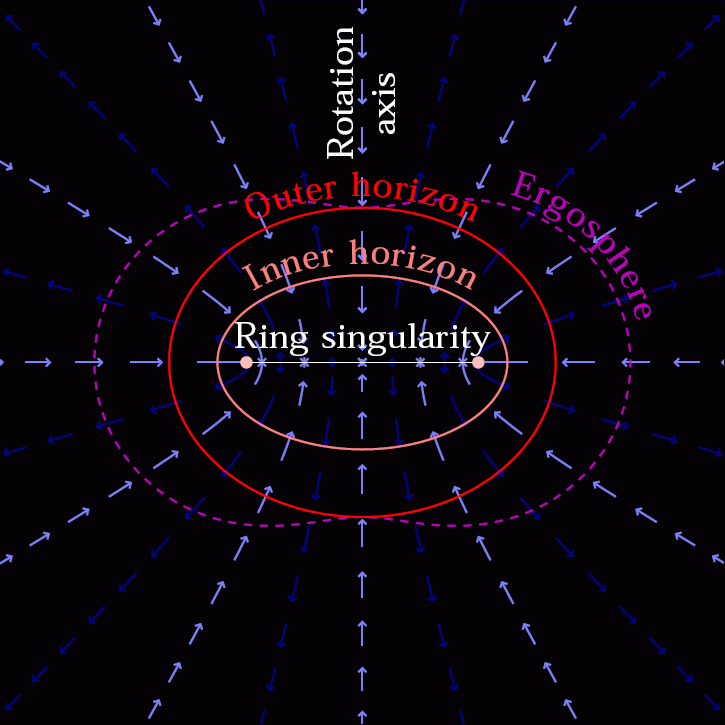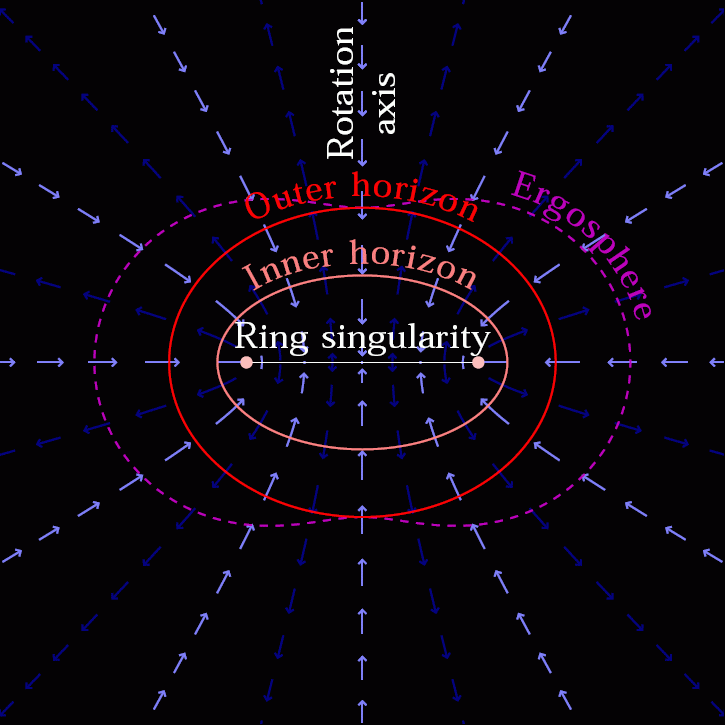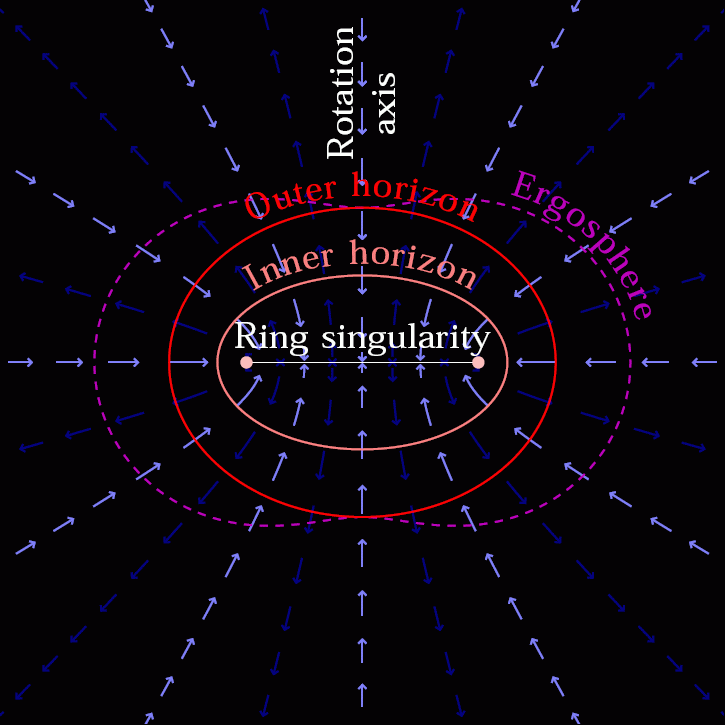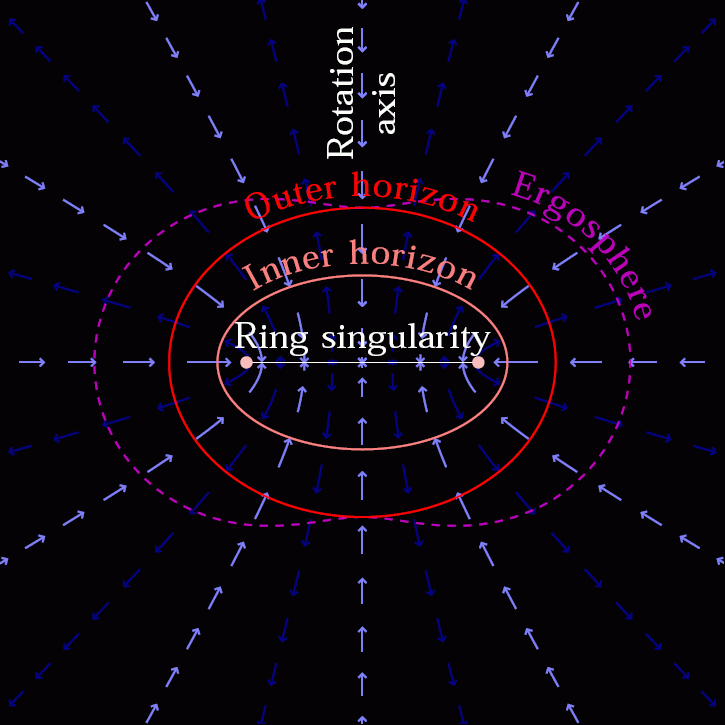
You might think, then, that perhaps time itself is pathological. Sure, we can measure it, quantify it, and even observe both its passing and the consequences of its passing. But shouldn’t it matter that your measurements of “how much time has passed” between the start and end of an event depends entirely on where you are and how you’re moving when you’re making those observations?
For example, if you’re on a moving train and you shoot a light wave from one end of the train to the other, you’ll get a value for how long it takes the light to reach the far end of the train. If you’re on a platform, however, watching the person on the train shoot the light from one end to the other, you’ll get a different answer.
For the person on the moving train, they’ll measure that a certain amount of time must elapse for the light to travel down to the far end of the train. But for the person on the ground, they’ll not only get a different, longer answer, but they’ll conclude that the person (and, for that matter, everything) on the train is actually aging more slowly than they are. To the stationary observer, an object in motion ages more slowly than an object at rest.

Is this paradoxical? Is it pathological?
Not at all. Noting that time is “relative” doesn’t mean it’s pathological. For our question about light traveling from one end of a moving train to the other, it’s possible that the train can come to rest, and the “on the ground” and the “on the train” observers can meet up again. Both of their measurements will be different, individually, but they’ll be consistently different with one another. When you perform the calculations for how much time passes for one observer relative to the other, each observer will be able to correctly predict not only what their own watches and clocks say, but the other observer’s as well. All it takes is a knowledge of special relativity.
Yes, you get different answers to the question of “How much time has passed?” or “When did this event occur?” or even “Which event happened first?” depending on where you are and how you’re moving, but no one is more “right” or “wrong” than anyone else. Instead, we just need to transform our idea of time — according to the laws of relativity — to match what someone at either a different location or moving at a different relative speed would conclude.

So, then, the notion that “time is relative” isn’t sufficient to claim that time doesn’t exist. But could it be the case that, perhaps, we only perceive time to exist, and that it isn’t, in fact, actually real?
We can consider this from a particular perspective: looking at the notions of symmetries in physics. After all, the laws of physics, at least as we know them, are time-symmetric. If you watch a ball falling under the influence of gravity, you have no idea whether:
- you’re watching time running forward as gravity pulls the ball down from a dropped position high above where you’re looking now,
- or whether you’re watching time running backward as a ball, having been thrown upward from a lower position, is rising higher and higher as the force of gravitation resists its motion.
In fact, almost all of the laws of physics — including motion, gravitation, electromagnetism, and even the strong nuclear force — are completely time-reversible. They are the same forward and backward in time, and you cannot discern, simply by watching a physical system unfold, which one is occurring.

But there are two ways to identify a physical difference between progressing forward in time and backward in time. The first is by looking at reactions that proceed via the weak nuclear force, such as radioactive decays.
Let’s imagine that you have a heavy atomic nucleus, full of protons and neutrons. If there are a large number of neutrons in that nucleus for the given number of protons that are present, there’s a chance that the nucleus will undergo a specific type of radioactive decay: beta decay. Beta decay is what happens when one of the neutrons in the nucleus decays into a proton, an electron, and an anti-electron neutrino, and it even happen for free (unbound) neutrons that aren’t part of any larger atomic nucleus.
It will often happen that a neutron decays into a proton, an electron, and an anti-electron neutrino. But it never happens that a proton, an electron, and an anti-electron neutrino spontaneously react together to form a neutron. In fact, in a variety of ways, the weak interaction is the poster-child for time-asymmetric reactions in physics.

The second way, however, is even more familiar to most of us. Every time you:
- scramble an egg,
- drop a full glass of water onto the ground and watch it shatter,
- or simply open the door between a hot room and a cold one,
you are creating a situation where there will be a thermodynamic arrow of time.
You may have heard of the concept of entropy before, which is often incorrectly defined as a “measure of disorder” of your system. But really, what’s going on is this: any physical system will have inside of it, some level of energy gradients. If you have an unscrambled egg, there’s an energy gradient between the albumen (the white part) and the yolk; the barrier around the yolk is what keeps things from mixing evenly. In an uncooked egg, there’s chemical potential energy that will be released — and new bonds will form — if you cook the egg. There’s potential energy in the structure of the glass, and shattering it will release it.
But perhaps, of all the examples, considering a hot room and a cold room right next to each other is the smartest way to talk about entropy.
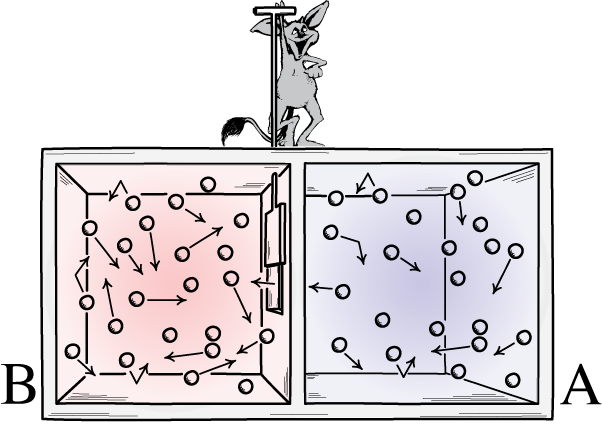
If you have a large number of particles on the hot side of the room, they’ll all be in what we call thermal equilibrium with one another. As they bounce off one another and interact, no part of the hot side will either be heated up or cooled down; there is no energy gradient for heat to flow from one part of that room to the other. (The cold side has precisely the same properties, except that thermal equilibrium occurs at a much lower temperature.)
But now, what if you remove the divider that separates the hot side of the room from the cold side? What happens?
The answer is that the hot particles and the cold particles will mix, and produce an intermediate-temperature room where all the particles come to the same equilibrium temperature. Before equilibrium is reached, energy can be extracted from the system; afterward, it cannot. When we talk about a state of maximum entropy, we’re talking about a state from which no further energy can be extracted; a maximum entropy system cannot perform work, as we say in physics.
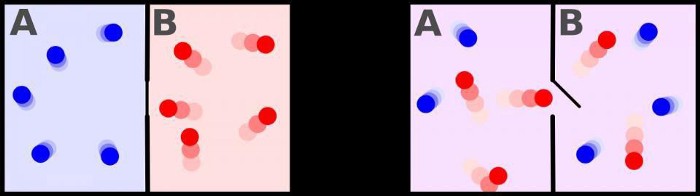
Work is physically real; entropy is physically real; thermodynamics is physically real. Time, as a measurable and observable and quantifiable quantity, is no different than any of those.
However, there are two important caveats to this discussion. While it’s true that time is real, it’s important to keep the following facts in mind.
- We do not know what causes our perceived arrow of time. We always observe time to be flowing forward and not backward; we recognize the passage of time and are subject to the laws of physics moving forward in time, just as all physical objects and quantities are. But whether the entropy of your system remains constant, increases slowly, increases rapidly, or is even artificially decreased by inputting energy into it, the perceived arrow of time never ceases to flow nor reverses direction.
- While time is definitely real, it may or may not be fundamental. In our present way of looking at the Universe, we view something like entropy as a derived quantity and treat time as though it’s fundamental. However, mathematically, it is possible to treat entropy as though it’s a fundamental quantity, and then time behaves as though it can be an emergent quantity. We do not yet know enough about the Universe to comment much about the potential validity of this approach.

Despite the popular trend to question the nature of time, its physical “realness” is not in doubt. Time is an integral part of the Universe, and the boundary between events that have been observed or measured to have a definitive outcome and those whose outcome has not yet been decided is the best way we have to define, precisely, what we mean by the moment of “now”. As esteemed physicist Lee Smolin put it in an exclusive interview with him:
“in the Copenhagen version of quantum mechanics, there is a quantum world and there is a classical world, and a boundary between them: when things become definite. When things that are indefinite in the quantum world become definite. And what they’re trying to say is that is the fundamental thing that happens in nature, when things that are indefinite become definite. And that’s what “now” is. The moment now, the present moment, that all these people say is missing from science and missing from physics, that is the transition from indefinite to definite.”
Time may or may not be fundamental, and our perceived arrow of time may or may not (my hunch is “not”) be related to the thermodynamic arrow of time. But the fact that we can measure, observe, and quantify it should put any doubts of its non-existence to rest.