Should You Play Powerball? Science Solves The Mystery

Last week’s $1.5 billion jackpot was a record that likely will be broken again and again. But when, if ever, should you play?
“I’ve done the calculation and your chances of winning the lottery are identical whether you play or not.” –Fran Lebowitz
Playing the lottery is the ultimate low-risk, high-reward scenario. If you lose, you’re only out a few dollars: the cost of your bet. But if you win, even though the odds are stacked against you, the payoff is a lifetime of easy living. Last week, Wednesday, January 13th, was the richest lottery jackpot in history, with the Powerball jackpot hitting a whopping $1.5 billion, a new record in lottery games worldwide.
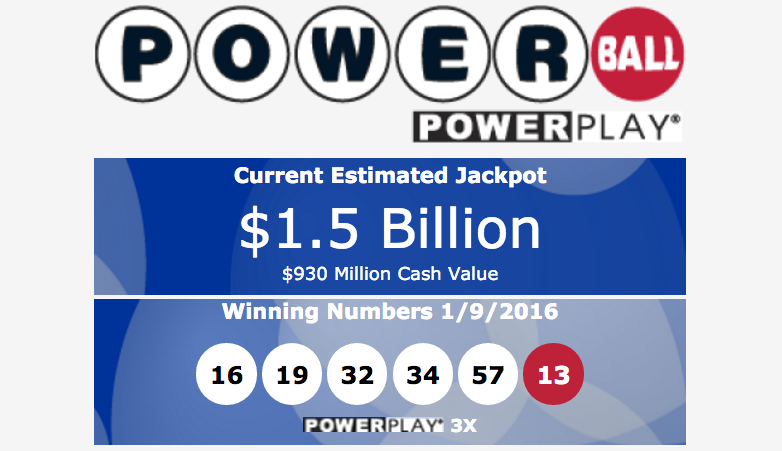
In order to win, you need to match five normal lottery numbers — white balls numbered 1-through-69 — plus the Powerball: a red ball numbered 1-through-26. Each Powerball ticket costs $2, plus you have the option to pay an extra $1 to activate the power play, a multiplier that increases your payout for non-jackpot prizes.

But what does mathematics have to say about whether you should play or not? In particular:
- What are your odds of winning each individual combination?
- How much does each winning possibility pay out?
- Is it worth it to activate the power play option?
- And finally, how big does the jackpot have to be in order for playing the Powerball lottery to be “worth it”?
The idea of “worth it” is a subjective one to most people, but from a scientific/mathematical standpoint, it has a very particular meaning. It means that the amount you can expect to win, on average, is greater than the amount you have to bet in order to play. If a lottery ticket costs $1, for example, it would be worth it if:
- You had a 51% chance of winning $2.
- Or, you had a 0.1% chance of winning $1001.
- Or, you had a 1-in-499,999 chance of winning $500,000.
While it wouldn’t be worth it if:
- You had a 49% chance of winning $2.
- Or, you had a 0.1% chance of winning $999.
- Or, you had a 1-in-500,001 chance of winning $500,000.
Notice how small these differences are, but how in the earlier cases, you can expect to win more than you bet, while in the latter cases, you expect to bet more than you win, at least on average. Mathematicians call this ratio of how-much-you-win vs. how-much-you-bet the expected value of a problem. If your expected value is greater than 1.0, it’s worth it to play.
So what, then, does this mean for Powerball? In every game of Powerball, you get one ticket with five white numbers (out of 69 possible choices) and one red number (the Powerball, out of 26).
Let’s hit the first point we asked about: what are your odds of winning, with each individual combination highlighted? Here’s an infographic I made that breaks it down.
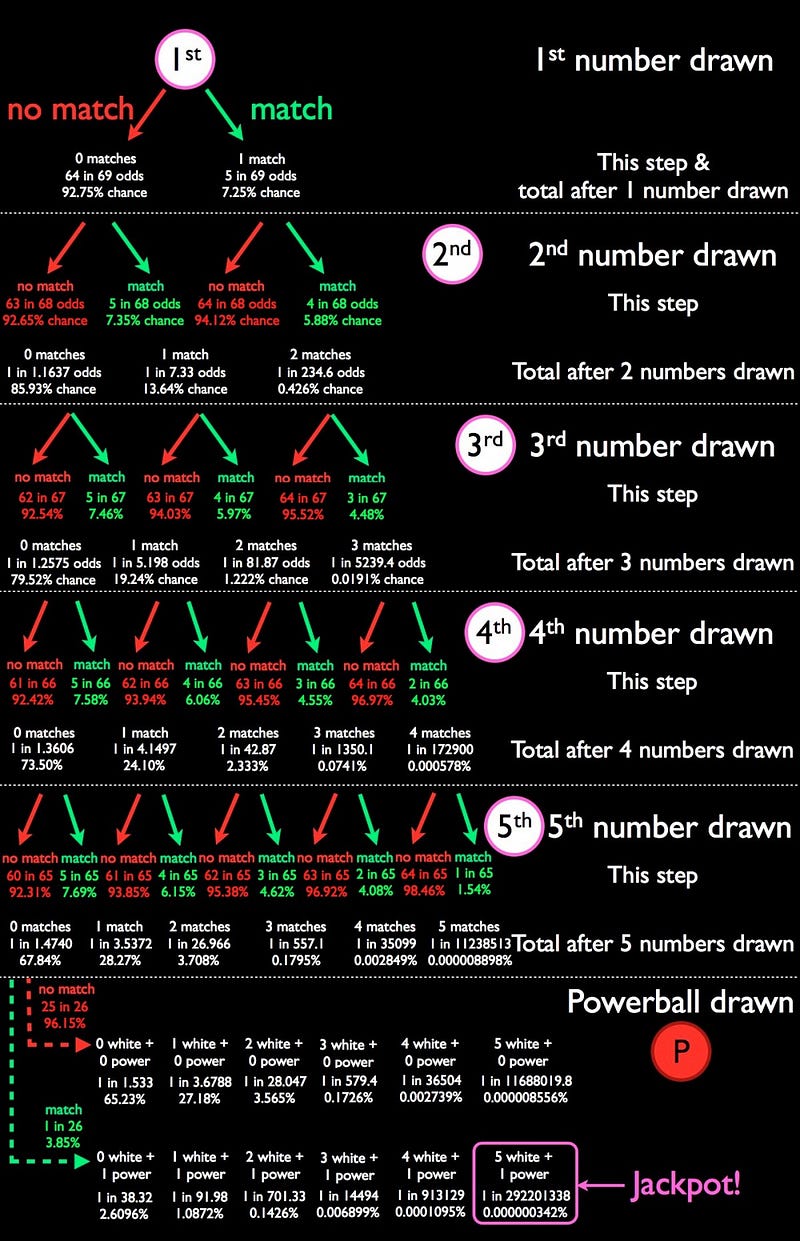
Your odds of actually winning the Powerball jackpot are pretty slim: one in 292,201,338. In fact, your odds of winning anything aren’t very good either, since the three most common results:
- no matches of any type (65.23%),
- one white ball and no powerball (27.18%), and
- two white balls and no powerball (3.565%),
all pay out absolutely nothing, and add up to 95.98% of the possible results.
But that means, 4.02% of the time, you will win something. And if — on average — it pays out enough, it will be worth it to bet.
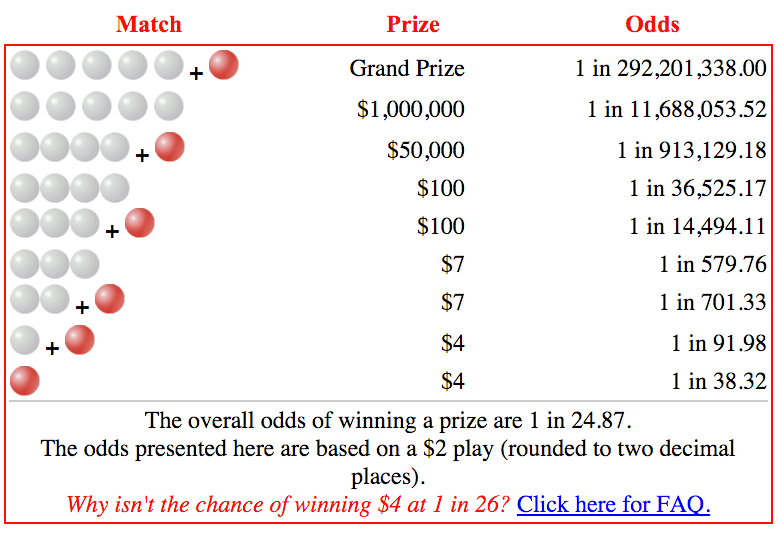
So if these are the possibilities for what the payout is, what does this mean in terms of expected value? In other words, each time you play the lottery, you have a small chance of winning a certain prize, and if you multiply your expected winnings by your odds of each option, you get your expected value for each option. (And remember, the cost of a ticket for the Powerball is $2.00.)
Let’s work it out! We’ll come back to the “Jackpot” in just a bit, because it’s a bit more complicated. But let’s take a look at all the other — more likely, but non-Jackpot — options first.
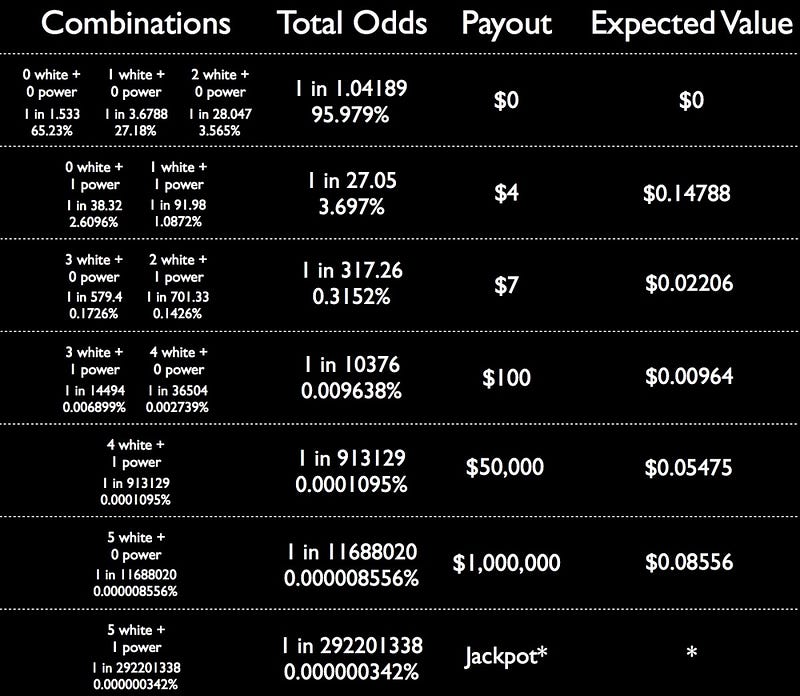
For every $2 ticket you buy, you can expect to recoup, on average:
- about $0.15 from the periodic $4 payouts,
- about $0.02 from the periodic $7 payouts,
- about $0.01 from the periodic $100 payouts,
- about $0.05 from the periodic $50,000 payouts, and
- about $0.09 from the periodic $1,000,000 payouts.
All told, the non-jackpot options make each ticket worth about $0.32, which is a far cry from the $2 you invested. This teaches us two things:
- It gives us the information we need to figure out how much the “Power Play” option is actually worth.
- It let’s us know how much the Jackpot needs to pay out in order for buying a Powerball ticket to be “worth it,” mathematically.
First, the Power Play.
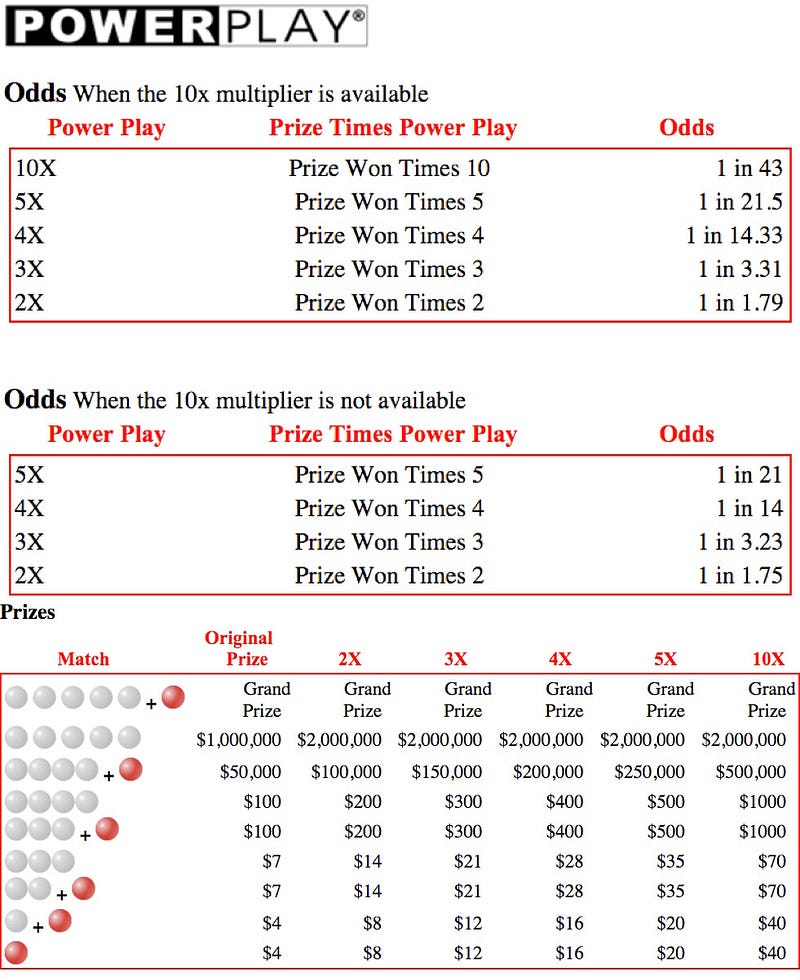
The Power Play option — which costs an extra $1.00, turning a $2 ticket into a $3 ticket — does the following:
- has no effect on the Jackpot/Grand Prize,
- always doubles the payout of the second-most-lucrative prize, and
- has a 1-in-1.75 chance of doubling the other prizes, a 1-in-3.23 chance of tripling them, and a 1-in-14 chance of quadrupling or a 1-in-21 chance of quintupling them.
- or, if the 10x multiplier is active, reducing the chances of all the other options a tiny bit, and adding in a 1-in-43 chance of multiplying the other prizes by ten.
So what’s the extra expected payoff for this extra $1 investment?
It takes the non-jackpot options, on average, from being worth $0.32 up to being worth $0.81. This means you’re spending an extra $1.00 to increase your expected payout by $0.49, a lousy deal any way you slice it. In fact, even if you happened to hit the 5x option, which happens only about 5% of the time, you only up your expected winnings to $1.34 for the non-jackpot options, which increases your winnings by a mere $1.02. That’s what you need to make it “worth” grabbing the Power Play option: a guaranteed 5x multiplier or more. The fact that the second-biggest-payout is only doubled, no matter what the Power Play multiplier happens to be, makes this a raw deal any way you slice it.
In other words, you should never take the Power Play option.
So finally, we come to the big prize: the Jackpot, or the Grand Prize, which you win by hitting all five numbers plus the Powerball, something that has a one-in-292,201,338 chance of happening.

You would think, perhaps, that if a Powerball ticket costs you $2, and you have an expected value of $0.32 from the rest of the ticket, as long as your expected value is $1.68 or higher from the Powerball Grand Prize, you’ll come out ahead, and you should play.
That logic is sound, by the way: you’re right on! If your ticket is “worth” more than $2 total, of course you should spend $2 on it.
But you might then take the next step, and say, “since my odds of winning the Jackpot are 1-in-292,201,338, all I need to do is find what payout corresponds to that expected value and, if the Jackpot is more than that, I’ll play.” Finding that value isn’t so hard: it’s $245,449,123.92. But if you play the lottery when the Jackpot is that size, you’ll come out behind, still, for two reasons.

One of those reasons is taxes. That’s right: you don’t get to keep 100% of your winnings, although you are responsible for 100% of the costs of the tickets. The “advertised jackpot” is how much you’d get, pre-taxes, if you deferred the payments out over a long period of time.
If, instead, you took a lump sum payment (which is drastically reduced to about 63% of the advertised value), and then paid (state and federal) taxes on that, you’d discover something shocking: you only get to keep 37.2% of the Grand Prize’s value! (Dependent on your state’s taxes, which are estimated to be around 6%.) In fact, state and federal taxes would be a big deal for theother, smaller prizes we talked about earlier, which would bump the $1,000,000 prize down to be worth only about $590,000, and would reduce the value of the “other” payouts from being worth a total of $0.36 to about $0.26, a much worse deal.
In order to hit the “break-even” point, and have a $2 ticket actually be worth $2 when you include taxes, you’d need to have the Jackpot come in at a value of a whopping $1.4 billion, which only today’s Powerball Jackpot has crested in all of history.
But even that makes an assumption: that if you win, that your winning ticket will be the only winning ticket, which runs counter to the facts. As you well know, people are far more likely to buy lottery tickets when the Jackpot is huge. While you might not think this would mean so much with incredibly long odds, once you start selling more than about 70 million tickets, the odds of more than one person hitting the jackpot rise extremely quickly, as Jeremy Elson’s research into the matter has demonstrated.
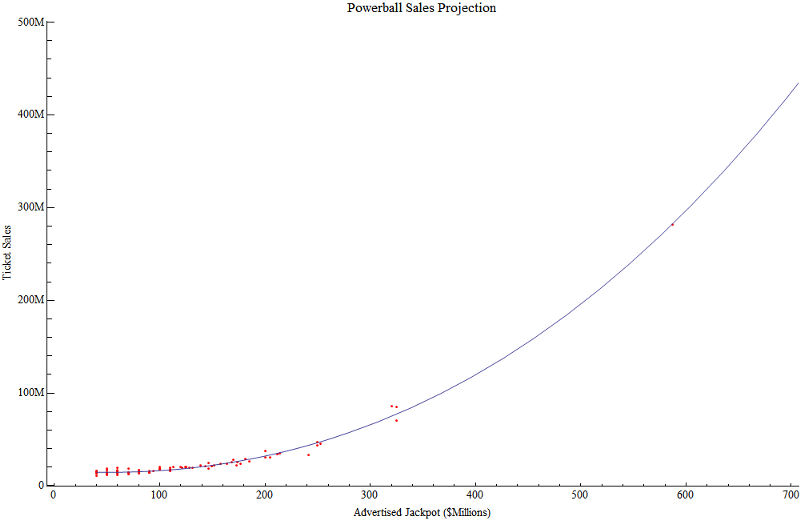
Based on various Jackpots that we’ve seen, once the advertised Jackpot passes about $300 million, ticket sales rise exponentially, with the largest jackpots resulting in hundreds of millions of tickets sold.
The thing is, not everyone gets a unique set of numbers: many tickets share the same numbers, so that if you get to, say, an advertised Jackpot of around $700 million, you’d expect to sell about 190 million tickets for the drawing. Even though the odds of any individual ticket hitting the Jackpot is 1-in-292,201,338, the odds that only one person will win that Jackpot is much lower than you’d think: about 37%. There’d be a 34% chance that no one would hit the Jackpot, and a 29% chance that two or more people would win.
What’s crazy — and unintuitive — is that as the Jackpot rises higher and higher, because more and more tickets get sold, the less valuable each ticket becomes! A ticket sold for a $1,500 million (or $1.5 billion) Jackpot, in fact, would only be worth about half as much as a ticket sold for a $500 million Jackpot, because you’d most likely have to split the Jackpot, even if you won, with between three and seven other people. And that’s probably what’s going to happen tonight.
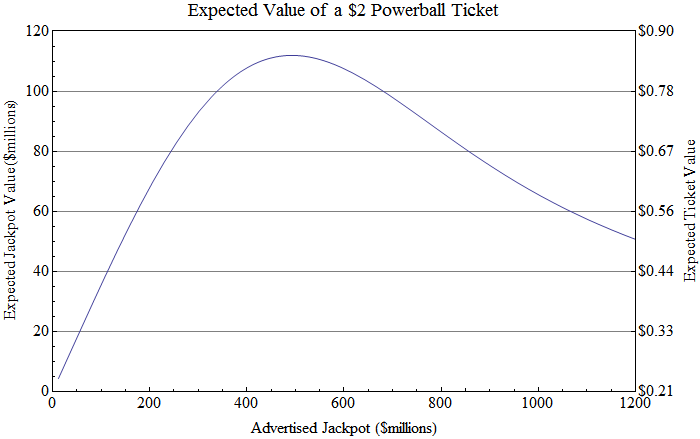
When you take both taxes and split Jackpots into account, you find that even at its maximum value, a $2 Powerball ticket is really only worth about $0.852, or just 43% of what you paid for it.
It’s long been said that lottery tickets are a tax on those who can’t do math, and now you’ve seen the mathematical proof of that. If you want to buy a Powerball ticket for fun, by all means, go right ahead. Just be aware that for every $2 you spend, you’re donating about $1.15 to whatever government programs that Powerball supports, and only betting $0.85 on whatever you might win in an otherwise “fair” lottery.
Leave your comments on our forum, and check out our first book: Beyond The Galaxy, available now, as well as our reward-rich Patreon campaign!