Weekend Diversion: Zooming Into A Fractal
Just open your eyes, full-screen it, and watch.
https://www.youtube.com/watch?v=PD2XgQOyCCk
“Exploring this set I certainly never had the feeling of invention. I never had the feeling that my imagination was rich enough to invent all those extraordinary things on discovering them. They were there, even though nobody had seen them before. It’s marvelous, a very simple formula explains all these very complicated things. So the goal of science is starting with a mess, and explaining it with a simple formula, a kind of dream of science.” -Benoit Mandelbrot
Sometimes, words don’t quite do justice to what a picture can illustrate. Have a listen to a great soundtrack for the following visuals in Yo La Tengo’s song, Night Falls On Hoboken,
while you consider the Mandelbrot set, and what a fractal is.
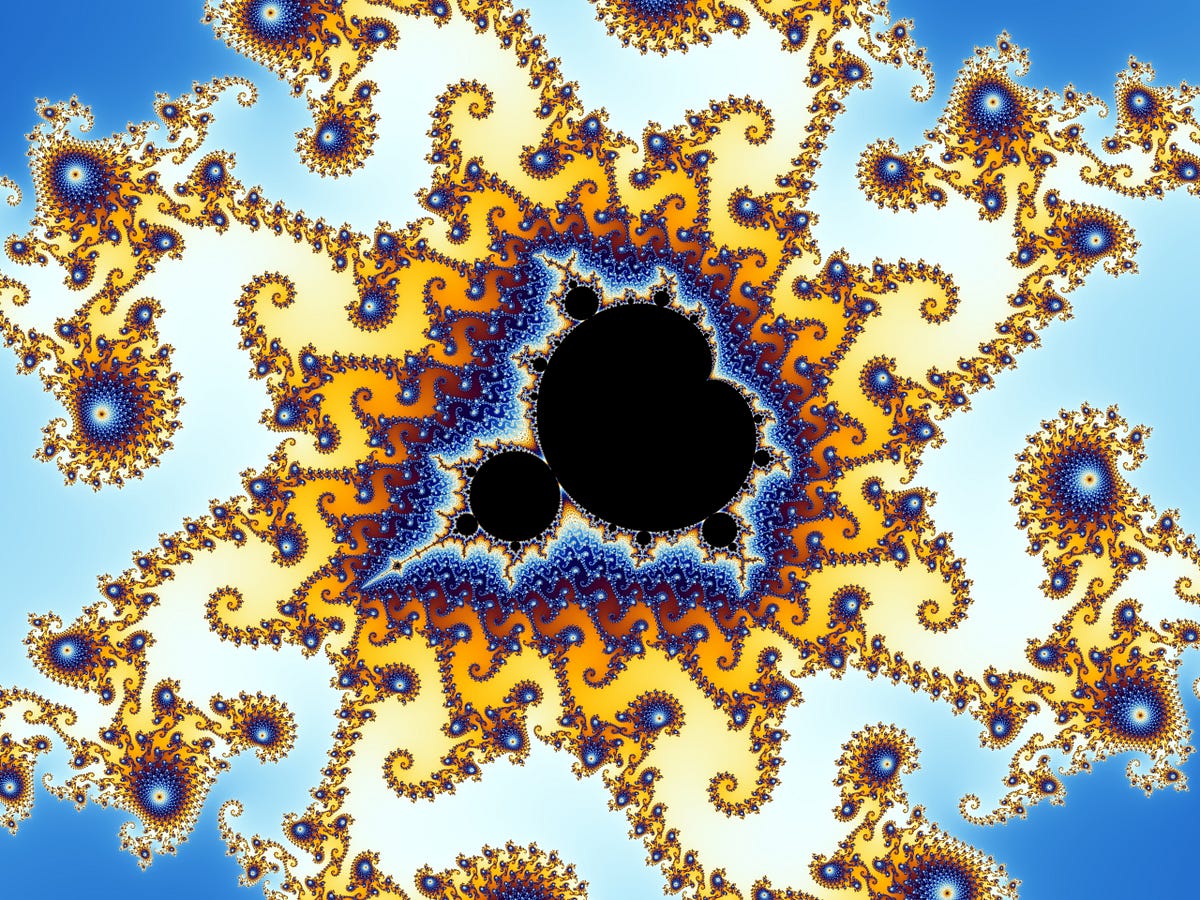
You’re used to real numbers: that is, numbers that can be expressed as a decimal, even if it’s an arbitrarily long, non-repeating decimal. There are also complex numbers, which are numbers that have a real part and also an imaginary part. The imaginary part is just like the real part, but is also multiplied by i, or the square root of -1.
And the Mandelbrot set consists of every possible complex number, n, where the sequence n, n^2 + n, (n^2 + n)^2 + n, etc. — where each new term is the prior term, squared, plus n — does not go to either positive or negative infinity.
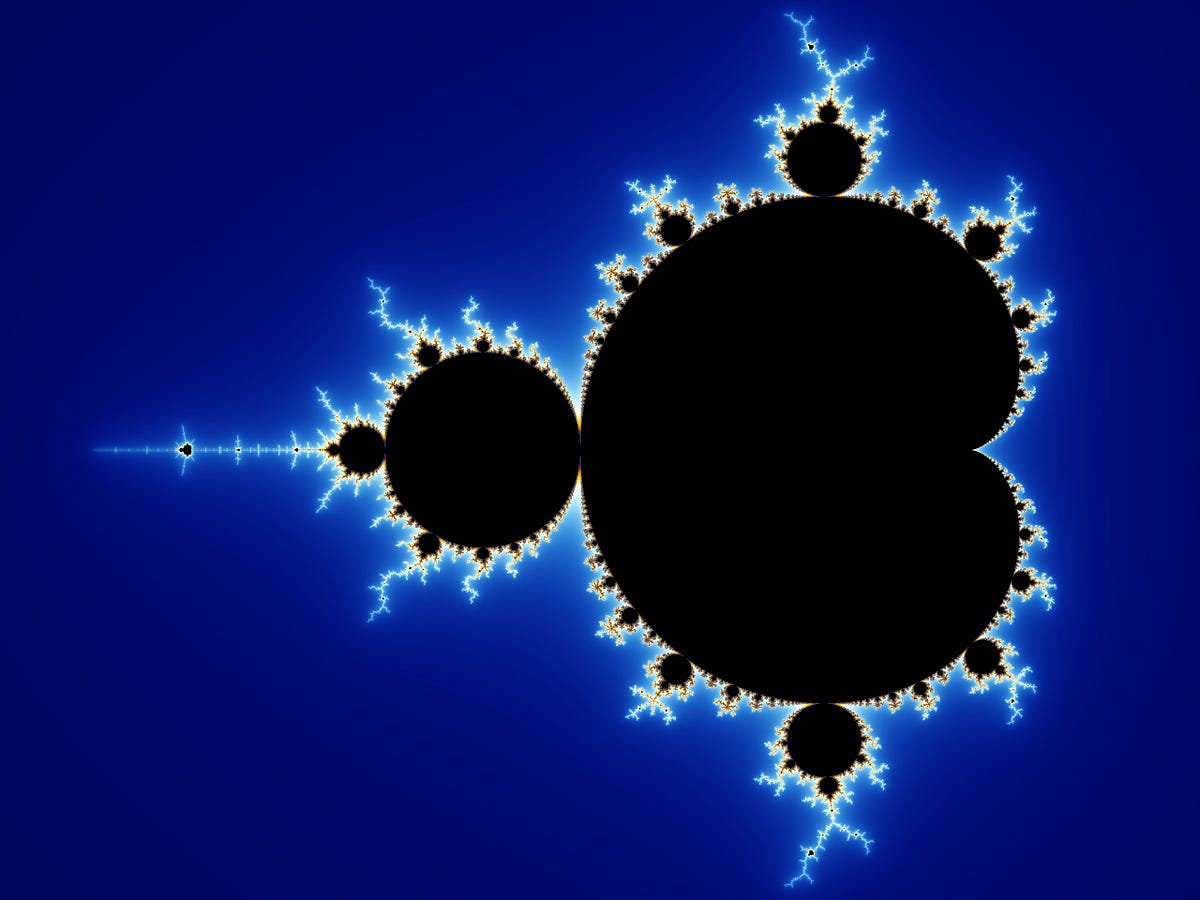
Mathematically, it has some amazingly interesting properties. Even though the boundary of the set makes a very complicated line through the complex plane, that line has not only an infinite length, it encloses a finite and quantifiable area, that comes in at just a little bit over one-and-a-half.
What we visualize as these intricate patterns by zooming in actually represents the border between what’s actually in the Mandelbrot set vs. what’s outside of it, with color coding normally representing how far away something is from being outside of the set.

What’s remarkable is how intricate and self-repeating this set is, and how zooming in allows you to see small regions that have — to the best of our knowledge — identical properties to the entire set itself. We call this property self-similarity, meaning that a small region has the same or nearly the same properties as either a larger region or the whole thing.
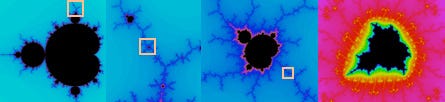
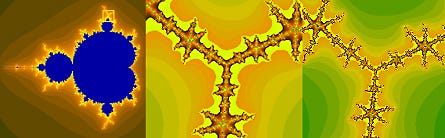
Unlike simple cases, however, the complexity of a fractal is what sets it apart: there is arbitrarily detailed structure no matter how fine a scale you zoom in on.
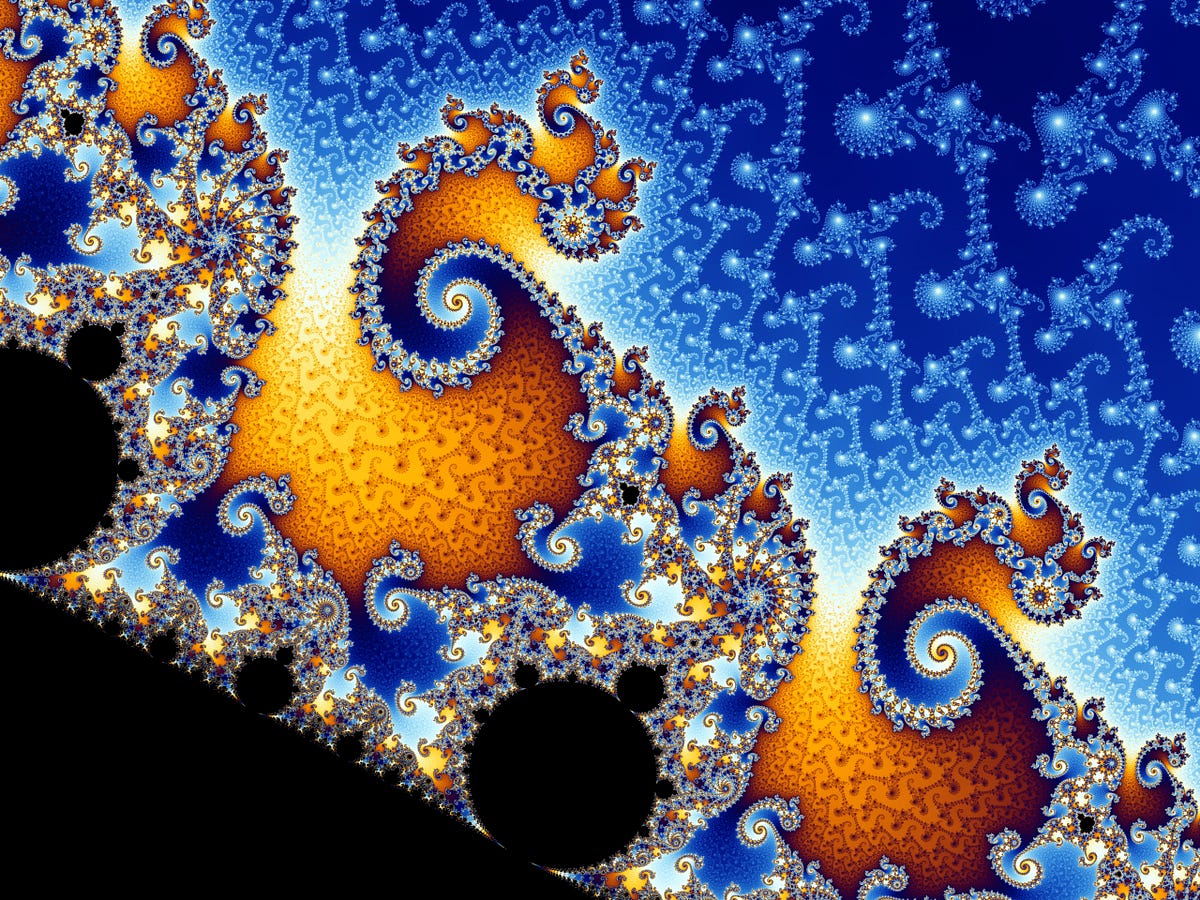
What’s most amazing? We’ve managed to zoom in by more than a factor of 10^200, or more than a googol squared, and we still find this same self-similarity, and the same remarkable, intricate structures. There are ideas that perhaps the Universe is self-similar like this, but if it is, there’s a finite limit: the largest observable scales are “only” 92 billion light years or so (from one edge of the observable Universe to the other), while the smallest theoretical scale, the Planck scale, is down at around 10^-35 meters. All told, this is just 62 orders of magnitude, which doesn’t even account for the fact that non-gravitational forces begin to play important roles on scales the size of galaxies and smaller.
Nevertheless, mathematics is not bound by the physical laws of our Universe, which allows us some incredible visualizations with different color-identification schemes. Here are a few of my favorites.
For those wondering, Mandelbrot — the most important developer of fractal geometry — lived to the age of 85, dying only in 2010, meaning he lived to witness the advances in computational technology that enabled these stunning visualizations that his mathematical work not only anticipated, but demanded.
And with these videos to cap it all off, I hope you have a great weekend, or whenever it is you get around to watching these. Enjoy!
Leave your comments at the Starts With A Bang forum on Scienceblogs!