Ask Ethan: Why Can’t Time Be Reversed For Three-Body Systems?

In principle, the laws of physics are the same forwards and backwards. But in practice, time only runs in one direction.
Most of the laws of physics are the same whether you run the clock forwards or backwards. A planet that revolves around a star appears to obey the same rules as it would if you recorded it and played the recording backwards. This is true for any gravitational, electromagnetic, or strong nuclear interaction between two particles: they are time-reversal invariant. Normally, we only see the non-reversible effects of chaos and thermodynamics with extremely large systems, but a recent paper claims to have shown this for the gravitational interaction with only three masses. Jonathan Belew wants to know what this means, asking:
Does this have any relevancy to the n-body problem, time-reversal symmetry as a whole, or implications for cosmology? Or is it theoretical and only apply to a small subset of cases that do not make up a significant portion of the observed universe?
Let’s unpack what it all means.

It’s well-known that nearly all interactions in physics obey what we call time-reversal invariance. This means that the laws of physics behave the same way forwards or backwards. You can view this in a number of ways, such as:
- running your clock forwards or backwards in time,
- running all of your system’s particles from the beginning with their initial momenta or from the end with the opposite of their final momenta,
- or starting from your final state and asking if there’s always a way to evolve that final state back into your initial state.
For any one or two particles experiencing any physical force or interaction, with the exception of the weak nuclear interaction (which is known to violate time-reversal symmetry), there’s always a way to recover your initial state if you begin with your final state and evolve it according to the known laws of physics.

However, the macroscopic world doesn’t appear to be time-reversal invariant at all. Sure, if you watch a ball flying through the air, up or down a hill, Earth spinning on its axis, or a moon orbiting a planet, you can’t tell just from looking at it whether the clock is running forwards or backwards. Because the laws of physics are the same forwards and backwards in time — because of this time-reversal invariance — the same rules are obeyed.
But other phenomena clearly have a direction: an arrow of time. Drop a glass of water onto a hard floor and watch it shatter; the time-reversed reaction will never, ever occur, not even if you perform the experiment quadrillions of times. Scramble and cook and egg; an egg will never “uncook” and “unscramble” itself. These are examples where there’s clearly a preferred direction to the Universe, something we refer to as the thermodynamic arrow of time.
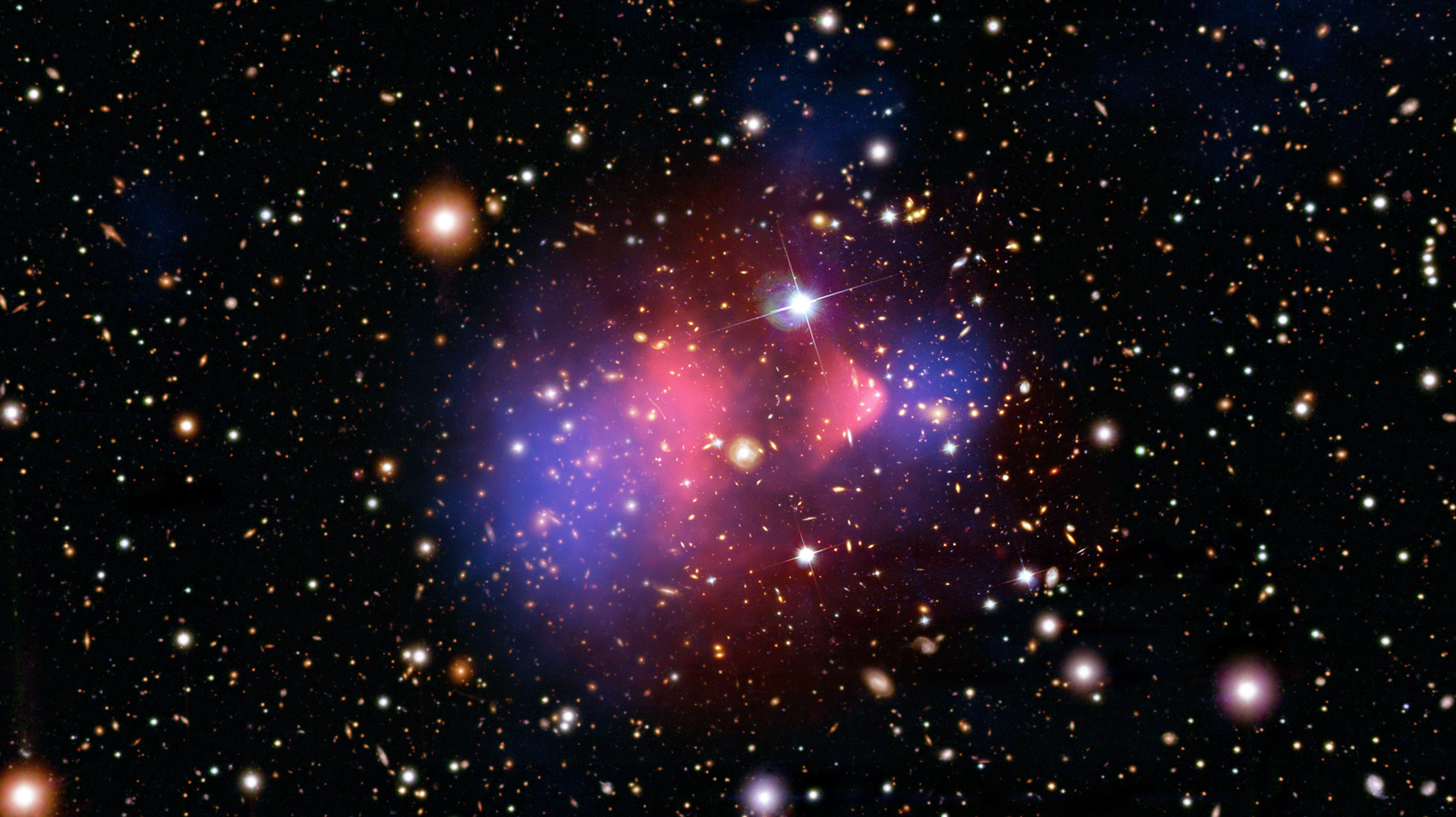
Even though, in both of these cases, only gravitational and electromagnetic interactions are at play, the interactions become so complex and occur between so many particles — chaotically, on top of classically — that the final state you wind up with is extraordinarily unlikely to ever return to the initial state, no matter how intricately you reverse the reaction.
It’s like taking a room with a divider down the middle, where one side is hot and the other is cold, removing the divider, and watching the gas molecules fly around. In the absence of any other inputs, the two halves of the room will mix and equilibrate, reaching the same temperature. No matter what you did to those particles, including reverse all of their momenta, they’d never reach the half-hot and half-cold state ever again.
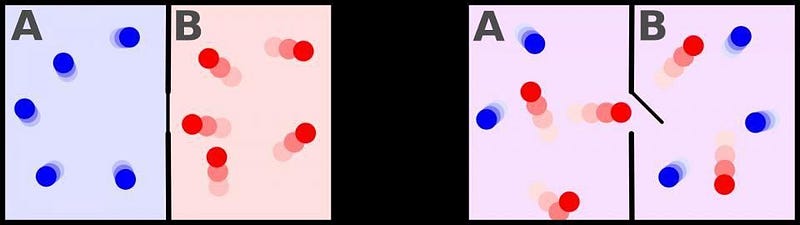
This type of irreversibility is well understood for large, many particle systems, and is an essential part of the sciences of thermodynamics and statistical mechanics. It’s part of why we use the quantity of entropy so frequently, and our understanding of these processes helps us understand the second law of thermodynamics: that in a closed system, entropy only increases or remains the same, never decreasing.
But it was only understood in a statistical sense. Only in systems with large numbers of particles all interacting with one another does this type of chaos typically manifest, driving these dual phenomena of time irreversibility and entropy increases. Of course, the same rules that govern many-particle systems have to govern few-particle systems as well, so there should be examples of chaos, irreversibility, and entropy increases in systems without many particles at all.

In the April 2020 issue of the Monthly Notices of the Royal Astronomical Society, a new paper was published with the title, Gargantuan chaotic gravitational three-body systems and their irreversibility to the Planck length. (Preprint available in full here.) Previous research has shown that chaos in as inherent property of many real-life astrophysical systems, including:
- for small, low-mass objects in the Solar System,
- systems with only small numbers of stars,
- individual star clusters,
- and galaxies that evolve with time.
If you have a small, tiny change in the initial conditions of your system — where just one object is in a slightly different location or has a slightly different velocity — you’ll get a completely different result down the road.
If you want to understand entropy increase, what you have to look at is the increase in the number of possible outcomes when starting from initial conditions that are only very slightly different from one another. Sometimes, if you vary the initial conditions only slightly, you’ll wind up with the same end state: that’s an example of a converging solution, where entropy doesn’t significantly increase.
But other times, you’ll wind up with very different end states: end states that appear to bear very little relation to what you started off with initially. Those are diverging solutions, and that’s where entropy increase comes from. Although physical systems with large numbers of particles can arrive at this, it’s important to connect them, physically, to the initial conditions that you start off with. This is harder to do for systems with larger numbers of particles, and has been a controversial area of study for the past few decades.

Recently, however, advances in computing power and brute-force algorithms have enabled certain very simple problems to be solved numerically, and to determine things like:
- which problems-and-conditions converge and which ones diverge,
- where everything can be calculated to arbitrary precision (at the expense of computing time),
- and where, if the solution is time-reversible, you can start at the end state and can retrieve the initial conditions to many digits of precision for every body in the system.
What the new paper by Boekholt, Portegies Zwart, and Valtonen did was to analyze a system of three equal mass, non-rotating black holes (i.e., point masses) that start off at rest but with arbitrary positions. Some solutions to this setup were previously known to be reversible, while others were thought to be irreversible.
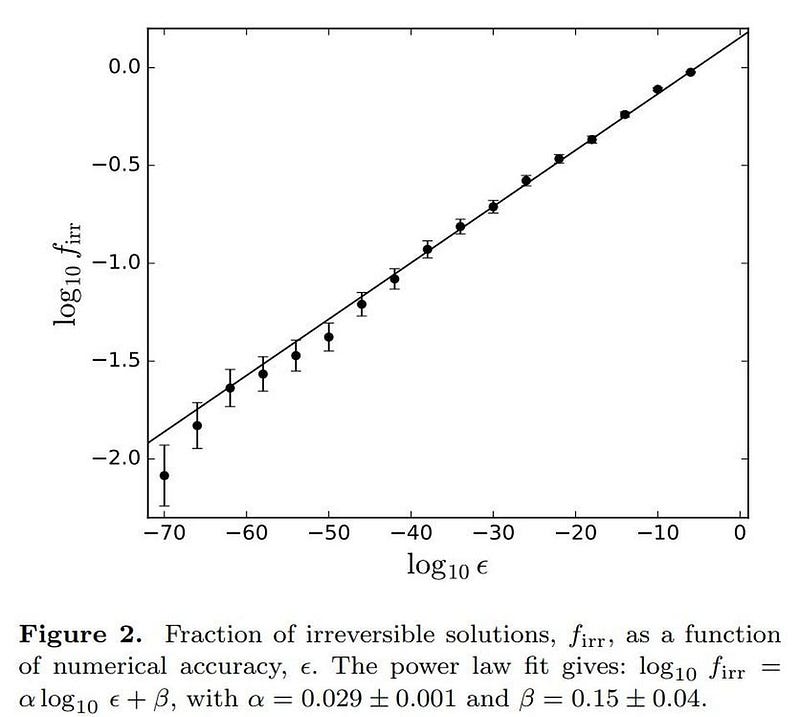
This new work really does take our understanding to the next level. As you increase the precision of your calculation, taking smaller and smaller steps and increasing your numerical accuracy, more and more of the solutions that appeared irreversible actually turned out to be reversible. The more precisely (i.e., the more significant digits) you calculated the distance between any two objects to be, the better time-reversibility began to look.
But there’s a limit to this: the limit set by the quantum rules that govern our Universe. You can’t compute distances to arbitrary accuracy in our physical reality because below a certain distance scale — the Planck scale, or around 10^-35 meters — the laws of physics break down. By considering black holes with masses of ~1 million solar masses and initial separations on the order of ~1 light-year, they find that about 5% of all configurations are fundamentally irreversible.
It’s a very clever result to learn that, for the realistically large-mass objects we have in our Universe, the precision required to calculate a truly rime-reversible solution is greater than the precision the physical Universe actually allows. If the laws of quantum physics and General Relativity are both correct, as we have every reason to believe that they are, then even purely gravitational systems with as few as three masses are fundamentally irreversible.
Of course, plenty of other reactions are known to be irreversible as well: two orbiting black holes emit gravitational radiation and inspiral, but no orbiting black holes absorb gravitational radiation and outspiral, for example. But for the first time, scientists have demonstrated — assuming the laws of physics are what we think they are — that a purely classical system with only three masses isn’t always time-reversible. The Universe really is unpredictable and chaotic at a fundamental level.
Send in your Ask Ethan questions to startswithabang at gmail dot com!
Ethan Siegel is the author of Beyond the Galaxy and Treknology. You can pre-order his third book, currently in development: the Encyclopaedia Cosmologica.