How Big is the Entire Universe?

To the limits of our observable Universe and well beyond, here’s what we know the minimum size of the Universe must be, along with how we know it.

“The greatest enemy of knowledge is not ignorance, it is the illusion of knowledge.” –Stephen Hawking

The Universe is a vast, seemingly unending marvel of existence. Over the past century, we’ve learned that the Universe stretches out beyond the billions of stars in our Milky Way, out across tens of billions of light years, containing close to a trillion galaxies all told.

And yet, that’s just the observable Universe! There are good reasons to believe that the Universe continues on and on beyond the limits of what we can see; the question is, how far does it go on? Forever? Or does it close back upon itself at some point?

To help us better understand this question, let’s turn to something more familiar (and smaller) that we know how to measure the size of: the Earth.

From the top of a tall mountain, like Mauna Kea, shown here, you might hope to measure the Earth’s curvature, but your efforts would be in vain. From even 14,000 feet up, the curvature of the Earth is totally indistinguishable from flat.

There are images out there where the Earth appears curved when you look out at the water, and indeed, they’re not hard to find. But is that because of the Earth’s curvature?

Not at all; it’s because of atmospheric distortion. If you were to try and calculate the circumference of the Earth from a photo like this, you’d get a world that was smaller than even the Moon is; you cannot measure the curvature of the Earth from any known location on the surface of the planet.

Moreover, when we’re talking about the land surface, the Earth itself isn’t perfectly smooth. Some places are curved upwards, others downwards, and any small region visible to you is unlikely to be a fair representation of the entire planet.

There is a way that you’d be able to tell, though, what the shape and size of the planet actually is. All you’d have to do is take the appropriate measurements and use geometry.

It’s as simple as going to three separate locations on Earth and drawing a triangle to connect those three points.

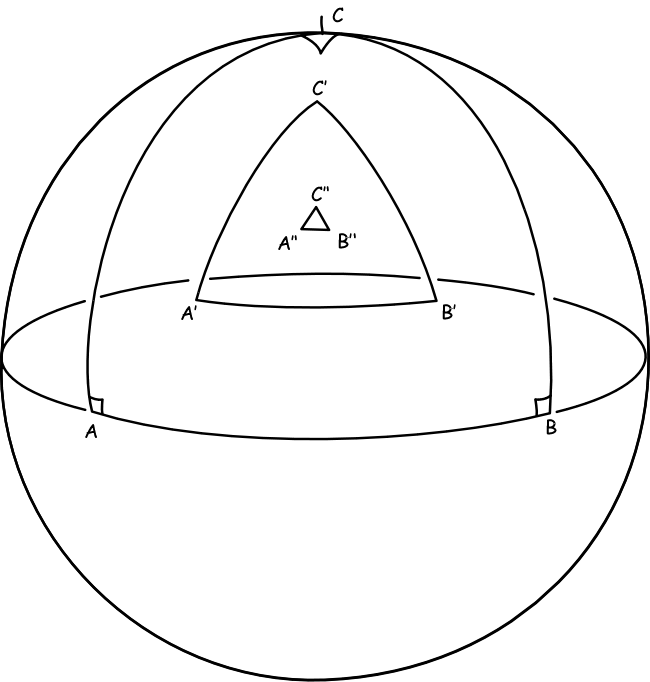
On a flat sheet of paper, the three angles of any triangle will always add up to 180°, as you well know. But if you’re on the surface of a sphere (or, mathematically, any surface of positive curvature), those angles will add up to more than 180°. Knowing the distance between each of those three points and the measure of all three angles allows you to calculate what the circumference of the Earth is.

And, of course, the farther away your three points are from one another, the less important the mountains, valleys and oceans are, and the more important the overall shape of the Earth is to your measurement. The converse would have been true if the Earth were shaped with negative curvature, like a saddle, as shown below.

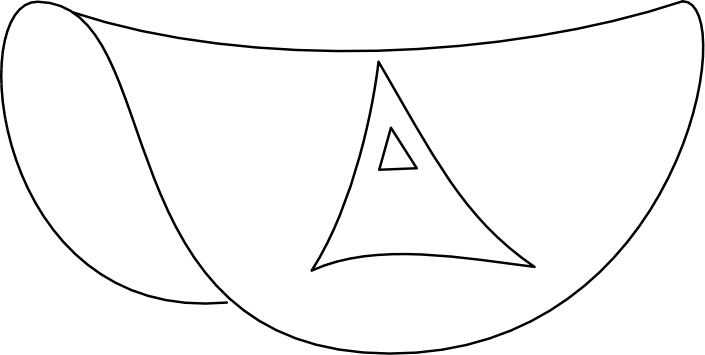
A surface of negative curvature has any three points form a triangle whose three angles sum to less than 180°, and again, knowing the distances and measurements of all three angles allows you to calculate the radius of curvature.

In practice, the very first calculation of the circumference of the Earth — dating to the 3rd Century B.C. — used a very similar method, again reliant on simple geometry.

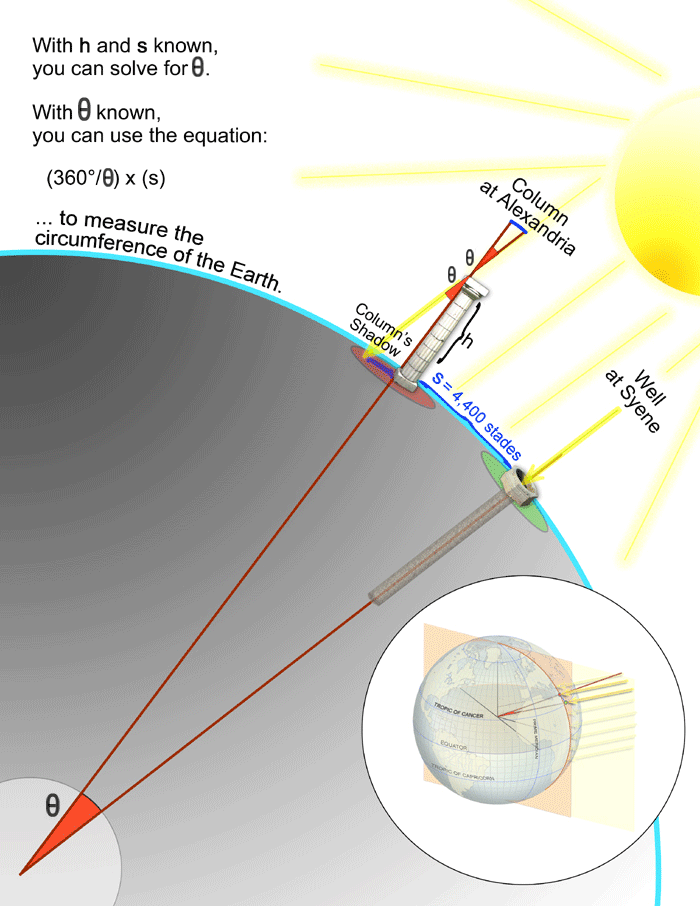
It would not be until the 20th Century that we were actually able to achieve altitudes capable of measuring the curvature of the Earth from space, something we are only able to do because we can step off of the two-dimensional surface of the Earth and look at it from afar.

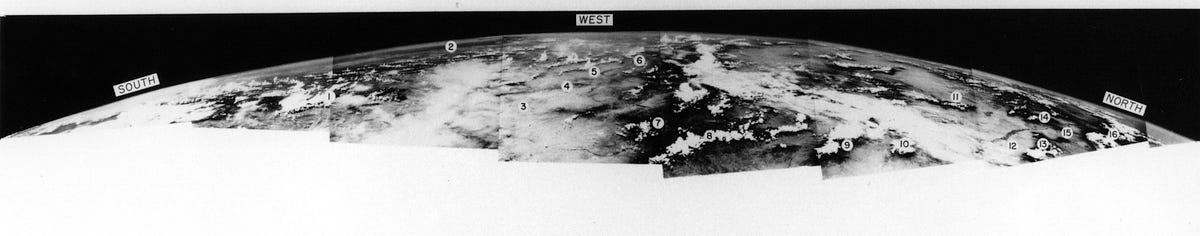
By 1948, we were creating mosaics of the Earth by stitching together multiple images of the Earth from space, and there could no longer be any doubt as to its circumference.

But space itself is a little trickier. Yes, it is just a geometric construct (albeit a slightly more complicated one), but it comes along with an inherent curvature to it. According to the rules of Einstein’s General Relativity, the amount that the space of our Universe is curved is directly related to the amount of matter and energy that we have in it, as well as its global expansion.

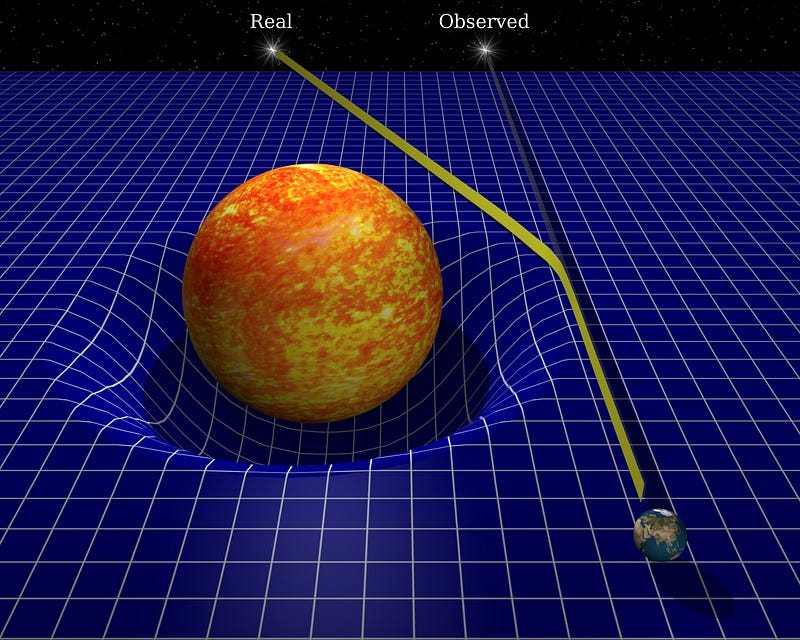
Dense, heavy masses like the Sun cause very large amounts of curvature in very small spaces, significant enough to bend starlight by amounts significant enough that you could notice it with 1919′s technology. But that’s local curvature, the same way mountains, valleys and ocean waves are local curvature here on Earth. What we’re interested in is whether the entire Universe ever closes back in on itself, and if so, how big it is. In other words, these local sources of curvature are things we need to not be fooled by.

The Earth, too, curves the spacetime around it. Remember that we use two dimensions as an illustration, but unlike measuring the curvature of the two-dimensional surface of the Earth, where we can fly “up” and observe the planet below, there is no extra spatial dimension to move through to step back from the curvature of our three-dimensional space.

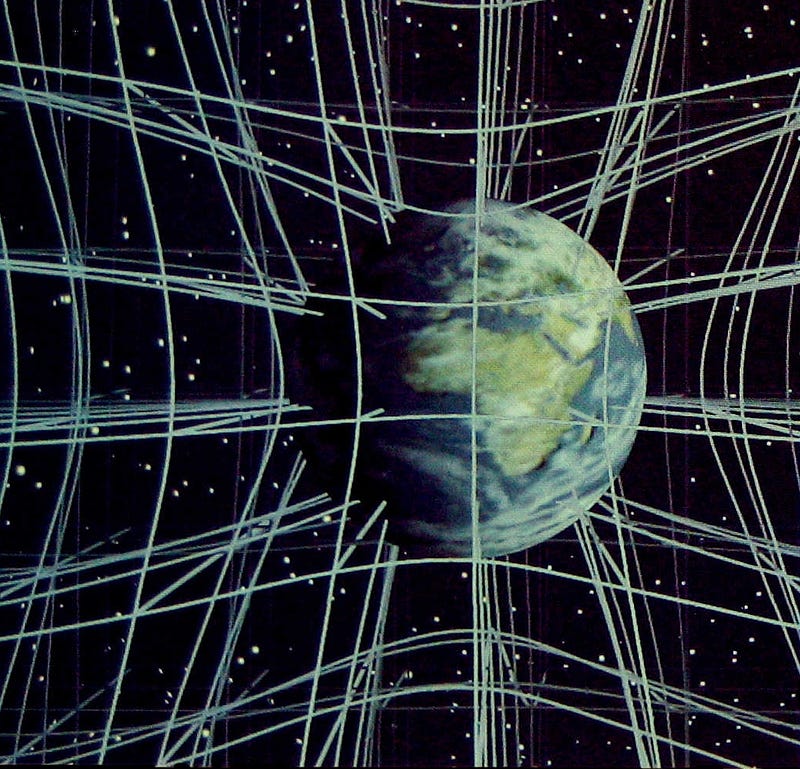
And all of the spatial dimensions are curved. Since stepping back from the Universe and observing it from afar isn’t an option, the only way to get a good handle on its curvature is to examine it on its largest scales — the same way we did it before spaceflight on Earth — and try to infer its geometry.

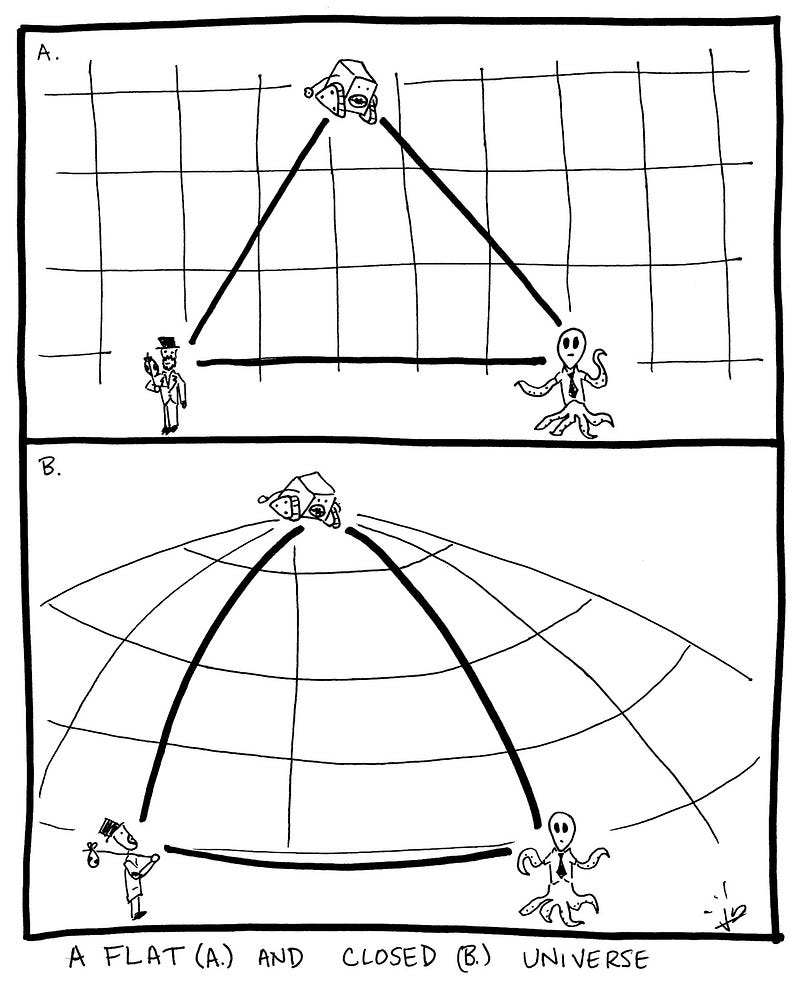
In principle, this is pretty straightforward. Just as any three points on a surface can help you calculate that surface’s curvature, you can do the exact same thing with the Universe! Take any three points that are far enough apart, measure the distances between those points and the relative angles between them as well, and you’ll be able to figure out not only how your space is curved, but also what the radius of curvature is!

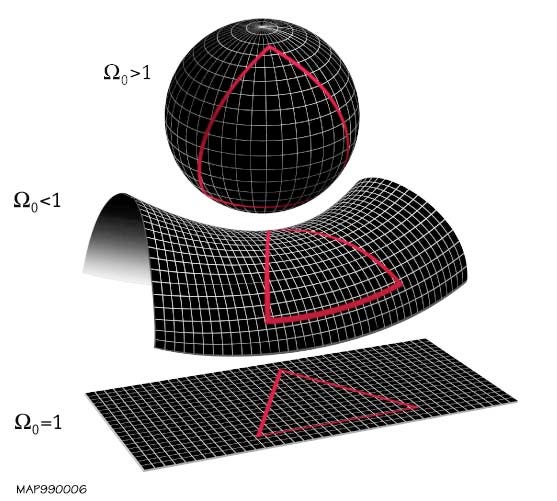
You can imagine three possible cases, of course. One is where the Universe is positively curved, like a higher-dimensional sphere, one is where the Universe is totally flat, like a higher-dimensional grid, and one where the Universe is negatively curved, like a higher-dimensional saddle. In the context of general relativity, it’s the energy density — the amount of matter and all other forms of energy — that determine this curvature.

In real life, we don’t have man-made objects far enough away to communicate with us across the necessary distances to measure curvature. Even if we did, it would take billions of years to do it, which is a disheartening way to attempt to do science. But we have light signals from when the Universe was just 380,000 years old, that tell us what the Universe is like 46 billion light years away.

The fluctuations in the cosmic microwave background — the leftover glow from the big bang — provide a window allowing us to see how our Universe is curved.

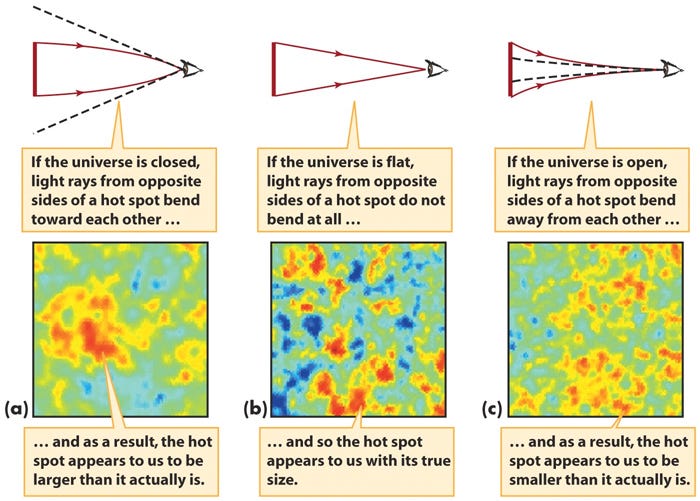
The first robust measurements of this came from the BOOMERanG experiment in the late 1990s (hearing Paolo de Bernardis talk about this in 2004 was a highlight for me during the early stages of my scientific career), where they first determined that rather than having significant positive or negative curvature, the Universe was indistinguishable from flat.

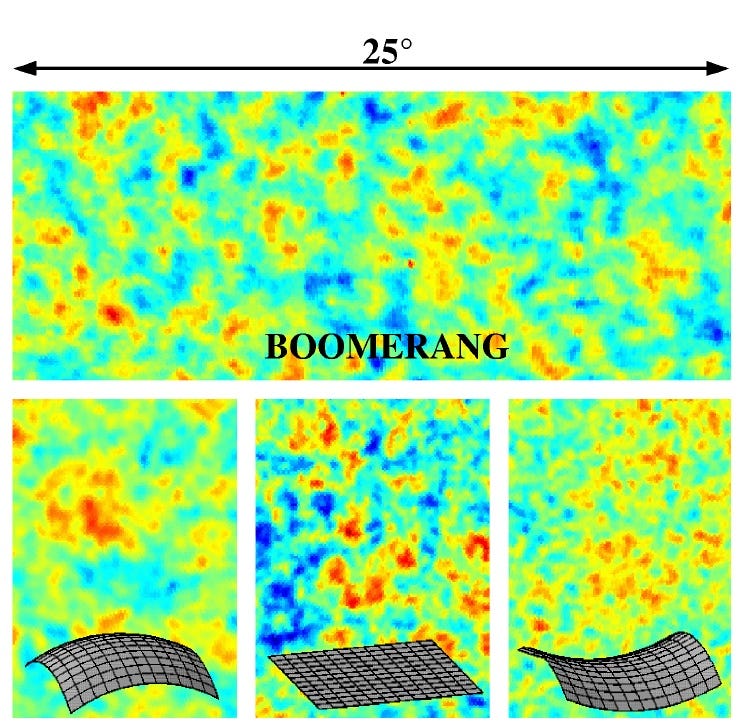
That doesn’t mean that it is flat, of course. If you walked outside and tried to measure the curvature of the Earth right now, but only within 5 km (or 3 miles) of your current location, you would find that the Earth is consistent with being flat, but it could also be positively or negatively curved on a larger scale than you’re currently measuring.

So it goes with the Universe as well. We were able to measure that the Universe, if it is curved, has a much larger radius of curvature than that of our observable Universe, which is about 46 billion light years. But if we could make that measurement more precise, we could conceivably measure a much smaller curvature than even that. Thanks to the Planck satellite, we now have the temperature fluctuations over the entire sky measured at a very narrow, tenth-of-a-degree resolution.

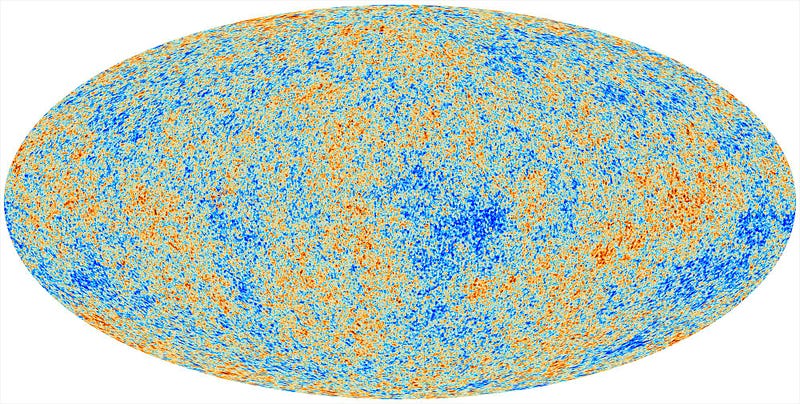
And what they teach us is that not only is the Universe consistent with being flat, it’s really, really, REALLY flat! If the Universe does curve back and close on itself, its radius of curvature is at least 150 times as large as the part that’s observable to us! Meaning that — even without speculative physics like cosmic inflation and multiverses — we know that the entire Universe extends for at least 14 trillion light years in diameter, including the part that’s unobservable to us today.

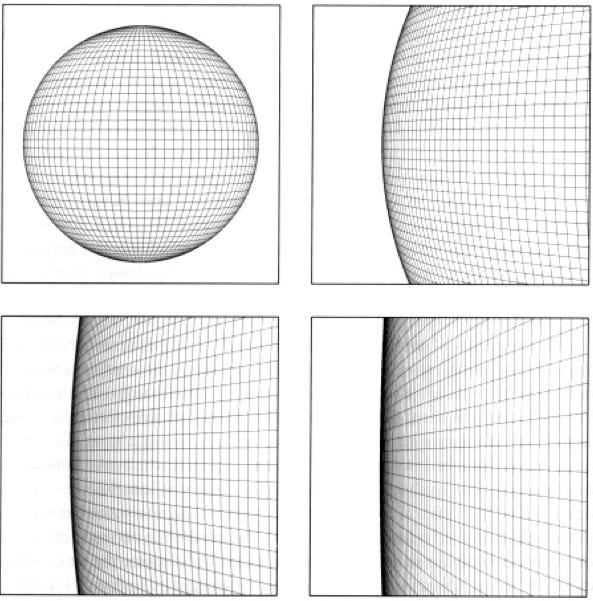
Just because the part of it we can see is indistinguishable from flat doesn’t mean it’s intrinsically flat in its entirety. But it does mean that the Universe is far larger than we’ll ever see. Even taking this minimum allowable estimate for the size of the Universe means that, at most, less than 0.0001% of the volume of the Universe is presently or will ever be observable to us. Once you put our knowledge about dark matter and dark energy in there, and consider how the Universe will expand into the future, you’ll realize that we’ll never see more of the Universe than we can right now.

So all that we see — from the billions of stars in our galaxy to the hundreds of billions of galaxies lighting up the observable Universe — is just a teeny-tiny fraction of what’s actually out there, beyond what the speed of light allows us to see.

And yet, we can know that it’s there. Isn’t science wonderful?

An earlier version of this post originally appeared on the old Starts With A Bang blog at Scienceblogs.