No, We Still Can’t Use Quantum Entanglement To Communicate Faster Than Light
It’s a pipe dream that violates the laws of physics, and not even quantum mechanics can give us a way out.
One of the most fundamental rules of physics, undisputed since Einstein first laid it out in 1905, is that no information-carrying signal of any type can travel through the Universe faster than the speed of light. Particles, either massive or massless, are required for transmitting information from one location to another, and those particles are mandated to travel either below (for massive) or at (for massless) the speed of light, as governed by the rules of relativity.
Since the development of quantum mechanics, however, many have sought to leverage the power of quantum entanglement to subvert this rule, devising clever schemes to attempt to transmit information to “cheat” relativity and communicate faster-than-light after all. Although it’s an admirable attempt to work around the rules of our Universe, faster-than-light communication is still an impossibility. Here’s the science of why.

Conceptually, quantum entanglement is a simple idea. You can start by imagining the classical Universe and one of the simplest “random” experiments you could perform: conducting a coin flip. If you and I each have a fair coin and flip it, we’d each expect that there’s a 50/50 chance of each of us getting heads and a 50/50 chance that each of us would get tails. Your results and my results should not only be random, they should be independent and uncorrelated: whether I get heads or tails should still have 50/50 odds irrespective of what you get with your flip.
But if this isn’t a classical system after all, and a quantum one instead, it’s possible that your coin and my coin will be entangled. We might each still have a 50/50 chance of getting heads or tails, but if you flip your coin and measure heads, you’ll instantly be able to statistically predict to better than 50/50 accuracy whether my coin was likely to land on either heads or tails.
How is this possible? In quantum physics, there exists a phenomenon known as quantum entanglement, which is where you create more than one quantum particle — each with their own individual quantum state — where you know something important about the sum of both states together. It’s as though there’s an invisible thread connecting your coin and my coin, and when one of us makes a measurement about the coin we have, we instantly know something about the state of the other coin that goes beyond the familiar classical randomness.
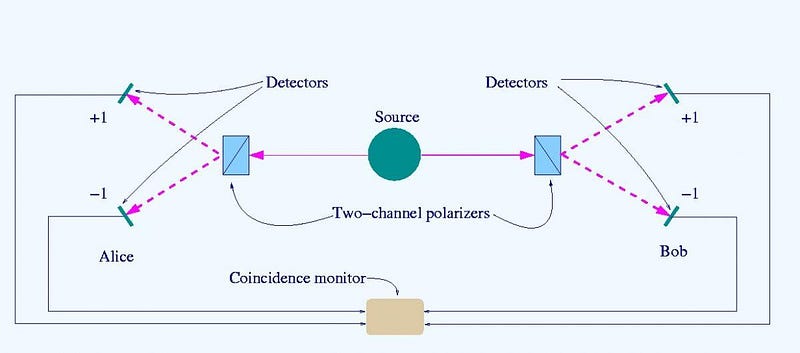
This isn’t mere theoretical work, either. We’ve created pairs of entangled quanta (photons, to be specific) that are then carried away from one another until they’re separated by large distances, then we have two independent measurement apparatuses that tell us what the quantum state of each particle is. We make those measurements as close to simultaneously as possible, and then get together to compare our results.
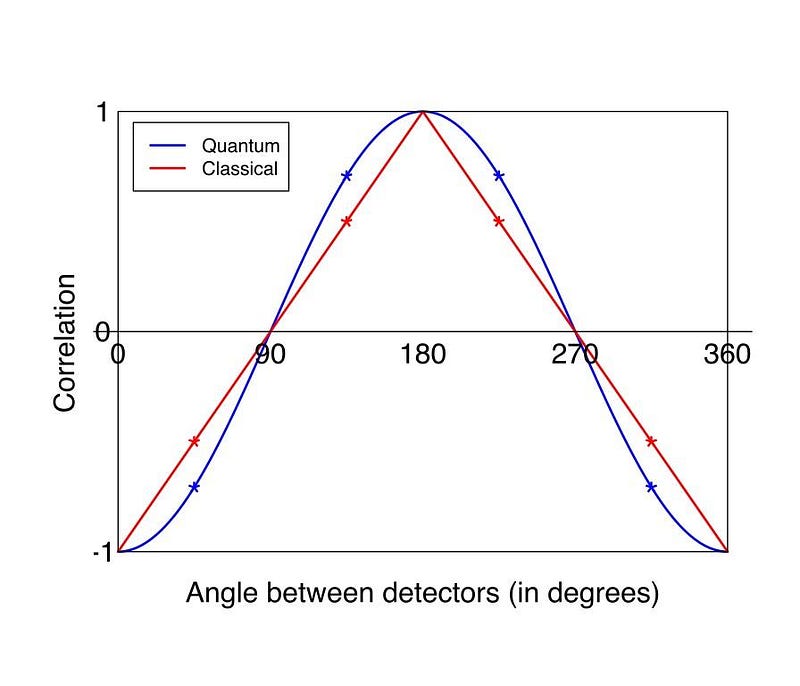
What we find, perhaps surprisingly, is that your results and my results are correlated! We’ve separated two photons by distances of hundreds of kilometers before making those measurements, and then measuring their quantum states within nanoseconds of one another. If one of those photons has spin +1, the other one’s state can be predicted to about a 75% accuracy, rather than the standard 50%.
Moreover, we can “know” that information instantaneously, rather than waiting for the other measurement apparatus to send us the results of that signal, which would take about a millisecond. It seems, on the surface, that we can know some information about what’s going on at the other end of the entangled experiment not only faster than light, but tens of thousands of times faster than the speed of light could ever transmit information.
Does that mean, though, that we can use quantum entanglement to communicate information at faster-than-light speeds?
It might seem so. For example, you might attempt to concoct an experiment as follows:
- You prepare a large number of entangled quantum particles at one (source) location.
- You transport one set of the entangled pairs a long distance away (to the destination) while keeping the other set at the source.
- You have an observer at the destination look for some sort of signal, and force their entangled particles into either the +1 state (for a positive signal) or a -1 state (for a negative signal).
- Then, you make your measurements of the entangled pairs at the source, and determine with better than 50/50 likelihood what state was chosen by the observer at the destination.
This seems like a great setup for enabling faster-than-light communication. All you need is a sufficiently prepared system of entangled quantum particles, an agreed-upon system for what the various signals will mean when you make your measurements, and a pre-determined time at which you’ll make those critical measurements. From even light-years away, you can instantly learn about what was measured at a destination by observing the particles you’ve had with you all along.
Right?
It’s an extremely clever scheme, but one that won’t pay off at all. When you, at the original source, go to make these critical measurements, you’ll discover something extremely disappointing: your results simply show 50/50 odds of being in the +1 or -1 state. It’s as though there’s never been any entanglement at all.

Where did our plan fall apart? It was at the step where we had the observer at the destination make an observation and try to encode that information into their quantum state.
When you take that step — forcing one member of an entangled pair of particles into a particular quantum state — you break the entanglement between the two particles. That is to say, the other member of the entangled pair is completely unaffected by this “forcing” action, and its quantum state remains random, as a superposition of +1 and -1 quantum states. But what you’ve done is completely break the correlation between the measurement results. The state you’ve “forced” the destination particle into is now 100% unrelated to the quantum state of the source particle.
The only way that this problem could be circumvented is if there were some way of making a quantum measurement to force a particular outcome. (Note: this is not something permitted by the laws of physics.)
If you could do this, then someone at the destination could conduct observations — for example, learning whether a planet they were visiting were inhabited or not — and then use some unknown process to:
- measure their quantum particle’s state,
- where the outcome will turn out to be +1 if the planet is inhabited,
- or -1 if the planet is uninhabited,
- and thereby enable the source observer with the entangled pairs to instantaneously figure out whether this distant planet is inhabited or not.
Unfortunately, the results of a quantum measurement are unavoidably random; you cannot encode a preferred outcome into a quantum measurement.
As quantum physicist Chad Orzel has written, there is a big difference between making a measurement (where the entanglement between pairs is maintained) and forcing a particular result — which itself is a change of state — followed by a measurement (where the entanglement is not maintained). If you want to control, rather than simply measure, the state of a quantum particle, you’ll lose your knowledge of the full state of the combined system as soon as you make that change-of-state operation happen.
Quantum entanglement can only be used to gain information about one component of a quantum system by measuring the other component so long as the entanglement remains intact. What you cannot do is create information at one end of an entangled system and somehow send it over to the other end. If you could somehow make identical copies of your quantum state, faster-than-light communication would be possible after all, but this, too, is forbidden by the laws of physics.
There’s an awful lot that you can do by leveraging the bizarre physics of quantum entanglement, such as by creating a quantum lock-and-key system that’s virtually unbreakable with purely classical computations. But the fact that you cannot copy or clone a quantum state — as the act of merely reading the state fundamentally changes it — is the nail-in-the-coffin of any workable scheme to achieve faster-than-light communication with quantum entanglement.
There are a lot of subtleties associated with how quantum entanglement actually works in practice, but the key takeaway is this: there is no measurement procedure you can undertake to force a particular outcome while maintaining the entanglement between particles. The result of any quantum measurement is unavoidably random, negating this possibility. As it turns out, God really does play dice with the Universe, and that’s a good thing. No information can be sent faster-than-light, allowing causality to still be maintained for our Universe.
Ethan Siegel is the author of Beyond the Galaxy and Treknology. You can pre-order his third book, currently in development: the Encyclopaedia Cosmologica.