Throwback Thursday: The Cosmic Speed Limit
The speed of light in a vacuum is the limit for massless particles, but massive ones are limited even further!
“All our sweetest hours fly the fastest.” -Virgil
If you’ve been around the block once or twice, you know that the speed of light in a vacuum — 299,792,458 meters-per-second — is the absolute maximum speed that any form of energy in the Universe can travel at. Gravitational waves move at this speed, light in the absence of other matter moves at this speed, even the gluon (in theory) moves at this speed! In shorthand, this cosmic speed limit is known as c to physicists.

But you or I, no matter how hard we try, will never attain that speed. There’s a simple reason for this: we have mass. And for an object with mass, you can accelerate it all you want, but it would take an infinite amount of energy to reach c, and I’m sorry, folks, there’s only a finite amount of energy in the Universe.
But that doesn’t mean we’ll settle for 90% of c, or 99%, or even 99.9999%. We always strive for that extra fraction of speed, that extra bit of energy, that extra push ever-closer to the unattainable limit. You learn about the frontiers of nature and push back the frontiers of knowledge with every little bit; with every extra fraction of a meter-per-second, with every fraction of a Kelvin closer to absolute zero, and with every extra attometer you probe this Universe.
You may be most familiar with our latest attempts to approach c at CERN, where we’ve recently discovered the Higgs Boson.

By smashing two protons into one another, one moving at 299,792,447 meters per second (just 11 m/s shy of the speed of light) in one direction and the other moving at the same speed in the opposite direction, we can produce incredibly energetic particles, bounded only by the energy available via Einstein’s E=mc^2. After the LHC’s upgrade is complete, that speed will increase to 299,792,455 m/s, which will make these by far the fastest protons ever created on Earth.
But they’re hardly the fastest particles we’ve ever made.

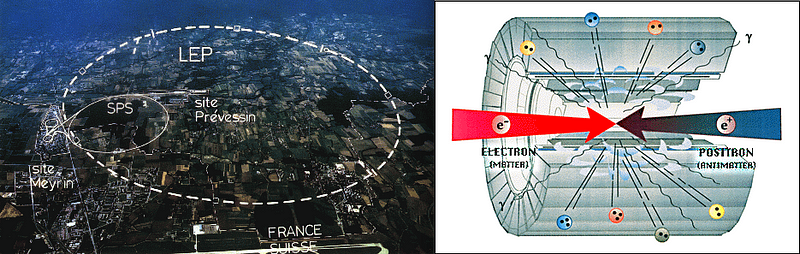
After all, a proton is a relatively heavy particle, some 1,836 times heavier than it’s orbiting friend, the electron! Even though we’ve created protons that are at higher energies than electrons, it only takes one-1,836th the energy (or 0.054%) to get the electron up to the same speed. (Those of you objecting that “this is not the formula for Kinetic Energy” should remember that these are ultra-relativistic speeds we’re talking about!) Which means that LEP — the Large Electron-Positron Collider (and the LHC’s predecessor) — where they got electrons up to 104.5 GeV of energy (compared to the 6,500 GeV expected for the LHC after the upgrade), still holds the record for particle accelerator record speed.
What is that speed? 299,792,457.9964 meters per second, or a whopping 99.9999999988% the speed of light, just 3.6 millimeters-per-second slower than light in a vacuum!
But that’s just here on Earth, with our meagre superconducting electromagnet accelerators, powered by puny chemical energy sources. Compared to what comes out of the Universe, our terrestrial sources don’t stand a chance.

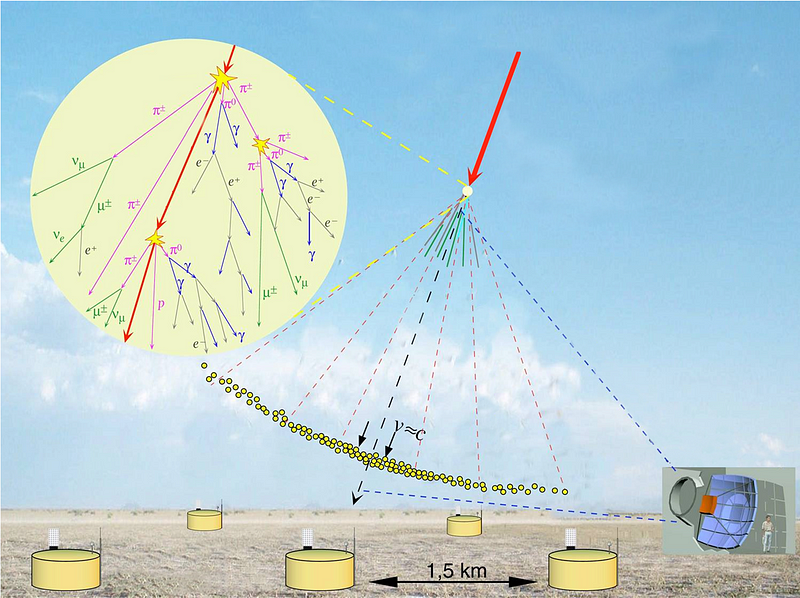
Outer space is filled with collapsed stars, supernovae and supermassive black holes — including ones at the center of active galaxies, above — where magnetic fields billions of times the likes of anything ever to appear on Earth are routine. From all directions in space, cosmic rays — high energy particles, mostly protons — fly through the Universe at energies dwarfing anything we’ve ever created or even experienced here on Earth.
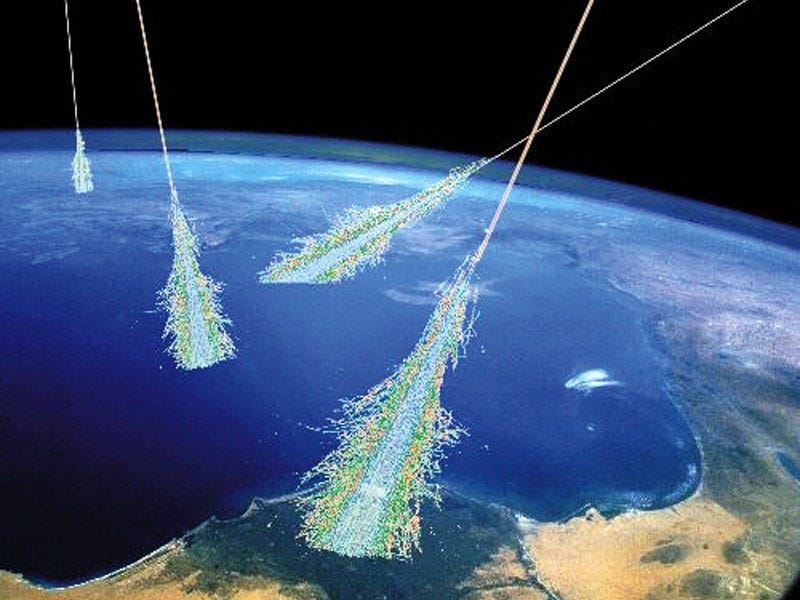
Yes, it’s true that there are fewer particles as we go to higher and higher energies, but the highest energies are no longer measured in terms of GeVs (Giga-electronVolts, or 10^9 eV), TeVs (Tera-electronVolts, or 10^12 eVs) or even PeVs (Peta-electronVolts, or 10^15 eVs). Instead, these energies can spike all the way up into-and-above the 10^19 eV-range!
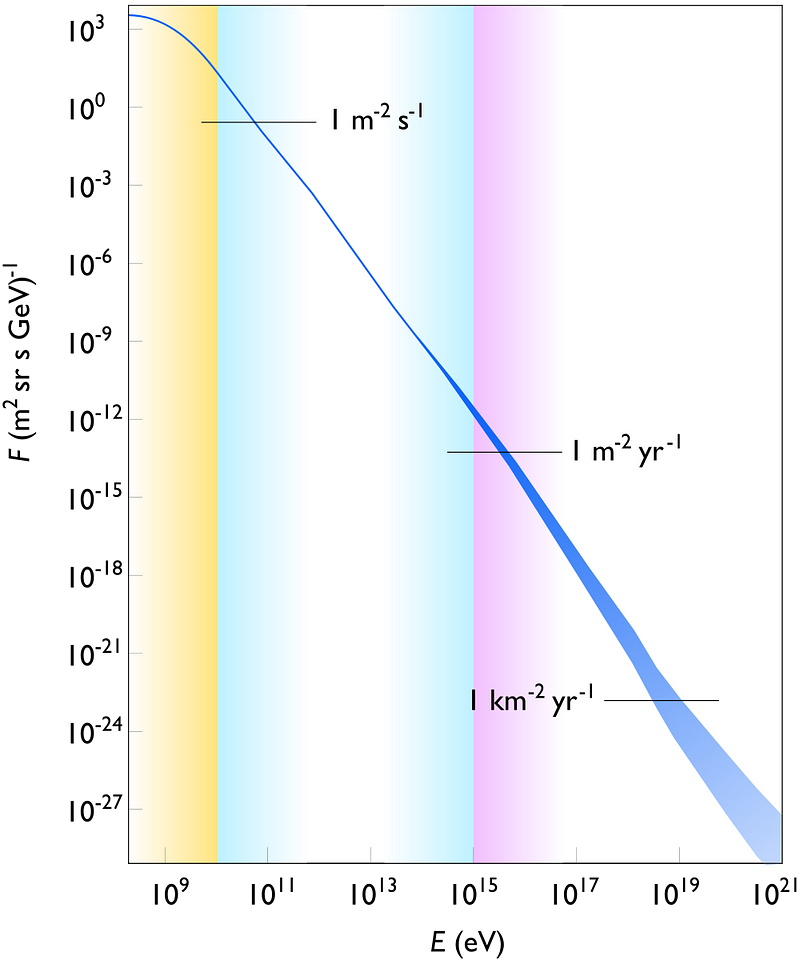
Now, this number is really, really interesting, and potentially even limiting! “How’s that,” you ask? Because above about 4-or-5 × 10^19 eV, the Universe won’t let you stay at that energy! The problem, believe it or not, is that no matter how high the energy is of the particle you initially made, it has to pass through the radiation bath that’s left over from the Big Bang in order to reach you.
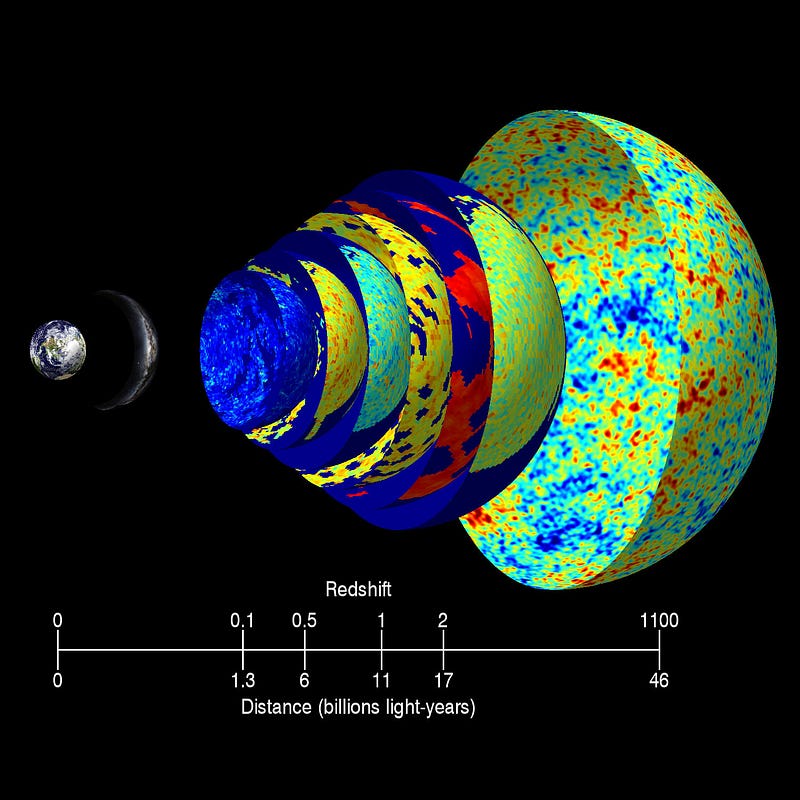
This radiation is incredibly cold, at an average temperature of some 2.725 Kelvin, or less than three degrees above absolute zero. If we sought to calculate the root-mean-squared energy of each photon in there, it’s on the order of just 0.00023 electron-Volts, a tiny number. Every time a high-energy charged particle has a chance to interact with a photon, it has the same possibility that all interacting particles have: if it’s energetically allowed, by E=mc^2, then there’s a chance it can make a new particle!

And that particle doesn’t get that energy for free; it has to come from the system that created it! Although you can make electron-positron pairs from a collision like this starting at energies around 10^17 eV, that’s a very inefficient process; particles can travel for many hundreds of millions of light-years above that energy.
But the lightest strongly interacting particle you can create from a collision like this is a neutral pion, which you need 135 MeV of energy to make. There’s a threshold for this that’s relatively easy to compute (previously done here), and what it tells you is that as long as you’re above a certain energy threshold — known as the GZK cutoff, named for Greisen-Zatsepin-and-Kuzmin — you’re going to emit those pions until you’re below that energy threshold! (And if you’re even higher energy, and can produce other particles, you’re going to lose energy even faster!)
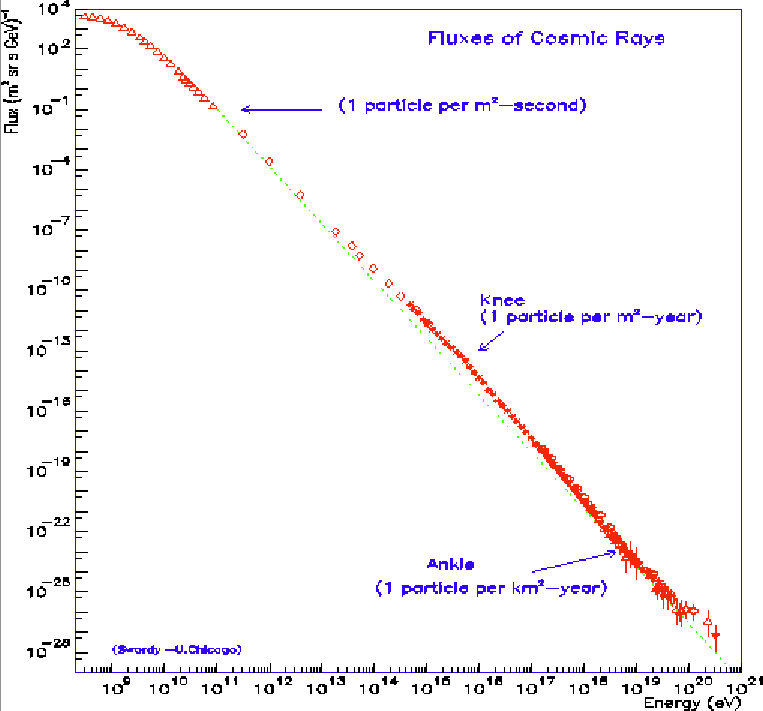
For a long time — until the last few years — many contended that we’d observed particles that exceeded this threshold, which meant that either they were being generated within our galaxy (somehow), which is the only location that would allow them to survive the trip to Earth, there was something wrong with our understanding of relativity (fat chance). But there was another, much more mundane option that most people thought likely: there was a problem measuring these unprecedented high energies.
Lo and behold, now the two most modern observatories/experiments looking for these — Pierre Auger Observatory and the High-Resolution Fly’s Eye Experiment — both see the GZK cutoff clearly, and no cosmic rays above about 5 × 10^19 eV. As far as a proton traveling with that energy goes, do you know what that means for speed? It tells us that a proton traveling at the GZK limit has a speed of:
299,792,457.999999999999918 meters-per-second.

Or, to put that in perspective, if you raced a proton of this energy and a photon to the nearest star-and-back (the red one in the middle, above), the photon would arrive first… with the proton just 22 microns behind, arriving 700 femtoseconds later.
And if you raced this proton and a photon all the way to the Andromeda galaxy and back — the largest galaxy gravitationally bound to us at a distance of about 2,540,000 light-years — the journey would take nearly 5 million years, and the proton would lose… by about 13 seconds.
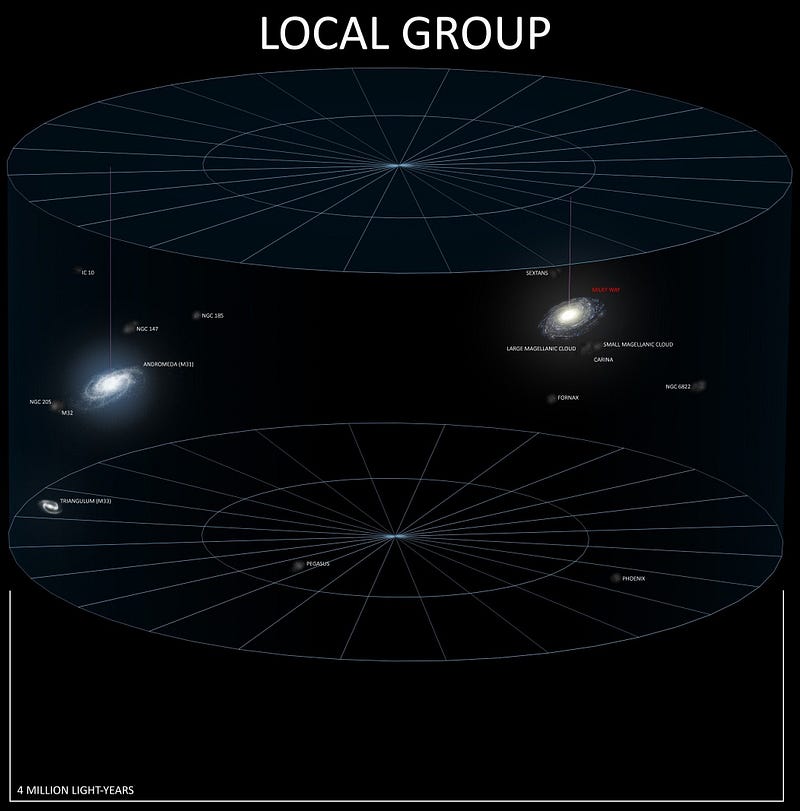
And every charged particle in the cosmos — every cosmic ray, every proton, every atomic nucleus — is limited by this speed! Not just the speed of light, but a little bit lower, thanks to the leftover glow from the Big Bang! So when you dream of traveling through the Universe, remember not to dream of moving arbitrarily close to the speed of light; the radiation from the Big Bang — at such low, microwave energies — will fry you if you do!
And that’s the cosmic speed limit for you, me, and everything else made of matter.
A version of this post originally appeared at the old Starts With A Bang blog on Scienceblogs. Have a question or comment? Head there now to our forum!