Throwback Thursday: The Fundamental Constants Behind Our Universe

How many does it take to give us our Universe, and what’s left unexplained?

“The joy of life consists in the exercise of one’s energies, continual growth, constant change, the enjoyment of every new experience. To stop means simply to die. The eternal mistake of mankind is to set up an attainable ideal.” –Aleister Crowley

But the Universe itself experiences continual growth, constant change, and new experiences all the time, and it does so spontaneously.

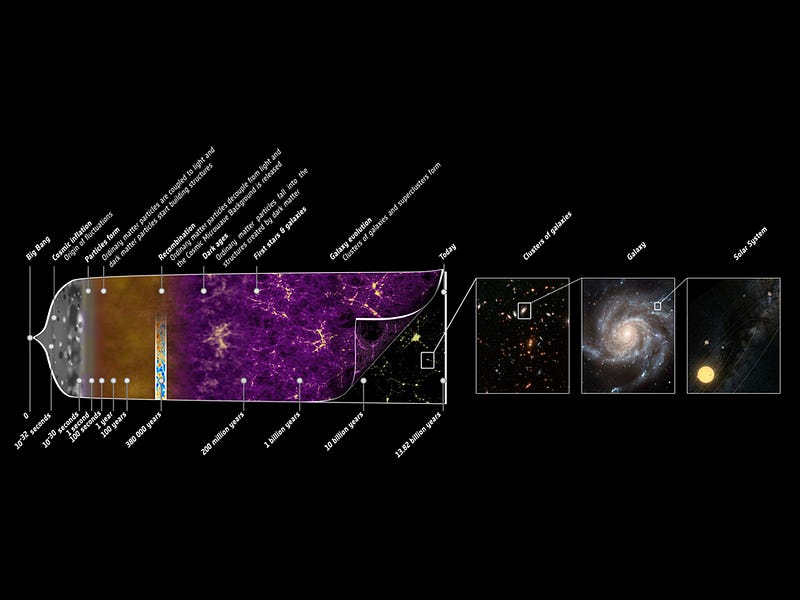
And yet, the better we understand our Universe — what the laws are that govern it, what particles inhabit it, and what it looked/behaved like farther and farther back in the distant past — the more inevitable it appears that it would look just as it appears today.

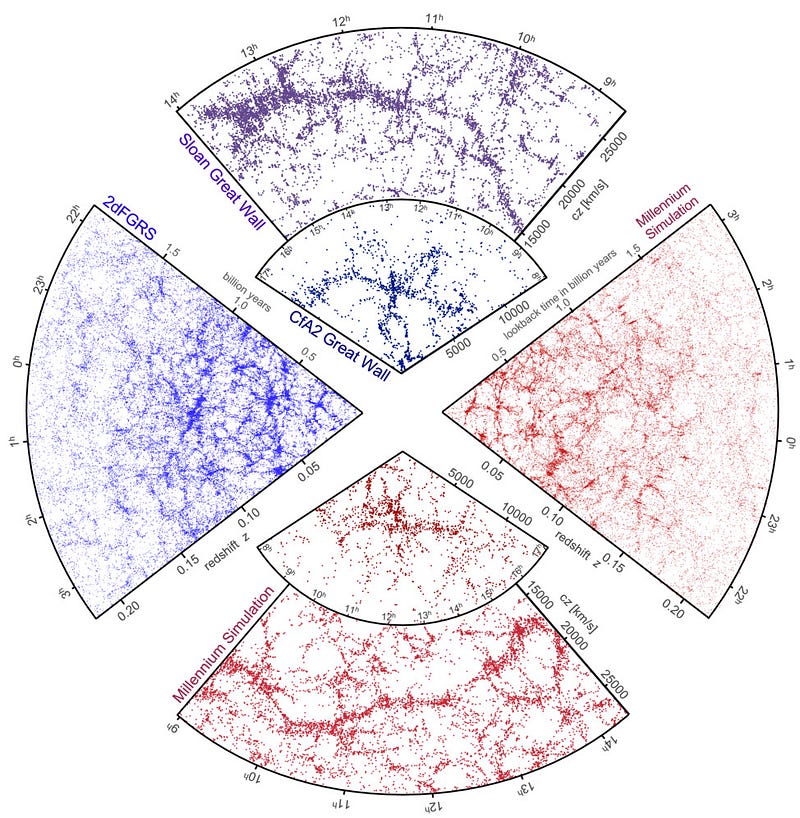
On the largest scales in our observable Universe, matter clumps and clusters together in a filamentary, web-like structure, while the densest parts form galaxies, stars and planets in isolation, in groups, and in clusters as appropriate.

Although different regions of space and different simulation runs will have slightly different details, the pattern of clustering is always the same; if we were to go as far back to the beginning as our physical understanding allows, we would get a Universe indistinguishable from ours in all but the most minute details 100 times out of 100.

By time the Universe is as old as ours is — 13.8 billion years — it will look exactly the same every time in so many important ways:

• It will have the same number of galaxies, of the same mass, clustered together in the same ways,
• The ratios of the elements in the Universe will be identical, overall, to the elemental abundance today,
• It will have the same number of stars and planets with the same mass distribution as our Universe,
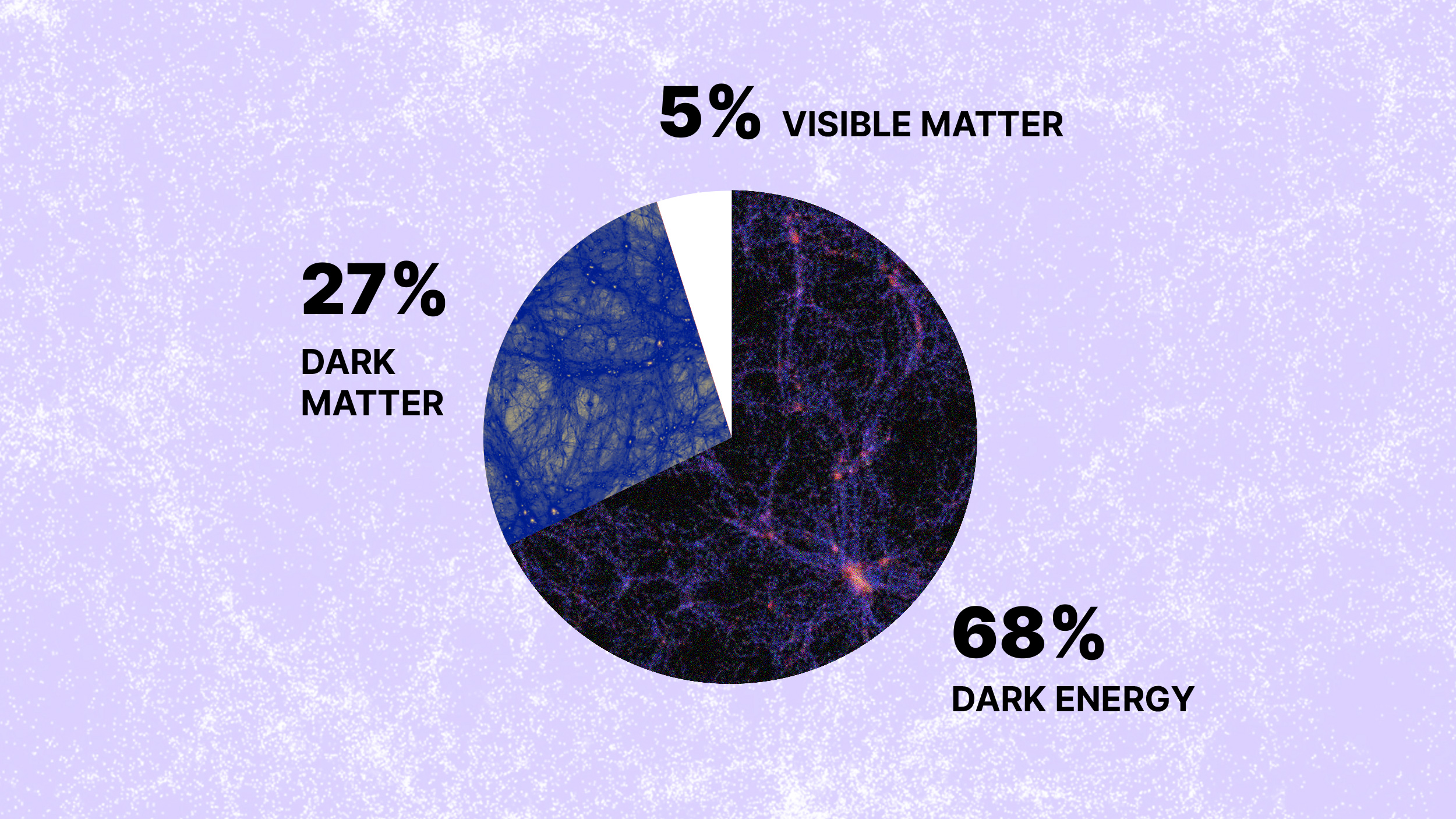
• It will have the same ratio of dark energy, dark matter, normal matter, neutrinos, and radiation as our Universe,
• and, perhaps most importantly, all of the fundamental constants will have the same value.

This last one is so important, because starting with the same rough initial conditions is what guarantees our Universe will look the way it does. But what are these constants?

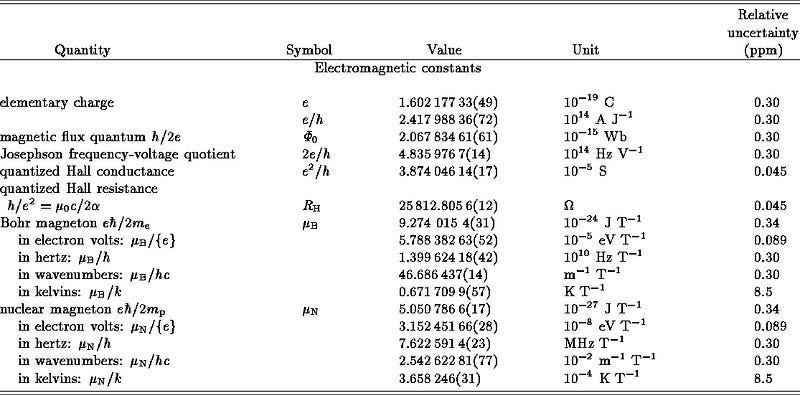
You might be used to constants like c, the speed of light, h (or ħ), Planck’s constant, and G, Newton’s gravitational constant. But these constants are dimension-ful, meaning that they depend on the units (e.g., meters, seconds, kilograms, etc.) you use to measure them.

But the Universe, very clearly, doesn’t care what units-of-measurement you use! So we can create dimensionless constants, or combinations of these physical constants that are simply just numbers, numbers that describe how different parts of the Universe relate to one another.

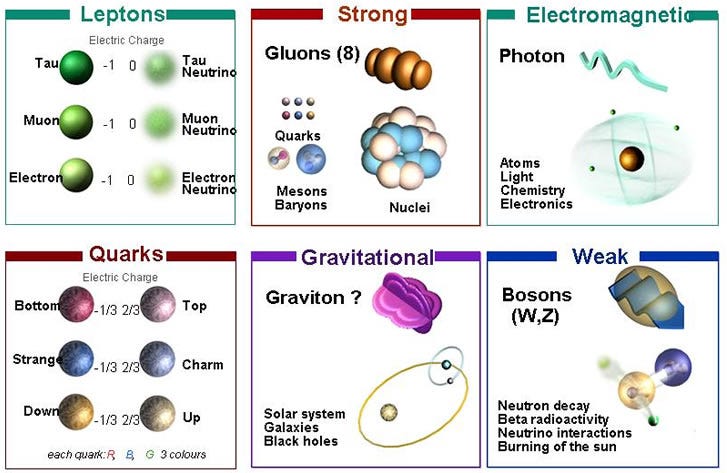
We’d like to describe our Universe as simply as possible; one of the goals of science is to describe nature in the simplest terms possible, but no simpler. How many of these does it take, as far as we understand our Universe today, to completely describe the particles, interactions, and laws of our Universe?

Quite a few, surprisingly: 26, at the very least. Let’s take a look at what these are.

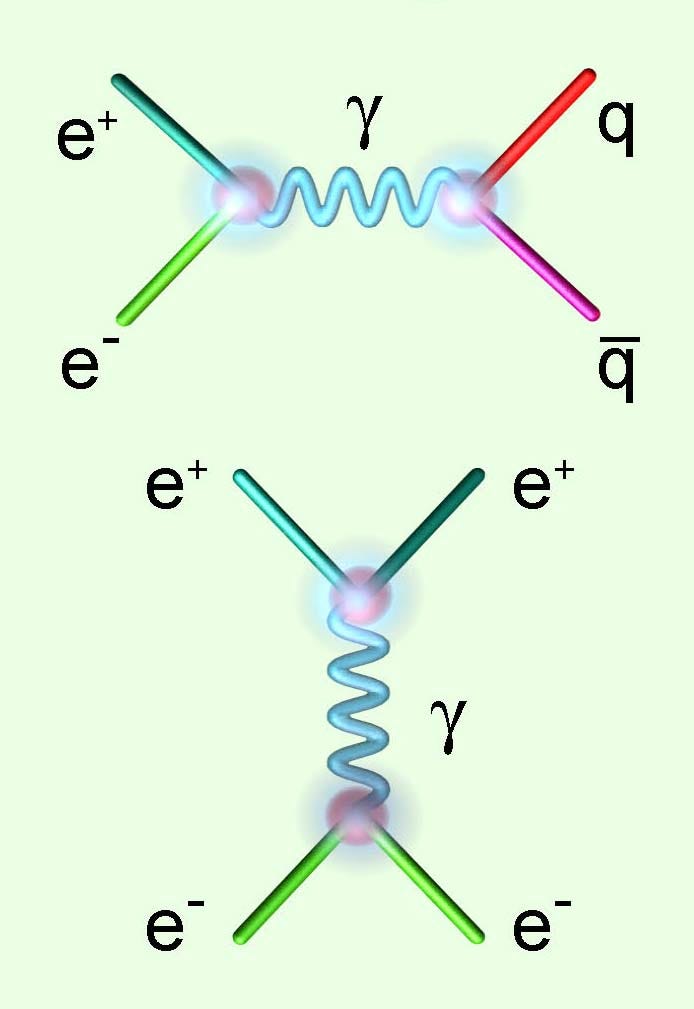
1.) The fine-structure constant, or the strength of the electromagnetic interaction. In terms of some of the physical constants we’re more familiar with, this is a ratio of the elementary charge (of, say, an electron) squared to Planck’s constant times the speed-of-light. At the energies of our Universe, this number comes out to ≈ 1/137.036, although the strength of this interaction increases as the energy of the interacting particle rises. This is thought to be due to a relative increase in how elementary charges behave at higher energies, although this is not yet a certainty.

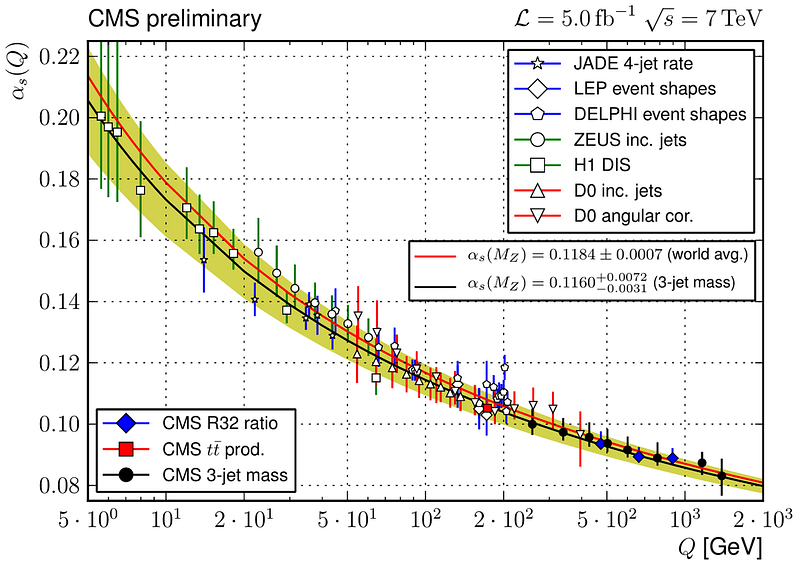
2.) The strong coupling constant, or the strength of the strong nuclear force. Although the way the strong force works is very different and counterintuitive compared with either the electromagnetic force or gravity, the strength of this interaction can be parametrized by a single coupling constant. This constant of our Universe, too, like the electromagnetic one, changes strength with energy.

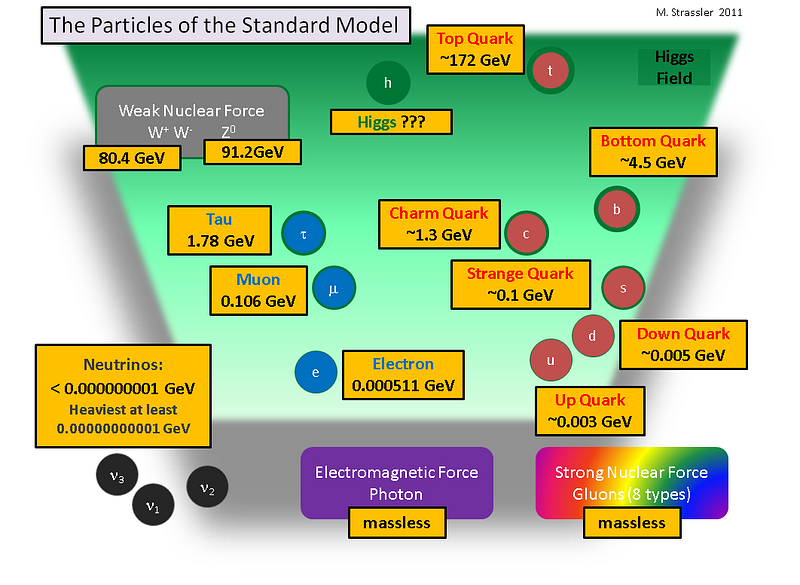
3–17.) The (non-zero) masses of the fifteen fundamental standard model particles with a rest-mass, relative to a fundamental scale set by Einstein’s gravitational constant. (This way, no separate constant is needed for gravitation.) In the standard model, this typically manifests itself via fifteen coupling constants (to the Higgs field) for the electron, muon and tau, the three neutrino species, the six quarks, the W and Z bosons, and the Higgs boson. (If you preferred a different parametrization, you could replace the W-and-Z masses with the weak coupling constant and the Higgs field’s expectation value; your choice.) The photon and the eight gluons don’t get one, being intrinsically massless particles.

This is, I’ll note, a source of much distress for theorists, who hoped that these constants — the fundamental masses of the elementary particles — would either be part of some pattern (they’re not), calculable from first principles (they’re not), or would emerge dynamically from some larger framework, like a GUT or string theory (they don’t).

18–21.) The quark mixing parameters. These four parameters dictate how all of the weak nuclear decays happen, and allow us to calculate the probability amplitudes of different radioactive decay products. Because the up, charm and top quarks (as well as the bottom, strange and down quarks on the other hand) all have the same quantum numbers as one another, they can mix together. The details of the mixing is normally parametrized by the Cabibbo-Kobayashi-Maskawa (CKM) Matrix, which gives three quark mixing angles, as well as one CP-violating complex phase.

These four parameters, again, cannot be predicted from any other principle, and must simply be measured at this point in time.

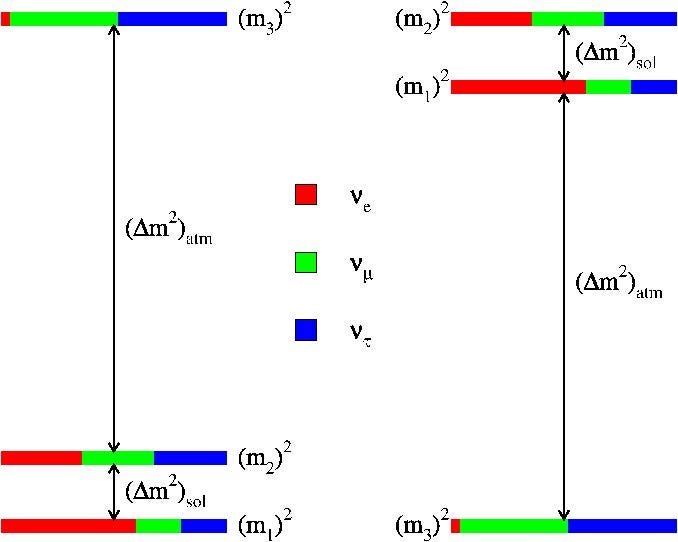
22–25.) The neutrino mixing parameters. Similar to the quark sector, there are four parameters that detail how neutrinos mix with one another, given that the three types of neutrino species all have the same quantum number. As of today, the three angles have been measured with some reasonable precision, although the CP-violating phase has not been. The mixing is parametrized by (what I know as) the Maki-Nakagawa-Sakata (MNS) Matrix, although it’s worth pointing out that the mixing angles are all huge compared to what they are for the quarks, so much so that the electron, muon and tau neutrinos are each superpositions of the three “fundamental” neutrino species that mix together significantly. This is because the mass differences between the different quark species is tremendous, ranging from maybe 6-to-300,000 times the mass of an electron, while the mass differences between neutrino species is at most 0.000016% an electron’s mass.

And finally…

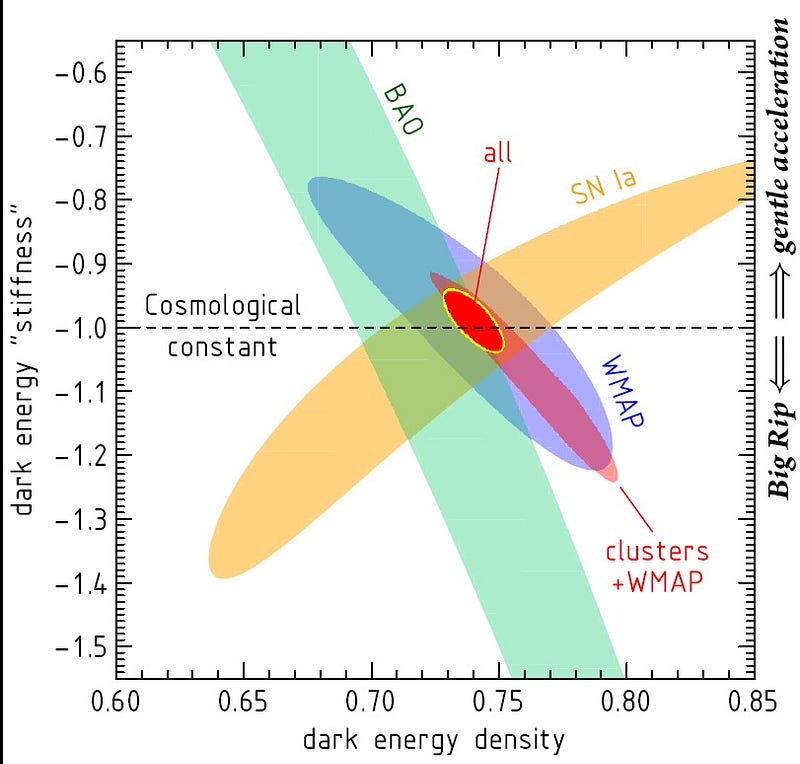
26.) The cosmological constant, or the dimensionless constant driving the accelerated expansion of the Universe. This is another constant whose value cannot be derived, and is simply a measured fact, at least at this point in time.

If you rewind the Universe to a time just maybe a few picoseconds after the Big Bang, and start it off with roughly the same initial conditions and these 26 fundamental constants, you’ll get roughly the same Universe each and every time. The only differences will be encoded in quantum mechanical probabilities and the extent that the initial conditions varied.

But even this can’t explain everything about the Universe! For example:

• The amount of CP-violation encoded by our constants, regardless of what the complex phase from the MNS-Matrix is, cannot explain the observed matter-antimatter asymmetry in our Universe. That requires some sort of new physics, which means there’s got to be a new fundamental parameter in there, too.
• If there is CP-violation in the strong interactions, that would be a new parameter as well, and if not, the physics (or symmetry) that prevents it may well carry a new constant (or multiple constants) along with it.
• Did cosmic inflation happen, and if so, what parameter(s) is/are associated with that?
• What is the dark matter? Given the (reasonable) assumption that it’s a massive particle, it almost certainly requires at least one (and probably more than one) new fundamental parameter to describe it.

And so that’s where we are today.

We don’t yet know where the values of these constants come from, or whether that’s something that will ever be known with the information available in our Universe. Some people chalk them up to anthropics or appeal to the multiverse; I haven’t given up on our Universe just yet, though!

Our journey through the cosmos continues, and there’s so much more still to learn.

Leave your comments at the Starts With A Bang forum on Scienceblogs!