3 big lessons from Einstein’s most famous equation: E=mc²

For as long as humans had been conducting science, there was a seemingly immutable law of nature that was never violated: that in any reaction occurring in the Universe, mass was conserved. If you took a boulder and cleaved it into two, the two pieces would have the same mass as the initial, unbroken stone. If you devoured a massive dinner, your weight after you finished eating was the same as your weight before your meal plus the meal itself. And even with the advent of chemistry, whether you dissolved salts in a liquid, precipitated crystals out of a solution, or combusted a reactive substance with oxygen in the air, if you kept track of everything that went into the reaction with everything that came out of it, you’d find that mass was conserved.

In other words, no matter what you put in, what reacted, and what comes out of any before-and-after scenario, the sum of what you began with and the sum of what you ended with must be equal. Mass, as far as we could tell, was truly a conserved quantity. But once we uncovered the laws of special relativity, we realized that mass, alone, simply couldn’t be the ultimate conserved quantity. After all, different observers that moved relative to one another would fundamentally disagree about what the total energy of a system was.

Instead of mass being strictly conserved, Einstein was able to derive a law that we still use today, governed by one of the simplest but most powerful equations ever to be written down, E = mc².

A nuclear-powered rocket engine, preparing for testing in 1967. This rocket is powered by eass/energy conversion, as dictated by Einstein’s most famous equation: E = mc². Few, even among those who knew Einstein best, could have foreseen the slew of remarkable developments he would introduce into physics in the early 1900s.

This one equation, E = mc², is arguably the most famous equation in all of physics, and may be the shortest, simplest, but most widely-known thing that Einstein ever put forth into the world. If we take a look at the individual parts of it, we realize that there are only four components to Einstein’s most famous statement:

1. E, or energy, which is the entirety of one side of the equation, and represents the total energy of the system in question.
2. =, or an equals sign, signifying that what’s on the left-hand side and the right-hand side of the equation are equivalent.
3. m, or mass, which is specifically the rest mass, or the mass you would measure for this object if it were not in motion in any way, which is related to the object’s energy by a conversion factor.
4. And c², which is the speed of light squared: the only proper conversion factor that we can use to balance the equation.

What it tells you, immediately, is that every body that has a rest mass to it, m, also has an equivalent amount of energy inherent to it, E, which isn’t necessarily surprising.

Niels Bohr and Albert Einstein, discussing a great many topics in the home of Paul Ehrenfest in 1925. The Bohr-Einstein debates were one of the most influential occurrences during the development of quantum mechanics. Today, Bohr is best known for his quantum contributions, but Einstein is better-known for his contributions to relativity and mass-energy equivalence. Both were known for thinking long and hard about the most difficult puzzles the Universe had to offer.

However, never before had that inherent energy been quantified correctly, nor had it been shown that energy and mass aren’t just related, but that they’re equivalent to one another: having mass, all by itself, means that you have something that contains energy as well. That’s part of the reason why the implications of Einstein’s most famous equation are so thoroughly world-changing. As Einstein himself put it:

“It followed from the special theory of relativity that mass and energy are both but different manifestations of the same thing — a somewhat unfamiliar conception for the average mind.”

If you have enough energy, his equation seemed to imply that you could generate mass. If you have mass, any mass at all, it should be possible to somehow extract that energy from it and use it for other purposes. And if you look at that conversion factor, c², you instantly recognize how enormous that is. The speed of light, c, is a fantastic 299,792,458 m/s, or about ten thousand times greater than Earth’s revolutionary orbit around the Sun. Square it, and that number becomes even larger, which implies that even small amounts of mass are storing fantastically large amounts of energy within them.

There are many different meanings that can be extracted from this simple equation, but here are the three most profound ways to interpret E = mc².

The quarks, antiquarks, and gluons of the standard model have a color charge, in addition to all the other properties like mass and electric charge. All of these particles, except gluons and photons, experience the weak interaction. Only the gluons and photons are massless; everyone else, even the neutrinos, have a non-zero rest mass.

1.) Even masses at rest have an inherent amount of energy to them. We’ve all learned about the various different types of energies, including:

• mechanical energy, such as the energy required to lift a heavy weight up to a certain height,
• chemical energy, such as the energy stored in the interior of an acid battery,
• electrical energy, such as the energy that flows through a light bulb and causes it to illuminate,
• as well as kinetic energy, such as the energy that a car barreling down the highway possesses.

These are all energies inherent to moving or reacting objects, and these forms of energy can be used to perform what physicists call work. This allows us to perform tasks such as run an engine, grind grain into flour, to heat or cool our homes, or even to power a large city.

That’s why it’s so shocking to discover that even plain, old, regular mass at rest not only has a positive, non-zero amount of energy inherent to it, but an amount of energy that is absolutely tremendous. This carries with it a momentous implication: that gravitation, which was previously thought only to occur between any two masses in the Universe in Newton’s picture, should also be at play when objects with only energy, which is equivalent to mass via E = mc², interact.

The production of matter/antimatter pairs (left) from two photons is a completely reversible reaction (right), with matter/antimatter annihilating back to two photons. This creation-and-annihilation process, which obeys E = mc², is the only known way to create and destroy matter or antimatter. If high-energy gamma-rays collide with normal photons from starlight, there is a chance to produce electron-positron pairs, diminishing the gamma-ray flux observed at great distances.

2.) Mass, under the right circumstances, can be converted into pure energy. This is the second meaning of the equation, E = mc². If the two sides of the equation are equal, then it’s possible to take something on one side of the equation, like mass, and convert it into the thing on the other side of the equation: energy. The relationship given by the equation itself tells us exactly how much energy you’ll liberate whenever you make that conversion: from mass into energy. Specifically, for every 1 kilogram of mass you turn into energy, you get 9 × 10¹⁶ joules of energy out, which is the energy equivalent of 21 Megatons of TNT, or one of the most powerful hydrogen bombs ever detonated on Earth.

This conversion is at play in many more reactions than just thermonuclear ones. When we experience a radioactive decay, a nuclear fission or fusion reaction, or even — if we make our measurements precisely enough — an energy-liberating chemical reaction, the mass of what we start with (the reactants) is greater than the mass we end up with (the products), rendering the old law of “conservation of mass” invalid.

In fact, the amount of the difference in mass between products and reactants equates to precisely how much energy is released during such a reaction! That’s true for everything from decaying uranium to fission bombs to nuclear fusion in the Sun to matter-antimatter annihilation. The amount of mass you destroy becomes energy, and the amount of energy you get is given by E = mc².

The particle tracks emanating from a high energy collision at the LHC in 2012 show the creation of many new particles. By building a sophisticated detector around the collision point of relativistic particles, the properties of what occurred and was created at the collision point can be reconstructed, but what’s created is limited by the available energy from Einstein’s E = mc². The maximum LHC energies are nearly a factor of a trillion (10^12) lower than the energies present at the start of the hot Big Bang.

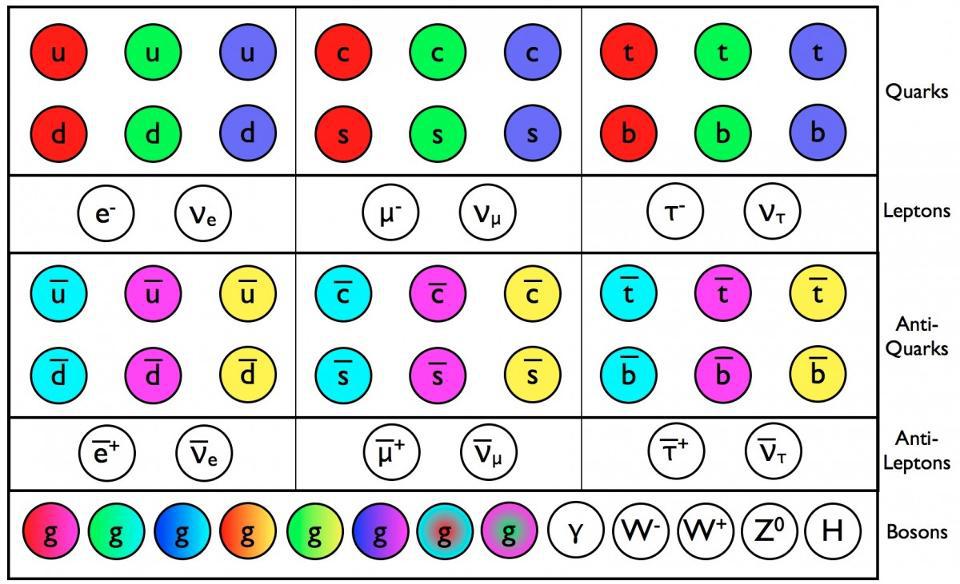
3.) Energy can be used to make mass out of literally nothing other than pure energy itself. The final meaning of E = mc² is arguably the most profound. If you take two billiard balls and smash them together, you get two billiard balls out. If you take a photon and an electron and smash them together, you get a photon and an electron out. If you smash two protons together, you’ll get two protons out. But if you smash two protons together with enough energy, you’ll have a chance to get two protons plus a new matter-antimatter pair of particles out. In other words, you will have created two new massive particles:

• a matter particle, such as an electron, proton, neutron, etc.,
• and an antimatter particle, such as a positron, antiproton, antineutron, etc.,

whose existence can only arise if you put in enough energy to begin with.

This is exactly the mechanism by which particle accelerators, like the LHC at CERN, search for new, unstable, high-energy particles (like the Higgs boson or the top quark) in the first place: by making new particles out of pure energy. The mass that you can create comes from the available energy: m = E/c². (It also means that if your particle has a finite lifetime, then due to Heisenberg’s uncertainty principle, there’s an inherent unknowability to its mass, since ∆E∆t ~ħ, and therefore there’s a corresponding ∆m that arises from Einstein’s E = mc², too.) When physicists talk about a particle’s width, this inherent mass uncertainty is a primary contributor to why all particles of the same species don’t have exactly the same measured mass.

The inherent width, or half the width of the peak in the above image when you’re halfway to the crest of the peak, is measured to be 2.5 GeV: an inherent uncertainty of about +/- 3% of the total mass. The mass of the particle in question, the Z boson, is peaked at 91.187 GeV, but that mass is inherently uncertain by a significant amount owing to its excessively short lifetime. This result is remarkably consistent with Standard Model predictions.

Interestingly enough, it was the very fact of this mass-energy equivalence that also led Einstein to what many consider his greatest scientific achievement: general relativity. One way to think about this is as follows. Imagine that you have, in your possession, two particles: a particle of matter and a particle of antimatter, each with the same rest mass. If these two particles (or, technically, this particle and antiparticle) meet, there’s a strong chance that they’ll annihilate, and when they do, they’ll produce two photons, each of a specific amount of energy: the energy given by Einstein’s mass-energy equivalence equation, E = mc².

Now, imagine that instead of these particles being initially at rest, you concocted a scenario where both members of this particle/antiparticle pair were moving rapidly, as though they had fallen from high up in outer space, and then annihilated close to the surface of Earth. What type of photons would we then produce?

Would those photons only have the energy, E, that you’d expect from Einstein’s E = mc²? No, that would violate the conservation of energy. Instead, those photons would now have extra energy: not just the E from E = mc², but the additional E from the amount of kinetic energy they gained by falling: via the conversion of gravitational potential energy into kinetic energy, or the energy of their motion.

If two objects of matter and antimatter at rest annihilate, they produce photons of an extremely specific energy. If they produce those photons after falling deeper into a gravitational field, the energy should be higher. This means there must be some sort of gravitational redshift/blueshift, the kind not predicted by Newton’s gravity, otherwise energy wouldn’t be conserved.

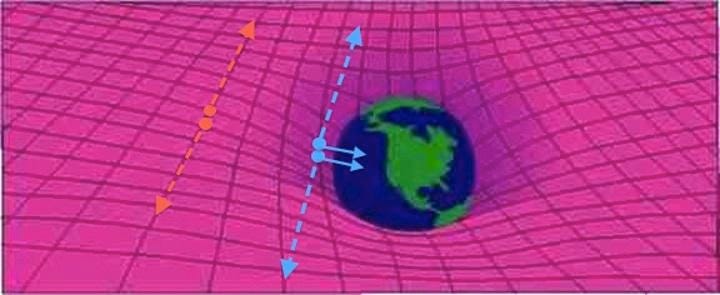
In order to obey the conservation of energy, we have to understand that a particle’s energy isn’t just the “rest mass” portion of the equation, which is E = mc², but that there’s also all the other types of energy present, including gravitational energy and kinetic energy. For photons, which are massless, the energy of a photon is directly related to its wavelength, and therefore, if a photon “climbs out” of a gravitational field, it will experience gravitational redshift, as its wavelength lengthens as it loses energy. Similarly, if a photon “falls deeper into” a gravitational field, it must gain energy, which means it experiences a gravitational blueshift, which shortens its wavelength.

In Newtonian gravity, there’s no way to account for this effect, but in Einstein’s general relativity, the curvature of space implies that falling into a gravitational field makes you gain energy, whereas climbing out of a gravitational field, conversely, makes you lose energy.

When a quantum of radiation leaves a gravitational field, its frequency must be redshifted to conserve energy; when it falls in, it must be blueshifted. Only if gravitation itself is linked to not only mass but energy, too, does this make sense. Gravitational redshift is one of the core predictions of Einstein’s general relativity, but has only recently been tested directly in such a strong-field environment as our galactic center.

The full and general relationship, then, for any moving object, isn’t just E = mc², which turns out to be only half of the full equation. Instead, the full equation relating energy to mass is E² = m²c⁴ + p²c², where p is momentum of the particle or object in question. (This even works for massless particles, like photons, which do carry energy through their momentum.) Only by generalizing our conception of reality to include energy, momentum, and gravity can we fully describe the Universe.

When the momentum of any particle (or set of particles) gets set to zero, which can happen for any particle (or antiparticle) with a non-zero rest mass, we then swiftly recover Einstein’s mass-energy-equivalence equation in its original form: E = mc². By starting with fewer particles (and/or antiparticles) with either a great amount of momentum or with a large amount of energy added to (or pumped into) them, we can literally create particle-antiparticle pairs if enough energy is available, where those new particles can have a rest mass (m) up to the amount of available energy (E/c²) needed to create them.

When a meson, such as a charm-anticharm particle shown here, has its two constituent particles pulled apart by too great an amount, it becomes energetically favorable to rip a new (light) quark/antiquark pair out of the vacuum and create two mesons where there was one before. A strong enough electric field, for long-enough lived mesons, can cause this to occur, with the needed energy for creating more massive particles coming from the underlying electric field, and with the amount of energy required to create these new particles (or particle-antiparticle pairs) described by E = mc².

But the start of all of that, of the knowledge of antimatter, of nuclear reactions, of the gravitational behavior of particles without mass, and even of general relativity can all trace back its origin to Einstein’s greatest and most well-known equation, E = mc². The equivalence of mass and energy is truly a triumph of the power and simplicity of fundamental physics. Matter, of all types, has an inherent amount of energy to it. Any particle with mass can be converted, under the right conditions, into energy that can be used for an enormous variety of both constructive and destructive purposes. And finally, energy alone is the only necessary ingredient for the creation of massive objects that did not exist previously.

It was by thinking about problems in this exact fashion that generations of subsequent physicists were able to:

• discover the fundamental particles that compose our Universe,
• to invent both nuclear power and nuclear weapons,
• and to discover the theory of gravity that describes how every object in the Universe interacts.

Remarkably, the key to figuring this equation out lies in a simple thought experiment that is rooted in putting two key assumptions together: that whenever particles interact, both energy and momentum must be conserved. The rest is just an inevitable consequence of the Universe’s laws and rules, which work exactly as Einstein speculated they ought to more than 100 years ago.